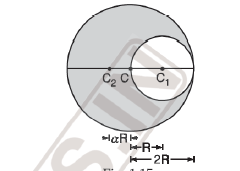
A circular disc of radius R is removed from a bigger circular dise of radius 2 R from one edge of the disc as shown in Fig. The centre of mass of the new disc is $α R$ from the centre of the bigger disc. Find the value of $α$ .
Solution in Punjabi

$\frac{1}{2}$

$\frac{1}{3}$

$\frac{1}{4}$

$\frac{1}{6}$

Video Solution

Answer

Step by step video & image solution for A circular disc of radius R is removed from a bigger circular dise of radius 2 R from one edge of the disc as shown in Fig. The centre of mass of the new disc is alpha Rfrom the centre of the bigger disc. Find the value of alpha. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS Moment of Inertia

A circular disc of radius R is removed from a bigger circular dise of radius 2 R from one edge of the disc as shown in Fig. The centre of mass of the new disc is α R from the centre of the bigger disc. Find the value of α . Solution in Punjabi

Topper's Solved these Questions

Similar Questions

MODERN PUBLICATION-Moment of Inertia-Example

A circular disc of radius R is removed from a bigger circular dise of radius 2 R from one edge of the disc as shown in Fig. The centre of mass of the new disc is $α R$ from the centre of the bigger disc. Find the value of $α$ .
Solution in Punjabi