Uniform thin, rod AB of length L and mass m is undergoing fixed axis rotation whose axis of rotation passes through A, such that end A remains stationary as shown. The kinetic energy of section AP of rod is equal to kinetic energy of section BP of rod at a given instant. Then the ratio of length AP and length AB, that is, APAB is equal to

12
121/3
1√2
12√2
The correct Answer is:B
A uniform thin .......
The KE of given section AP and PB will be equal if Ml of each section AP and section PB about A is same.

IAP=(λx)x23
Ipg=IAB−IAP=(2x)L23−(2x)x23
∴IAPIPB⇒λx33=λl33−λx33
orx3=L33orx=L21/3
Topper's Solved these Questions
Similar Questions
A uniform thin rod AB of length L and mass m is undergoing rotation about a fixed axis passing through end A and perpendicular to the rod, such that end A remains stationary as shown. The kinetic energy of section AP of the rod is equal to the kinetic energy of section BP of the rod at an instant. Then what is the value of (ABAP)3? (AB and AP are lengths of respectively parts of the rod)

View SolutionA uniform thin rod AB of length L and mass m is undergoing rotation about a fixed axis passing through end A and perpendicular to the rod, such that end A remains stationary as shown. The kinetic energy of section AP of the rod is equal to the kinetic energy of section BP of the rod at an instant. Then what is the value of (ABAp)3? (AB and AP are lengths of respectively parts of the rod)

View Solution
Knowledge Check
A uniform thin rod of length l is suspended from one of its ends and is rotated at f rotations per second. The rotational kinetic energy of the rod will be
A23π2f2ml2B43f2ml2C4π2f2ml2DzeroA uniform thin rod of length l is suspended from one of its ends and is rotated at f rotations per second. The rotational kinetic energy of the rod will be
A23π2f2ml2B43f2ml2C4π2f2ml2DZeroA uniform rod of mass m and length l makes a constant angle θ with an axis of rotation which passes through one end of the rod. Its moment of inertia about this axis is
Aml23Bml23sinθCml23sin2θDml23cos2θ
A uniform thin rod of length l is suspended from one of its ends and is rotated at f rotations per second. The rotational kinetic energy of the rod will be
View SolutionA uniform rod AB of length l and mass m is free to rotate about an Axis passing through A and perpendicular to length of the rod. The rod is released from rest as shown in figure. Given that the moment of inertia of the rod about A is ml23 The initial angular acceleration of the rod will be
View SolutionA thin uniform copper rod of length l and mass m rotates uniformly with an angular velocity ω in a horizontal plane about a vertical axis passing through one of its ends. Determine the tension in the rod as a function of the distance r from the rotation axis. Find the elongation of the rod.
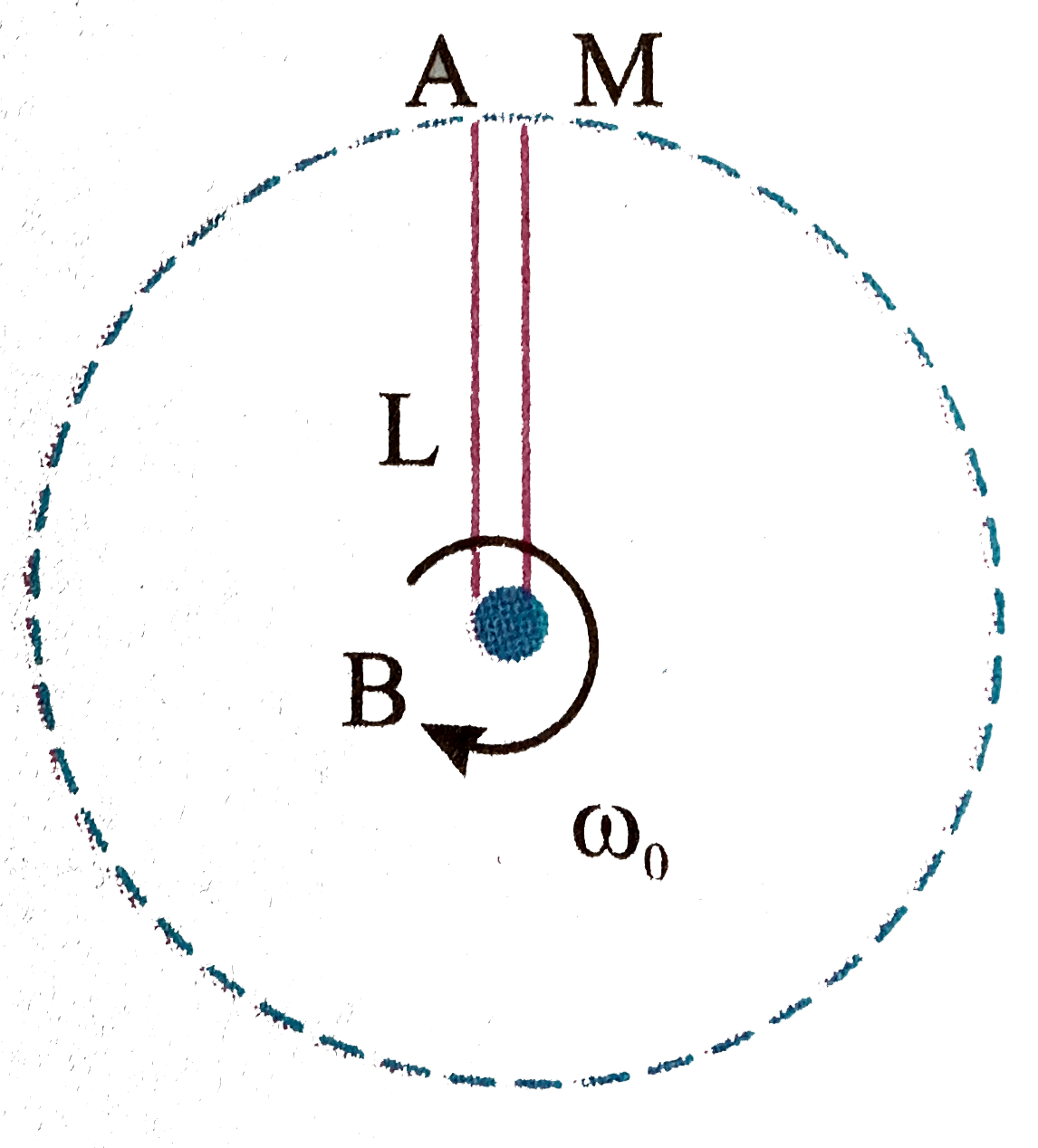
View SolutionA thin rod AB of mass M and length L is rotating with angular speed ω0 about vertically axis passing through its ends B on a horizontal smooth table as shown. If at some instant the hinge at end B of rod is opened then which of the following statemtents is / are correct about motion of rod?
View SolutionA uniform rod of mass m and length l is rotating with constant angular velocity ω about an axis which passes thorugh its one end and perpendicular to the length of rod. The area of cross section of the rod is A and its Young's modulus is y. Neglect gravity. The strain at the mid point of the rod is:
View Solution
