A uniform rod of mass m and length l is rotating with constant angular velocity ω about an axis which passes thorugh its one end and perpendicular to the length of rod. The area of cross section of the rod is A and its Young's modulus is y. Neglect gravity. The strain at the mid point of the rod is:
mn2l8Ay
3mω2l8Ay
3mω2l4Ay
mω4Ay
dT=dm(l−x)ω2
dT=mldx(l−x)ω2
⇒∫T0dT=∫l/20mω2l(l0x)dx
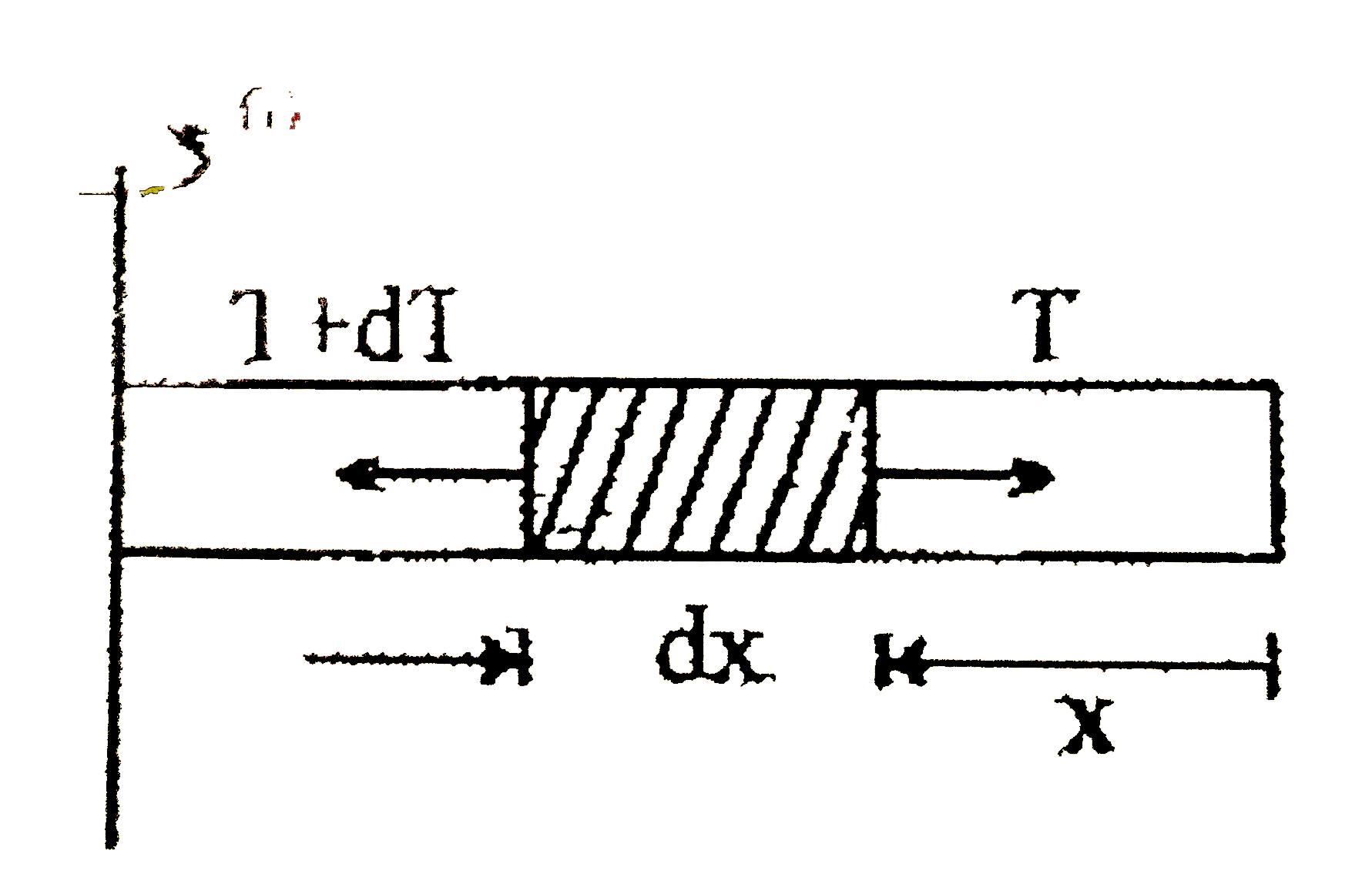
mω2l[lx−x22]l/20=mω2l[l22−l2g]
∴ Tension at mid point is:
T=38mlω2⇒ stress =3mlω28A
⇒ strain 3mlω28AY
Alternatively Tension at mid point can be found by using

Fext=macm
T=m2(ω23l4)=38mω2l
Topper's Solved these Questions
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART (A)
Explore 32 Videos
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART (B)
Explore 3 Videos
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART- C
Explore 4 Videos
- SEMICONDUCTORSBook:BANSALChapter:SEMICONDUCTORSExercise:CBSE Question
Explore 32 Videos
Similar Questions
A uniform rod of mass m and length l0 is rotating with a constant angular speed ω about a vertical axis passing through its point of suspension. Find the moment of inertia of the rod about the axis of rotation if it make an angle θ to the vertical (axis of rotation).
View SolutionA thin uniform rod of mass m and length L rotates with the constant angular velocity ω about .the vertical axis passing through the rod's suspensjon point O. It describes a carried surface, then:
View Solution
Knowledge Check
A uniform rod of length l is rotating in a gravity free region about an axis passing through its one end and perpendicular to it length. Choose graph between stress developed and distance the axis of roation.
A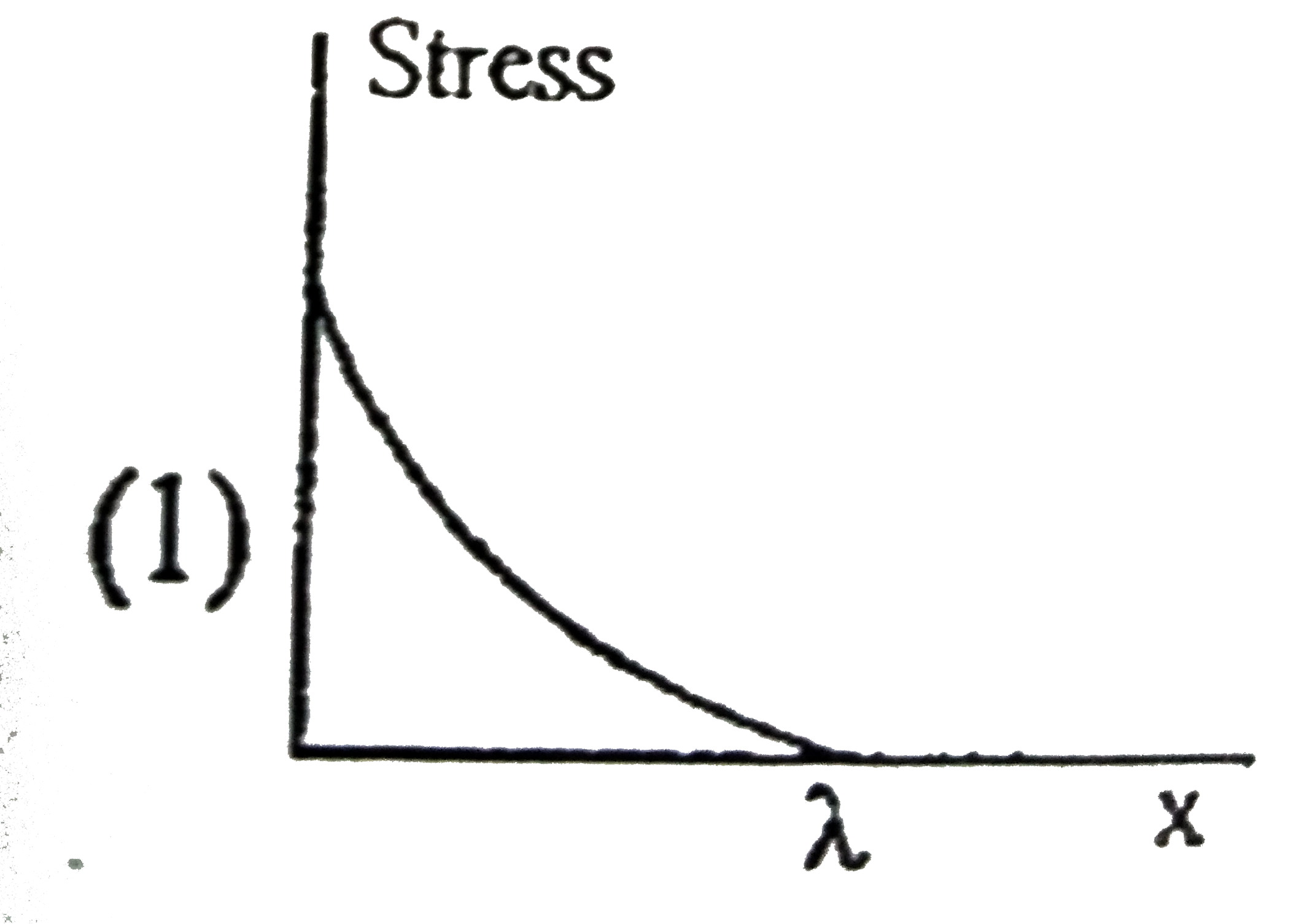 B
B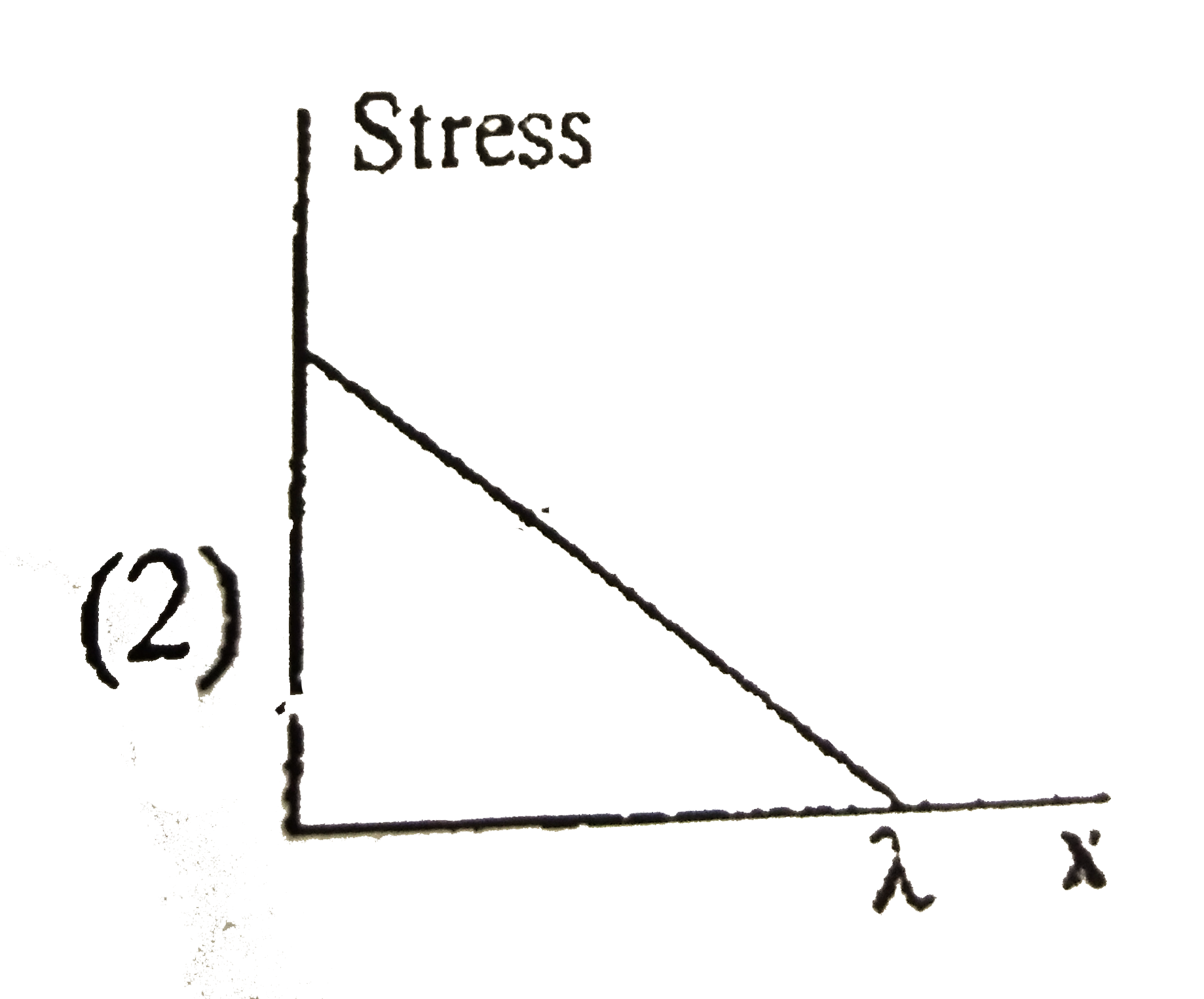 C
C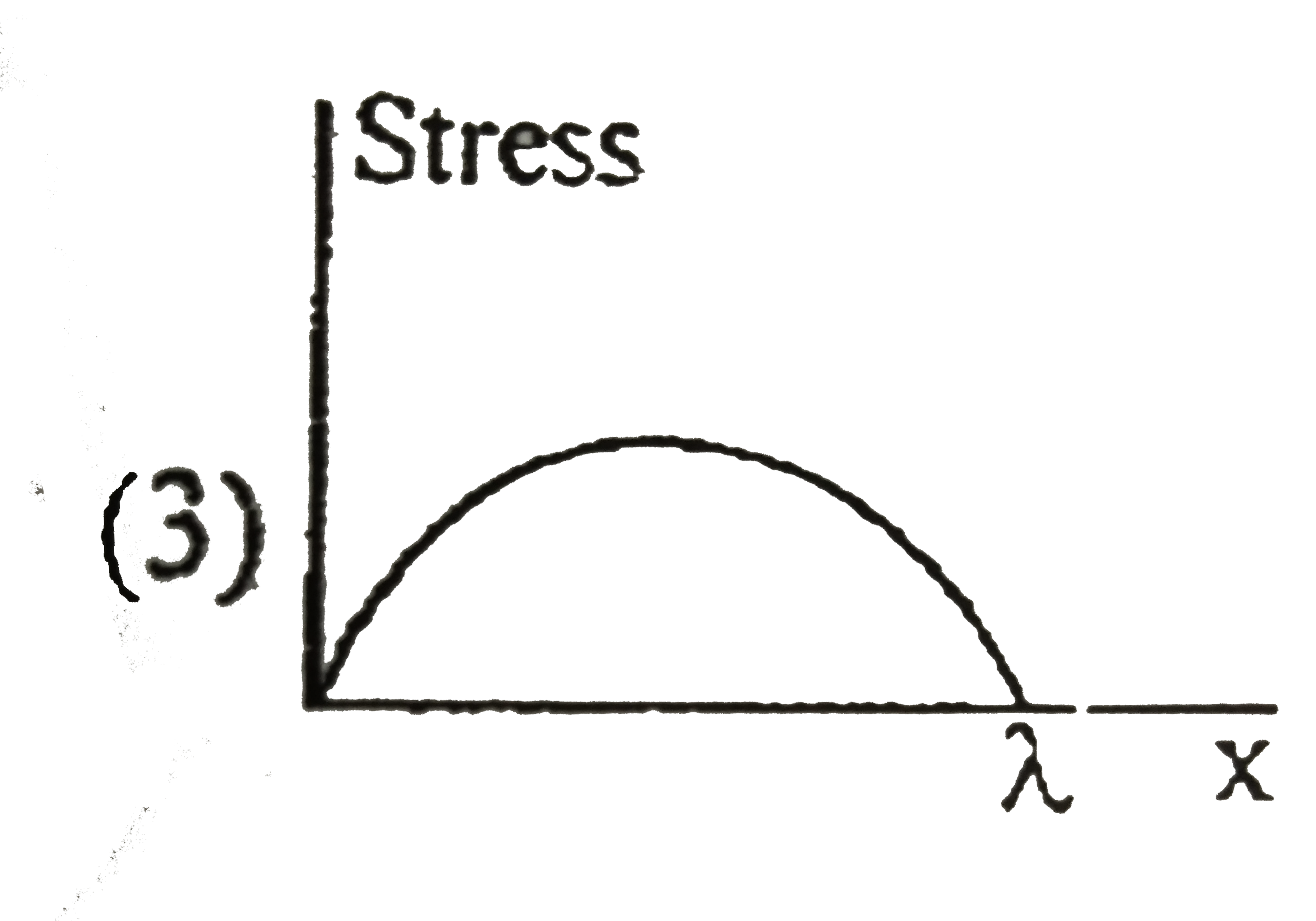 D
D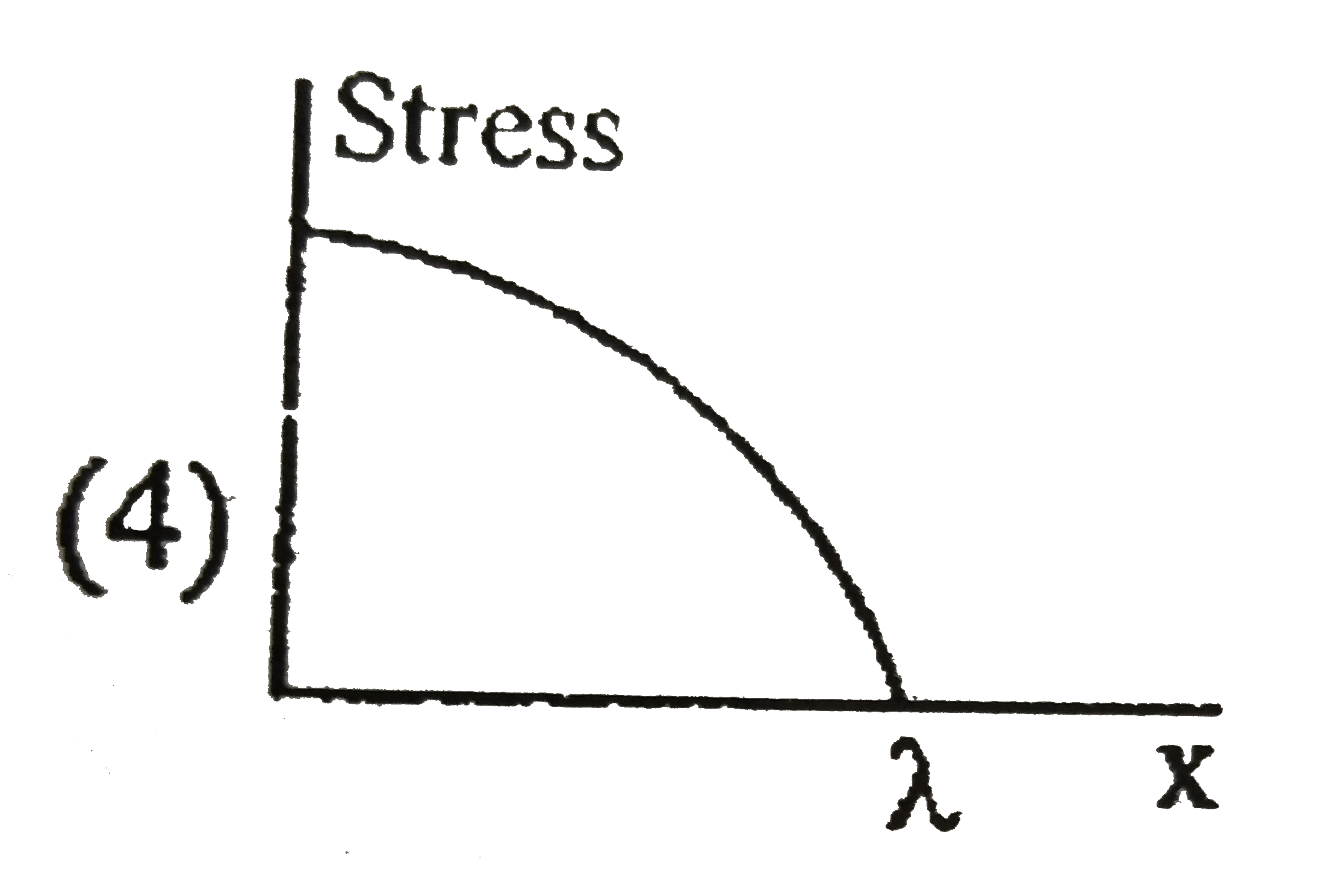
A uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
A12mω2xB12mω2(1−x2l)C12mω2l(1−x2l2)D12mω2l[1−xl]The radius of gyration of a uniform rod of length l about an axis passing through one of its ends and perpendicular to its length is
Al√2Bl3Cl√3Dl2
A uniform rod of mass m and length l makes a constant angle θ with an axis of rotation which passes through one end of the rod. Its moment of inertia about this axis is
View SolutionA uniform rod of mass m and length / makes a constant angle θ with an axis of rotation which passes through one end of the rod. Its moment of inertia about this axis is
View SolutionThe radius of gyration of an uniform rod of length L about an axis passing through its centre of mass and perpendicular to its length is.
View SolutionA conducting rod rotates with a constant angular velocity 'ω' about the axis which passes through point 'O' and perpendicular to its length . A uniform magnetic field 'B' exists parallel to the axis of the rotation . Then potential difference between the two ends of the rod is :-
View SolutionA uniform metal rod of length 1 and mass m is rotating with an angular velocity of magnitude o about an axis passing through its centre and perpendicular to the rod. If the temperature of the rod increases by T∘C , then change in its magnitude of angular velocity varies directly with
View Solution