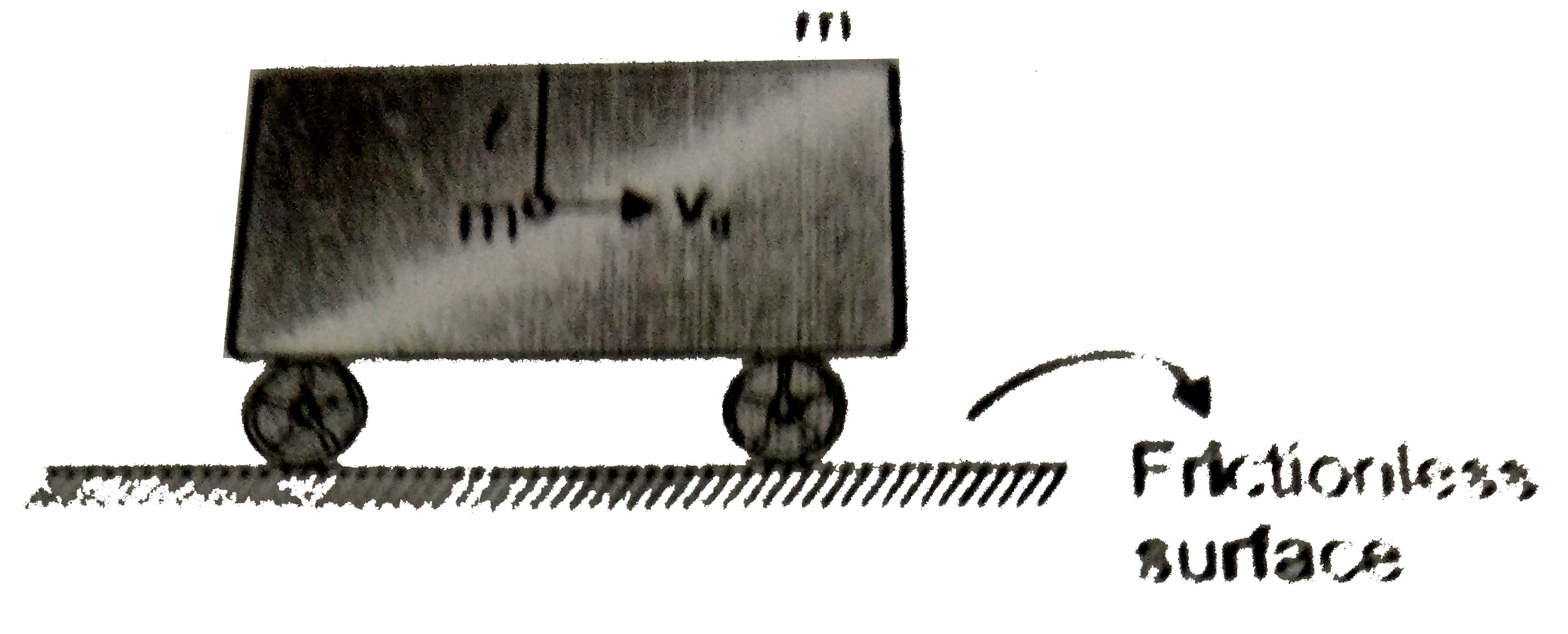
A small bob mass 'm' is suspended by a massless string from a cart of the same mass 'm' as shown in the figure. The friction between the cart and horizontal ground is negligible. The bob is given a velocity V0 in horizontal direction as shown. The maximum height attained by the bob is , ( initially whole system ( bob + string + cart ) was at rest ).
2V20g
V20g
V204g
V202g
The correct Answer is:C
A small bob of
By linear momentum conservation in horizontal direction = for (bot +string+cart)
v=v02
By mechanical energy conservation for
(bob+string +cart +earth)
12mV2a+0+0=12(2m)v2+mgh+0
12mV20−12(2m)V204=mgh
Solving it h=V204g
Topper's Solved these Questions
Similar Questions
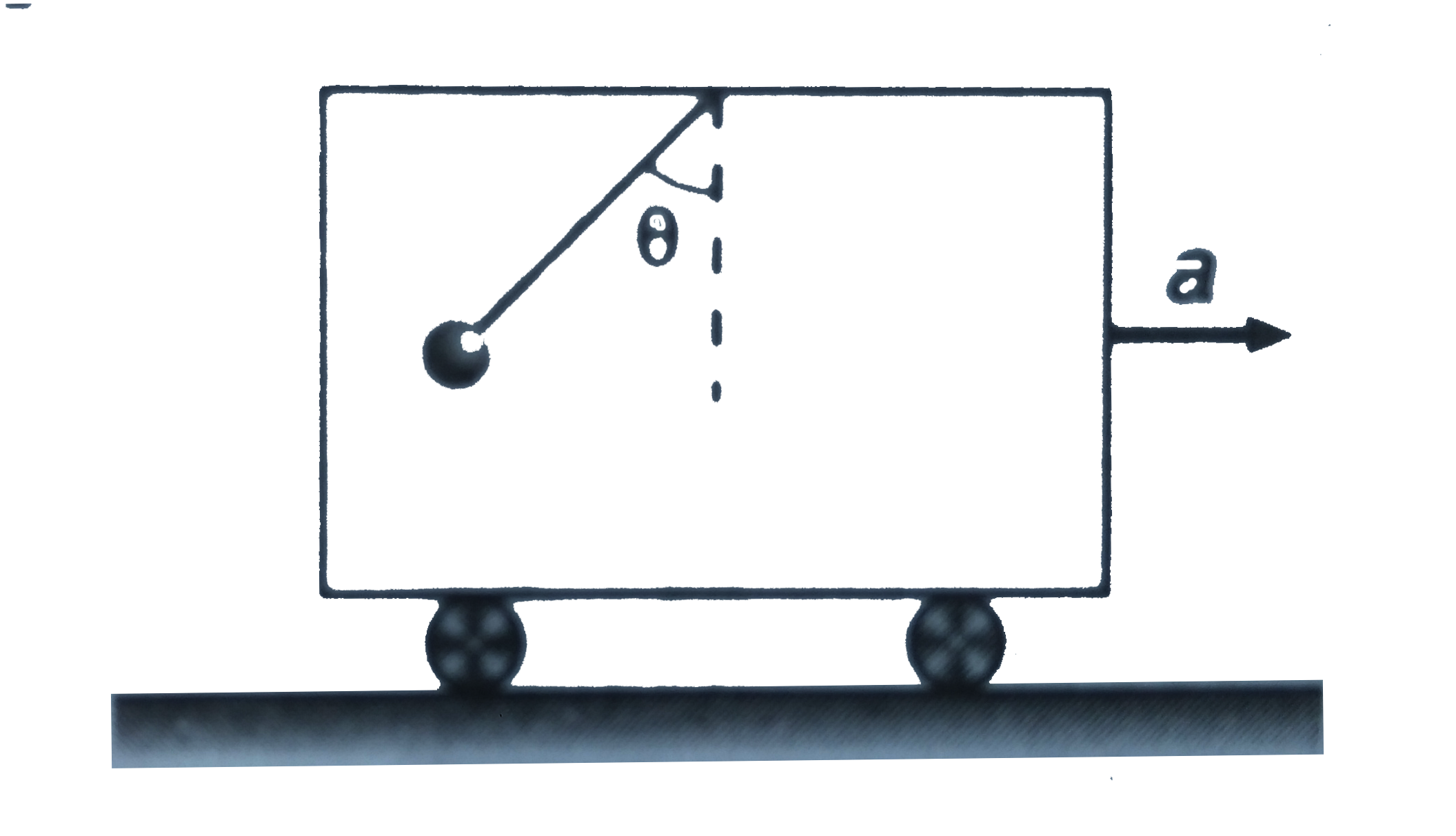
A bob of mass m is suspended from the ceiling of a train moving with an acceleration a as shown in figure. Find the angle θ in equilibrium position.
View SolutionA bob of mass m is hanging from a cart of mass M. System is relased from rest from the position shown. Find the maximum speed of the cart with respect to ground.
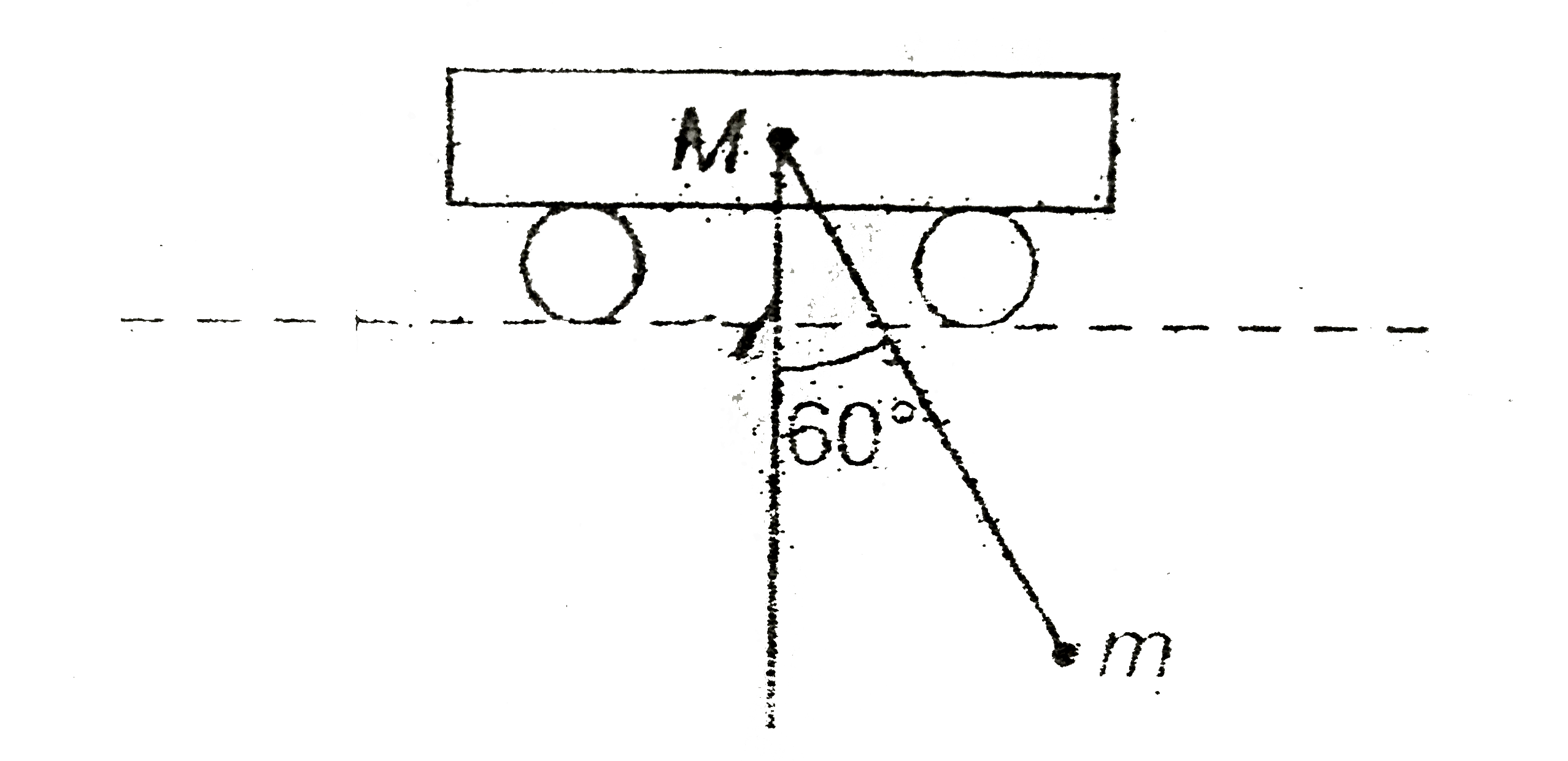
View Solution
Knowledge Check
The block of mass m is in contact with a moving cart as shown in the figure.The coefficient of friction between the block and the cart is . The acceleration a of the cart that will prevent the block from falling is
 Aα≥gμBα<gμCα>mgμDα>gμm
Aα≥gμBα<gμCα>mgμDα>gμmA pendulum bob 'm' is released from rest from horizontal position as shown in the figure. ltbr> Choose the position where the chance of. Braking of the string is maximum.
 AABBCCDD
AABBCCDDA bob in tied to a string of length l & and kept on a horizontal rough surface as shown in the figure when point O of the string is fixed. The bob is given a tangential velocity V0 keeping the string taut as shown in figure. Find the tension in the string as a function of time. The coefficient of kinetic friction is μ.
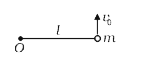 Am(v0−μgt)2/1Bm(v0+μgt)2/1Cm(v0+>)2/1Dm(v0−>)2/1
Am(v0−μgt)2/1Bm(v0+μgt)2/1Cm(v0+>)2/1Dm(v0−>)2/1
Bob B of the pendulum AB is given an initial velocity √3Lg in horizontal direction. Final the maximum height of the bob from the starting point,
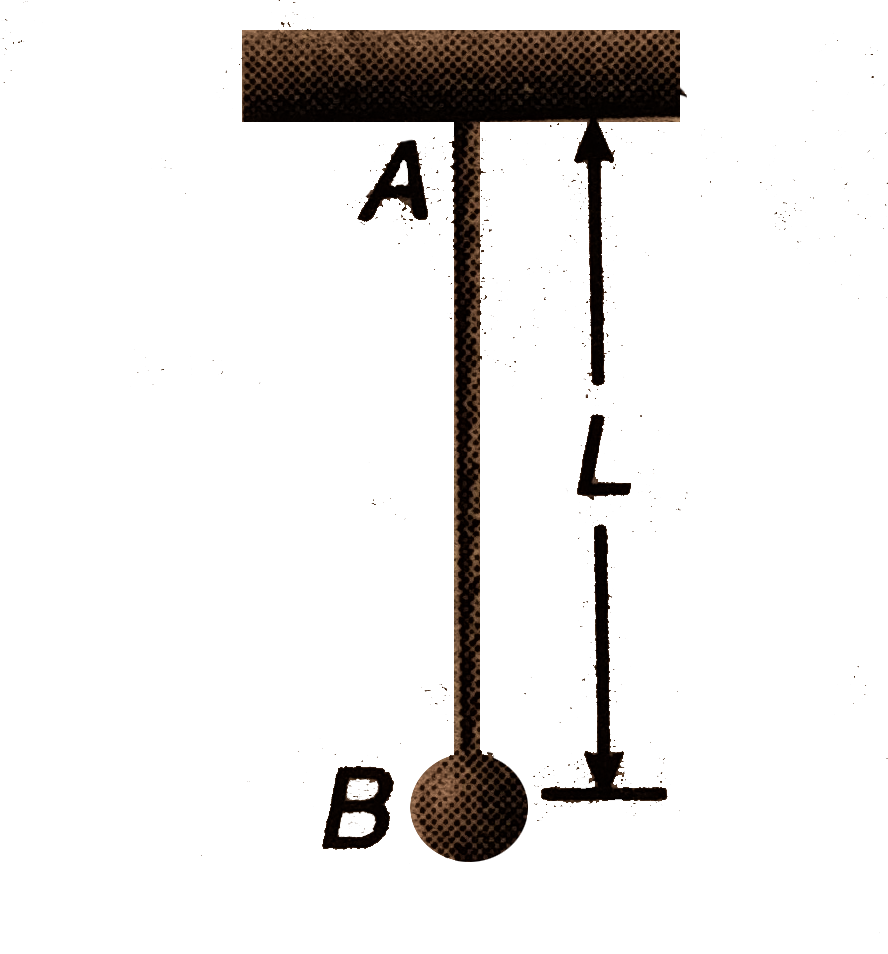
(a) if AB is a massless rod,
(b) if AB is a massless staring.
View SolutionA ball B is suspended from a string of length l attached to a cart A, which may roll on a frictionless surface. Initially the cart is at rest and the ball is given a horizontal velocity v0. Determine
the velocity of B as it reaches the maximum height.
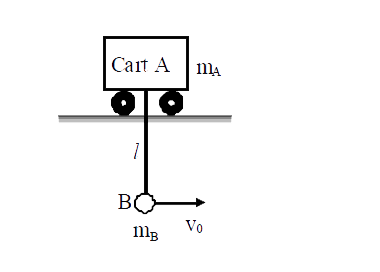
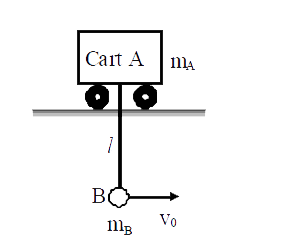
View SolutionA ball B is suspended from a string of length l attached to a cart A, which may roll on a frictionless surface. Initially the cart is at rest and the ball is given a horizontal velocity v0. Determine
the maximum height reached by the ball.
View SolutionA cart of mass M has a block of mass m in contact with it as shown in the figure-2.133. The coefficient of friction between the block and the cart is μ. What is the minimum acceleration of the cart so that the block m does not fall ?

View SolutionA small ball of mass m collides elastically with a simple pendulum with bob of mass ma at rest. The macimum height attained by the bob after collision is
View Solution
