Figure shows a small loop carrying a current I. The curved portion is an arc of a circle of radius R and the straight portion is a chord to the same circle subtending an angle θ.The magnetic induction at centre O is

zero
always inward irrespective of the value of θ
inward as long as θ is less than π
always outward irrespective of the value of θ
The correct Answer is:C
The magnetic field due to finite wire at the centre of the loop will be
B1=μ0I4πR(cosθ)(sinθ+sinθ) (From =μ0I2πRtanθ
Right hand thumb rule, the magnetic field will be inward)
the magnetic field due to the circular loop will be
B2=μ0I4πRθ (The magnetic field will be outward direction)
The net magnetic field at the centre is
B=B1−B2
B=μ0I4πR(2tanθ−θ)
For θ≥π,2tanθ−θ<0, therefore magnetic field will be outward for
θ≥π and inward for θ≤π.
Topper's Solved these Questions
Similar Questions
A wire is bent in the form of a circular arc of radius r with a straight portion AB. If the current in the wire is i, then the magnetic induction at point O is
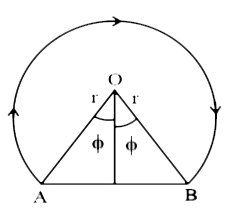
View SolutionA wire is bent in the form of a circular arc of radius r with a straight portion AB. If the current in the wire is i, then the magnetic induction at point O is

View Solution
Knowledge Check
Consider a circle of radius R. What is the lengths of a chord which subtends an angle θ at the centre ?
A2Rsin(θ2)B2RsinθC2Rtan(θ2)D2RtanθConsider a circle of radius R. What is the lengths of a chord which subtends an angle θ at the centre ?
A2Rsin(θ2)B2RsinθC2Rtan(θ2)D2RtanθIn the figure, the curved part represents arc of a circle of radius x. If it carries a current I then the magnetic field at the point O is
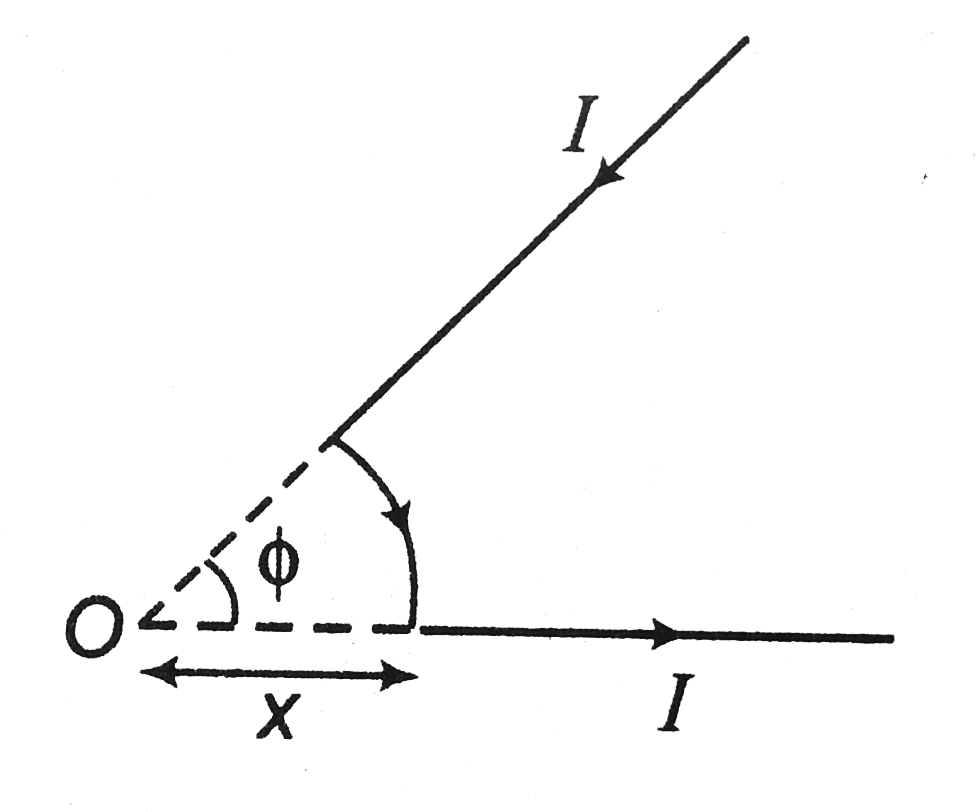 Aμ0Iϕ2πxBμ0Iϕ4πxCμ0Iϕ2xDμ0Iϕ4x
Aμ0Iϕ2πxBμ0Iϕ4πxCμ0Iϕ2xDμ0Iϕ4x
What is the area of a segment of a circle of radius r subtending an angle θ at the centre ?
View SolutionIn the figure, the curved part represents arc of a circle of radius x. If it carries a current I then the magnetic field at the point O is

View SolutionTwo very long wires carrying equal current i are bent and arranged as shown in figure. Both AB and CD are arcs of the same circle, both subtending right angles at the centre O. The magnetic field at O is:

View SolutionA circular arc of wire of radius of curvature r subtends an angle of p/4 radian at its centre. If i current is flowing in it then the magnetic induction at its centre is -
View SolutionThe chord of a circle is equal to its radius, find the angle subtended by this chord at the centre.
View Solution
