If −1<3+4x≤23,x∈R (real numbers), then the solution set of x is

A

−1≤x<5,x∈R

B

−1<x≤5,x∈R

C

−1≤x≤5,x∈R

D

−1<x<5,x∈R

Text Solution
Verified by Experts

The correct Answer is:B

Updated on:21/07/2023
Doubtnut Promotions Banner
|
Class 10MATHSLINEAR INEQUATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • If 2x−3≤x+1≤4x+7,x∈I (integers), then the solution set of x is

    A{−2,−1,0,1,2,3,4}
    B{−1,0,1,2,3}
    C{−2,−1,0,1,2,3}
    D{−1,0,1,2,3,4}

  • If 2x−5≤5x+4≤11 and x is a natural number (N), then the solution set of x is

    A{1}
    B{−3,−2,−1,0,1}
    C{−2,−1,0,1}
    D{−2,−1,0}

  • Assertion: The solution set of −1<3+4x≤23,x∈R on the number line is
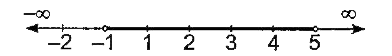
    ocr_image
    Reason: In the solution set, the number -1 is not included and the number 5 is included.

    ABoth assertion and reason are correct and reason is the correct explanation of assertion
    BBoth assertion and reason are correct but reason is not the correct explanation of assertion
    CAssertion is correct but reason is incorrect
    DAssertion is incorrect but reason is correct