A pendulum bob of mass 50 gm is suspended from the ceiling of an elevator. The tension in the string if the elevator goes up with uniform velocity is approximately

0.30 N

0.40 N

0.42 N

0.50 N

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

$T = m g = 0.05 \times 10 = 0.5 N$

Updated on:21/07/2023

Class 11 PHYSICS NEWTONS LAW OF MOTION

Topper's Solved these Questions

BOOK - ERRORLESSCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - NCERT BASED QUESTION (THIRD LAW OF MOTION)
11videos
NEWTON'S LAWS OF MOTION
BOOK - ERRORLESSCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - NCERT BASED QUESTION (CONSERVATION OF LINEAR MOMENTUM AND IMPULSE)
29videos
NEWTON'S LAWS OF MOTION
BOOK - ERRORLESSCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - ASSERTION & REASON
18videos
NEWTON'S LAWS OF MOTION
BOOK - ERRORLESSCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - ASSERTION & REASON
19videos
MOTION IN TWO DIMENSION
BOOK - ERRORLESSCHAPTER - ROTATIONAL MOTIONEXERCISE - Assertion & Reason
25videos
ROTATIONAL MOTION

Knowledge Check

Question 1 - Select One
A simple pendulum of length 1 m is freely suspended from the ceiling of an elevator. The time period of small oscillations as the elevator moves up with an acceleration of $2 m / s^{2}$ is (use g=10 $m / s^{2}$ )
A $\frac{π}{\sqrt{5}}$ s
B $\sqrt{\frac{2}{5}} π s$
C $\frac{π}{\sqrt{2}}$ s
D $\frac{π}{\sqrt{3}}$ s
Question 2 - Select One or More
Two blocks of masses $m_{1}$ and $m_{2} (m_{1} > m_{2})$ are connected by a massless threads, that passes over a massless smooth pulley. The pulley is suspended from the ceiling of an elevator. Now the elevator moves up with uniform velocity $v_{0}$ Now, select the correct options:

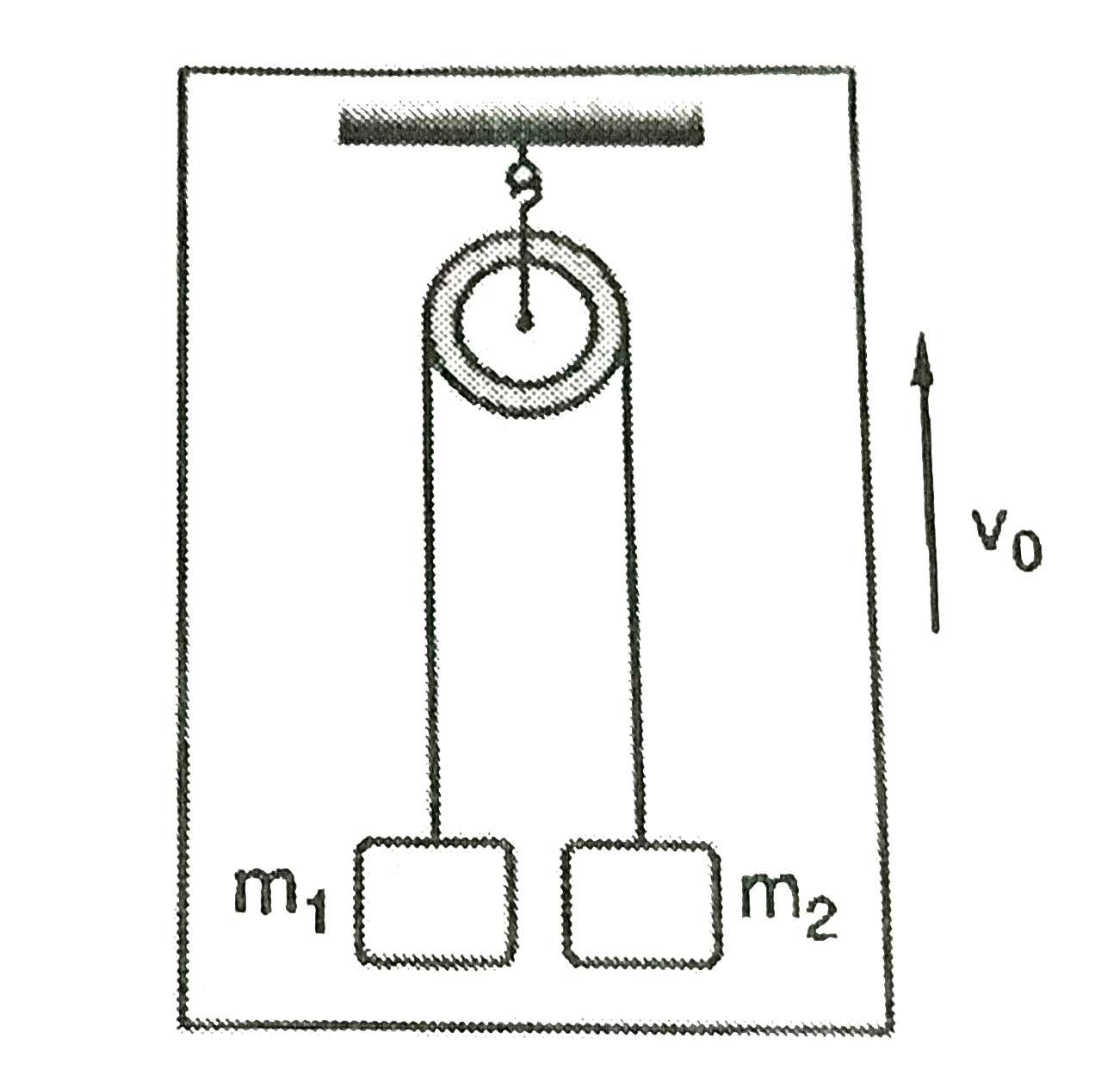AMagnitude of acceleration of $m_{1}$ with respect to ground is greater than $\frac{(m_{1} - m_{2}) g}{m_{1} + m_{2}}$
BMagnitude of acceleration of $m_{1}$ with respect to ground is equal to $\frac{(m_{1} - m_{2}) g}{m_{1} + m_{2}}$
CTension in the thread that connects $m_{1}$ and $m_{2}$ is equal to $\frac{2 m_{1} m_{2} g}{m_{1} + m_{2}}$
DTension in the thread that connects $m_{1}$ and $m_{2}$ is greater than $\frac{2 m_{1} m_{2} g}{m_{1} + m_{2}}$
Question 3 - Select One or More
Two blocks of masses $m_{1}$ and $m_{- 2} m > (m_{2})$ are connected by massless threads that passes over a massless smooth pulley The pulley is suspended from the ceiling of an elevator Now the elevator moves up with uniform velocity $V_{0}$ Now select the correct options
.
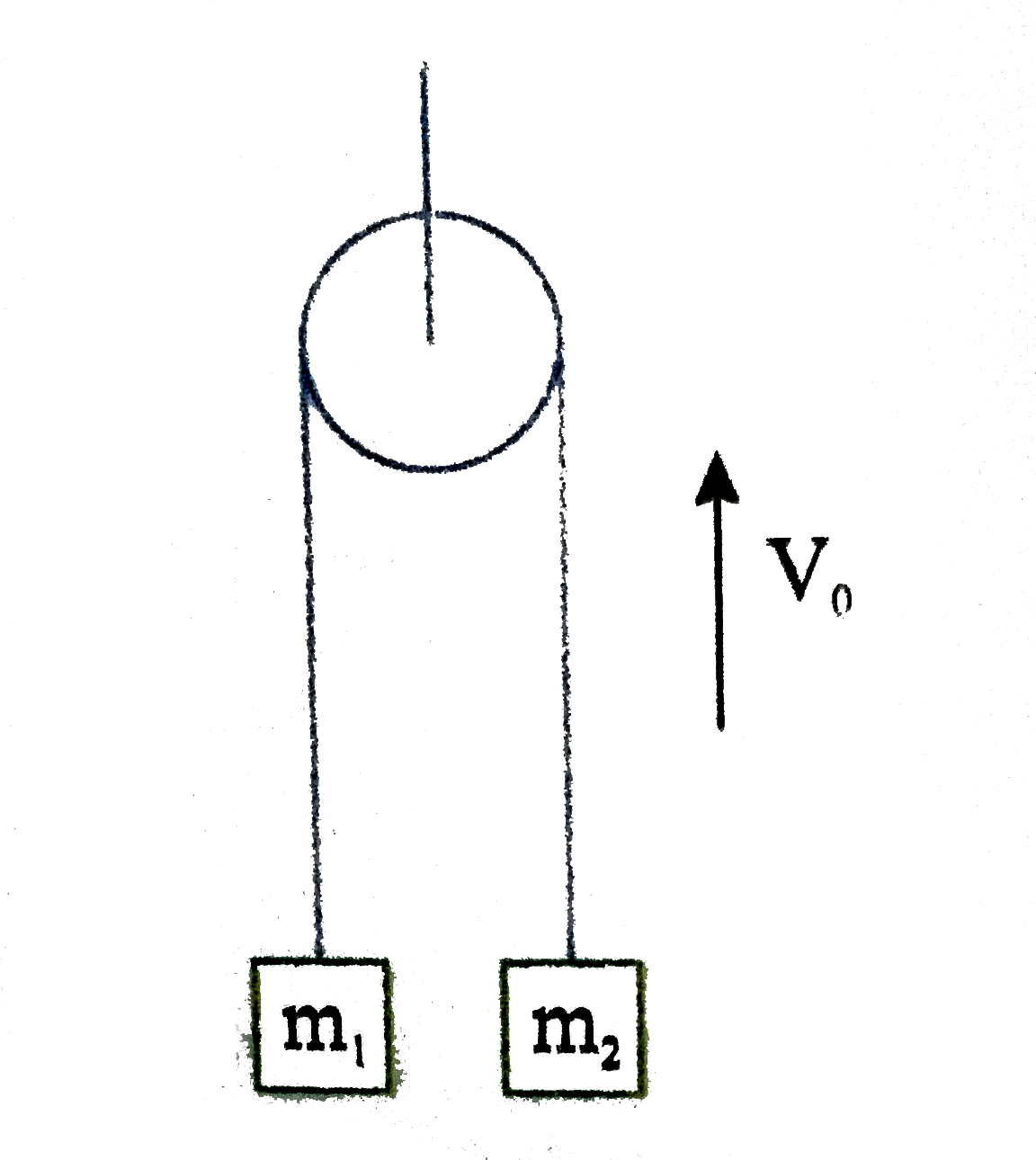AMagnitude of acceleration of $m_{1}$ with respect to ground is greate than $\frac{(m_{1} - m_{2}) g}{m_{1} + m_{2}}$
BMagnitude of acceleration of $m_{1}$ with respect to ground is greate than $\frac{(m_{1} - m_{2}) g}{m_{1} + m_{2}}$
CTension in the thread that connects $m_{1}$ and $m_{2}$ is equal to $\frac{2 m_{1} m_{2} g}{m_{1} + m_{2}}$ .
DTension in the thread that connects $m_{1}$ and $m_{2}$ is equal to $\frac{2 m_{1} m_{2} g}{m_{1} + m_{2}}$ .

ERRORLESS-NEWTON'S LAWS OF MOTION-NCERT BASED QUESTION ( SECOND LAW OF MOTION)

Home
Ask Doubt
Profile

A pendulum bob of mass 50 gm is suspended from the ceiling of an elevator. The tension in the string if the elevator goes up with uniform velocity is approximately

The correct Answer is:D

T=mg=0.05×10=0.5N

Topper's Solved these Questions

Similar Questions

Knowledge Check

A simple pendulum of length 1 m is freely suspended from the ceiling of an elevator. The time period of small oscillations as the elevator moves up with an acceleration of 2m/s2 is (use g=10m/s2)

Two blocks of masses m1 and m2(m1>m2) are connected by a massless threads, that passes over a massless smooth pulley. The pulley is suspended from the ceiling of an elevator. Now the elevator moves up with uniform velocity v0 Now, select the correct options:

Two blocks of masses m1 and m−2m>(m2) are connected by massless threads that passes over a massless smooth pulley The pulley is suspended from the ceiling of an elevator Now the elevator moves up with uniform velocity V0 Now select the correct options .

ERRORLESS-NEWTON'S LAWS OF MOTION-NCERT BASED QUESTION ( SECOND LAW OF MOTION)

$T = m g = 0.05 \times 10 = 0.5 N$

A simple pendulum of length 1 m is freely suspended from the ceiling of an elevator. The time period of small oscillations as the elevator moves up with an acceleration of $2 m / s^{2}$ is (use g=10 $m / s^{2}$ )

Two blocks of masses $m_{1}$ and $m_{2} (m_{1} > m_{2})$ are connected by a massless threads, that passes over a massless smooth pulley. The pulley is suspended from the ceiling of an elevator. Now the elevator moves up with uniform velocity $v_{0}$ Now, select the correct options:

Two blocks of masses $m_{1}$ and $m_{- 2} m > (m_{2})$ are connected by massless threads that passes over a massless smooth pulley The pulley is suspended from the ceiling of an elevator Now the elevator moves up with uniform velocity $V_{0}$ Now select the correct options
.