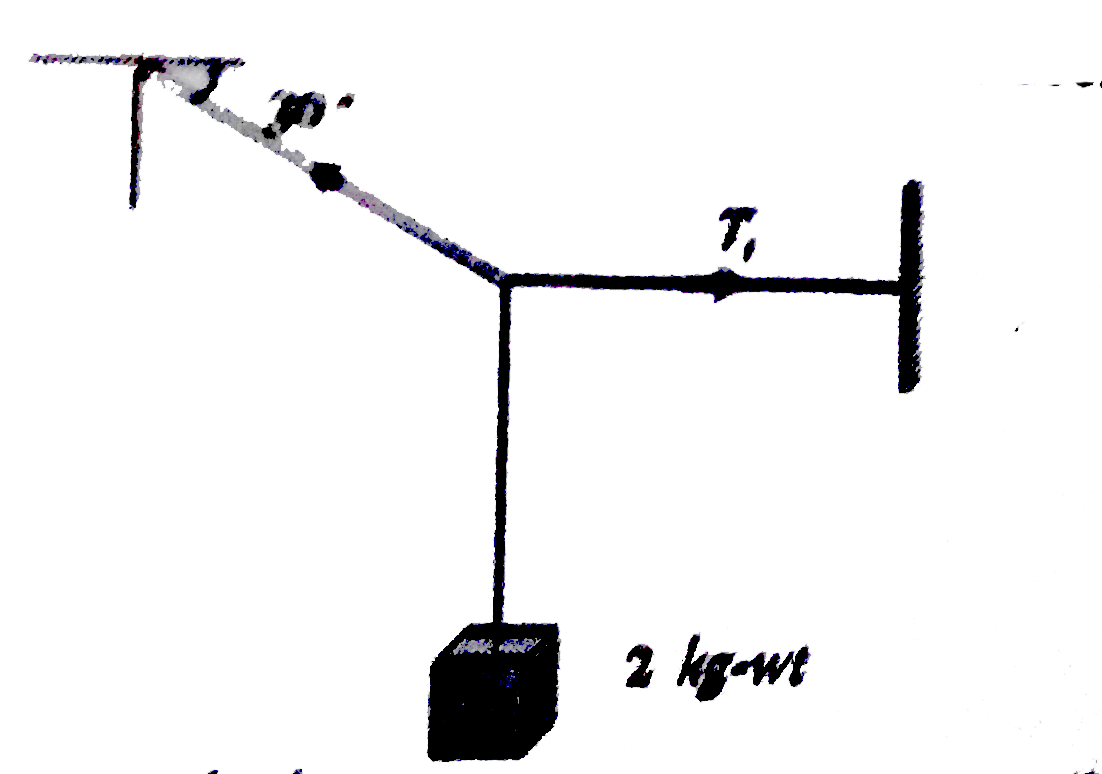
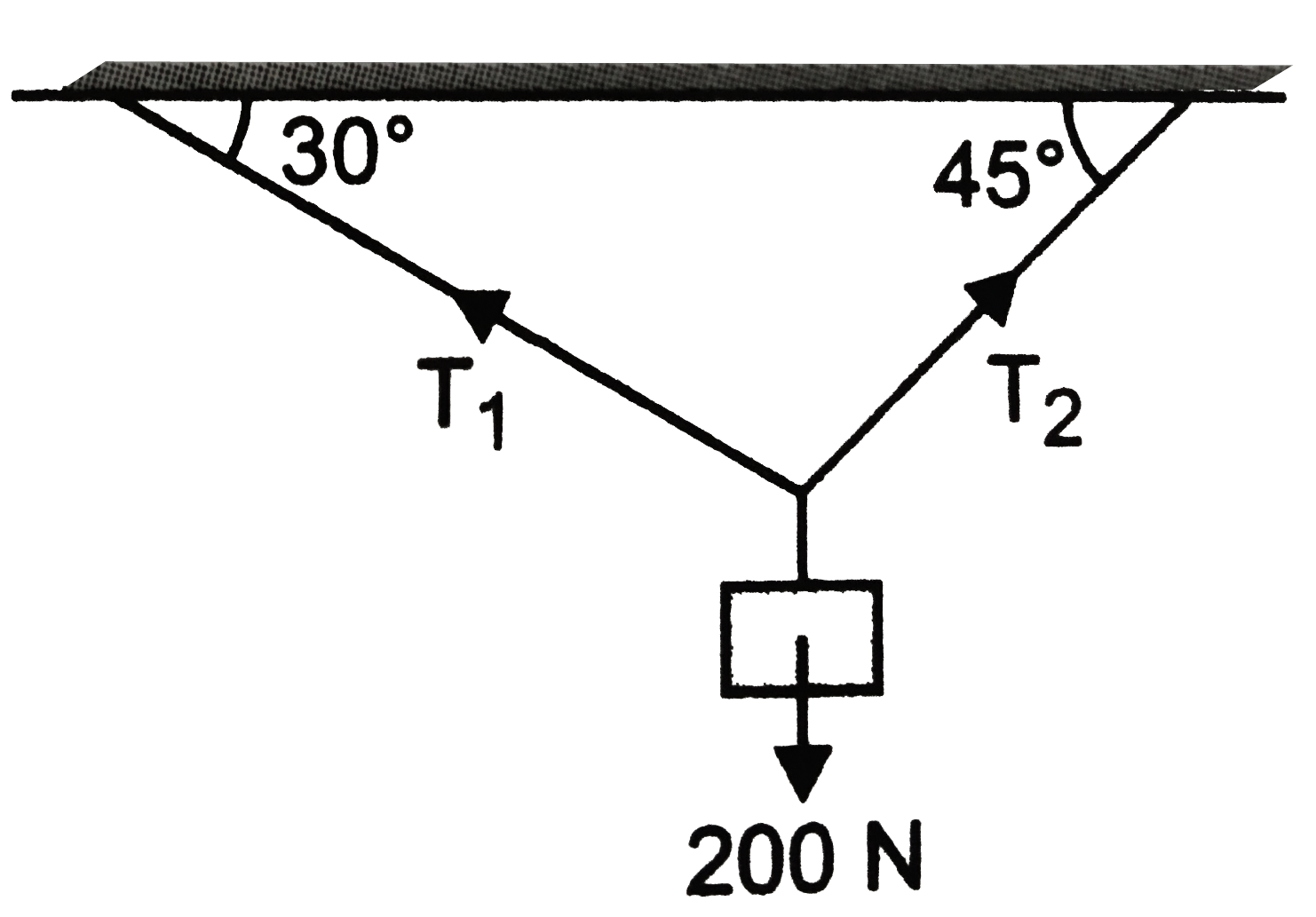
A body of weight 2 kg is suspended as shown in the figure. The tension T1 in the horizontal string (in kg wt ) is
A
2/√3
B
√3/2
C
2√3
D
2
Video Solution
Text Solution
The correct Answer is:C
Tcos60∘=20
⇒T=40N
Tsin60∘=T1
⇒T1=40×√32=20√3
⇒T1=2√3 kg wt

|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - ERRORLESSCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - PAST YEARS QUESTIONS14videos
NEWTON'S LAWS OF MOTION
- BOOK - ERRORLESSCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - ASSERTION & REASON18videos
NEWTON'S LAWS OF MOTION
- BOOK - ERRORLESSCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - NCERT BASED QUESTION (EQUILIBRIUM OF FORCES)4videos
NEWTON'S LAWS OF MOTION
- BOOK - ERRORLESSCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - ASSERTION & REASON19videos
MOTION IN TWO DIMENSION
- BOOK - ERRORLESSCHAPTER - ROTATIONAL MOTIONEXERCISE - Assertion & Reason25videos
ROTATIONAL MOTION
Similar Questions
Knowledge Check
- Question 1 - Select One
A block of mass 10 kg is suspended by three strings as shown in figure . Tension T2 is
 A100NB100√3NC√3×100ND50√3N
A100NB100√3NC√3×100ND50√3N - Question 2 - Select One
Three equal weight A,B and C of mass 2kg each are hanging on a string passing over a fixed frictionless pulley as shown in the figure. The tension in the string connecting weights B and C is approximately
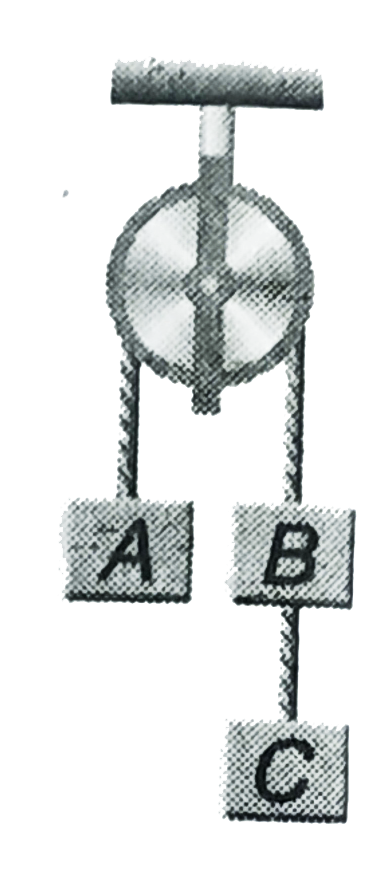 AzeroB13NC3.3ND19.6N
AzeroB13NC3.3ND19.6N - Question 3 - Select One
Two blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.
 A188 NB2=368 NC288 ND168 N
A188 NB2=368 NC288 ND168 N
A block of weight 100 N is suspended (as shown) with the help of there strings. Find the tensions in the three strings).
View SolutionIn the shown figure the tension in the horizontal cord is 30N. Find the weight of the body B.
 View Solution
View SolutionA 'block' of mass 10 kg is suspended with string as shown in figure. Find tension in the string. (g=10m/s2)
 View Solution
View SolutionA block of mass 10 kg is suspended with two strings as shown in the fig. Find the tensions T1andT2 in the two string.
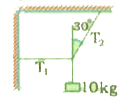 View Solution
View SolutionA block of mass 30 kg is suspended by three string as shown in fig, Find the tension in each string.
 View Solution
View Solution


 .
.