The mode of a grouped frequency distribution is 75. and the modal class is 65-80. The frequency of the class preceding the modal class is 6 and the frequency of the class succeeding the modal class is 8. Find the frequency of the modal class.
To find the frequency of the modal class given the mode, modal class, and the frequencies of the classes preceding and succeeding the modal class, we can use the formula for the mode in a grouped frequency distribution.
1. Identify the given values:
- Mode (Mo) = 75
- Modal class = 65-80
- Lower limit of modal class (L) = 65
- Frequency of the class preceding the modal class (F0) = 6
- Frequency of the class succeeding the modal class (F2) = 8
- Height of the modal class (h) = 80 - 65 = 15
2. Use the mode formula:
The formula for the mode in a grouped frequency distribution is:
Mo=L+F1−F0(F1−F0)+(F1−F2)×h
where:
- Mo = mode
- L = lower limit of the modal class
- F1 = frequency of the modal class (unknown)
- F0 = frequency of the class before the modal class
- F2 = frequency of the class after the modal class
- h = width of the modal class
3. Substitute the known values into the formula:
75=65+F1−6(F1−6)+(F1−8)×15
4. Simplify the equation:
First, simplify the denominator:
(F1−6)+(F1−8)=2F1−14
Now, substitute this back into the equation:
75=65+F1−62F1−14×15
5. Isolate the fraction:
Subtract 65 from both sides:
10=F1−62F1−14×15
Now, divide both sides by 15:
1015=F1−62F1−14
Simplifying 1015 gives 23:
23=F1−62F1−14
6. Cross-multiply to solve for F1:
2(2F1−14)=3(F1−6)
Expanding both sides:
4F1−28=3F1−18
7. Rearranging the equation:
Subtract 3F1 from both sides:
4F1−3F1=28−18
This simplifies to:
F1=10
Final Answer:
The frequency of the modal class (F1) is 10.
- Mode (Mo) = 75
- Modal class = 65-80
- Lower limit of modal class (L) = 65
- Frequency of the class preceding the modal class (F0) = 6
- Frequency of the class succeeding the modal class (F2) = 8
- Height of the modal class (h) = 80 - 65 = 15
The formula for the mode in a grouped frequency distribution is:
Mo=L+F1−F0(F1−F0)+(F1−F2)×h
where:
- Mo = mode
- L = lower limit of the modal class
- F1 = frequency of the modal class (unknown)
- F0 = frequency of the class before the modal class
- F2 = frequency of the class after the modal class
- h = width of the modal class
75=65+F1−6(F1−6)+(F1−8)×15
First, simplify the denominator:
(F1−6)+(F1−8)=2F1−14
Now, substitute this back into the equation:
75=65+F1−62F1−14×15
Subtract 65 from both sides:
10=F1−62F1−14×15
Now, divide both sides by 15:
1015=F1−62F1−14
Simplifying 1015 gives 23:
23=F1−62F1−14
2(2F1−14)=3(F1−6)
Expanding both sides:
4F1−28=3F1−18
Subtract 3F1 from both sides:
4F1−3F1=28−18
This simplifies to:
F1=10
The frequency of the modal class (F1) is 10.
Topper's Solved these Questions
Similar Questions
Knowledge Check
For the following distribution
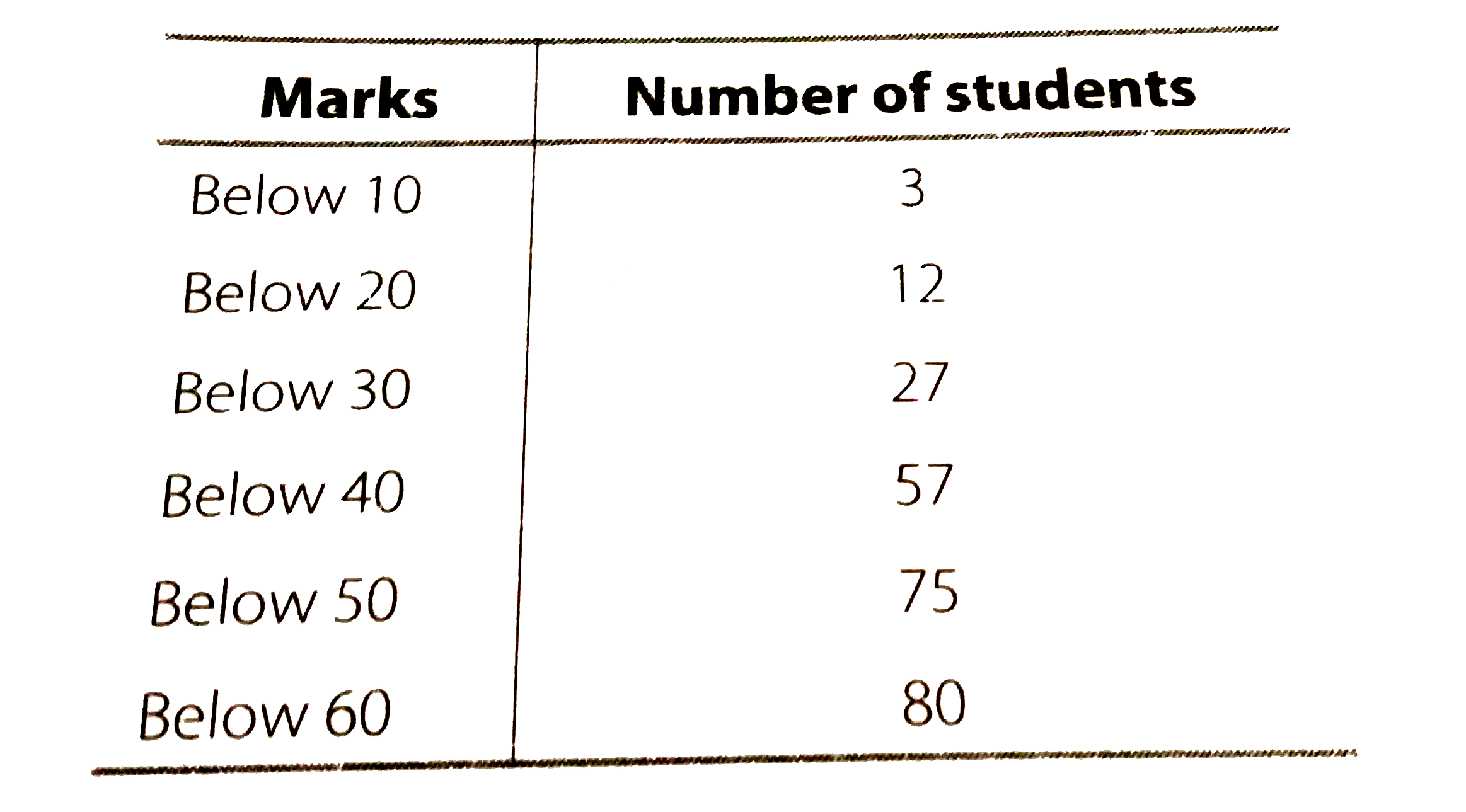
the modal class isA40-50B20-30C30-40D50-60If the greater than cumulative frequency of a class is 60 and that of the next class is 40 , then find the frequency of that class .
A10B20C50D30How many classes a frequency distribution should have?
ALarge numberBSmall numberCNeither very large nor very small numberDNot more than 10.
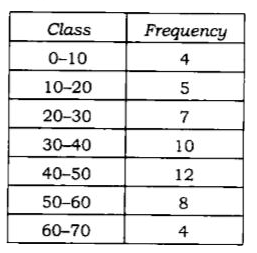
Consider the following frequency distribution
What is the median class?
View SolutionThe mean of the following distribution is 18. Find the frequency f of the class 19−21.
View SolutionFind the sum of the lower limit of the median class and the upper limit of the modal class.
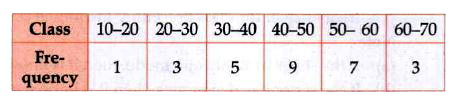
View SolutionIn the following frequency distribution, find the median class.

What is the upper limit of median class ?
View SolutionIn a frequency distribution, the mid values of a class is 10 and width of the class is 6. The lower limit of the class is
View Solution

