In the figure AE bisects and , prove that .

The correct Answer is:AE||BC
Topper's Solved these Questions
- LINES AND ANGLESBook:MTG IIT JEE FOUNDATIONChapter:LINES AND ANGLESExercise:NCERT SECTION( EXERCISE 6.1)
Explore 6 Videos
- LINES AND ANGLESBook:MTG IIT JEE FOUNDATIONChapter:LINES AND ANGLESExercise:NCERT SECTION( EXERCISE 6.2)
Explore 6 Videos
- LINES AND ANGLESBook:MTG IIT JEE FOUNDATIONChapter:LINES AND ANGLESExercise:OLYMPIAD/HOTS CORNER
Explore 20 Videos
- LINEAR EQUATIONS IN TWO VARIABLES Book:MTG IIT JEE FOUNDATIONChapter:LINEAR EQUATIONS IN TWO VARIABLES Exercise:OLYMPIAD/HOTS CORNER
Explore 5 Videos
- NUMBER SYSTEMSBook:MTG IIT JEE FOUNDATIONChapter:NUMBER SYSTEMSExercise:Olympiad/HOTS Corner
Explore 20 Videos
Similar Questions
In Figure, AE bisects ∠CAD and ∠B=∠C. Prove that AE∣∣BC
View SolutionIn the given figure, AB∣∣EC,AB=AC and AE bisects ∠DAC. Prove that:
View SolutionIn Figure, AC=AE, AB=AD and ∠BAD=∠EAC. Prove that BC=DE
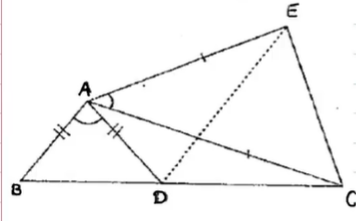
View SolutionIn the given figure, if x=y and AB=CB, then prove that AE=CD.
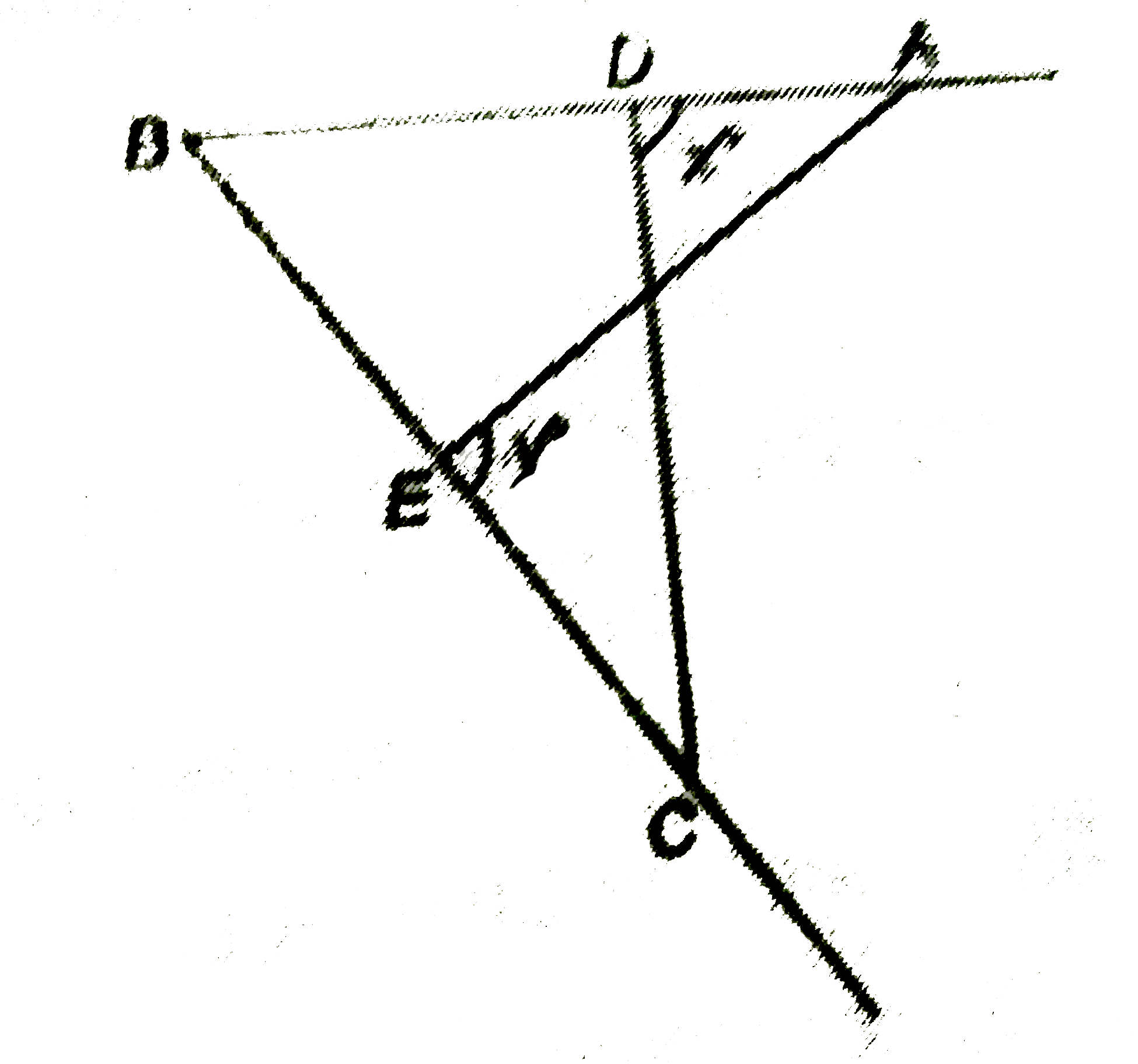
View SolutionIn the given figure, if x=y and AB=CB, then prove that AE=CD.

View SolutionIn Figure, it is given that AE=AD and BD=CE. Prove that AEB ≅ADC
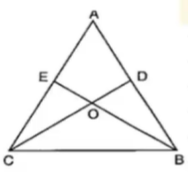
View SolutionIn figure, it is given that AE=AD and BD=CE. Prove that ΔAEB≅ΔADC.
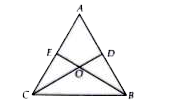
View SolutionIn the given figure, AB = AC and AD = AE. Prove that:
BD = CE
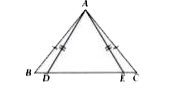
View SolutionIn the given figure, ΔABC is an isosceles triangle in which AB=AC and AE bisects ∠CAD. Prove that AE∣∣BC.
View SolutionIn the given figure, AE = DE and BC || AD. Prove that the points A,B,C and D are concyclic. Also, prove that the diagonals of the quadrilateral ABCD are equal.
View Solution
