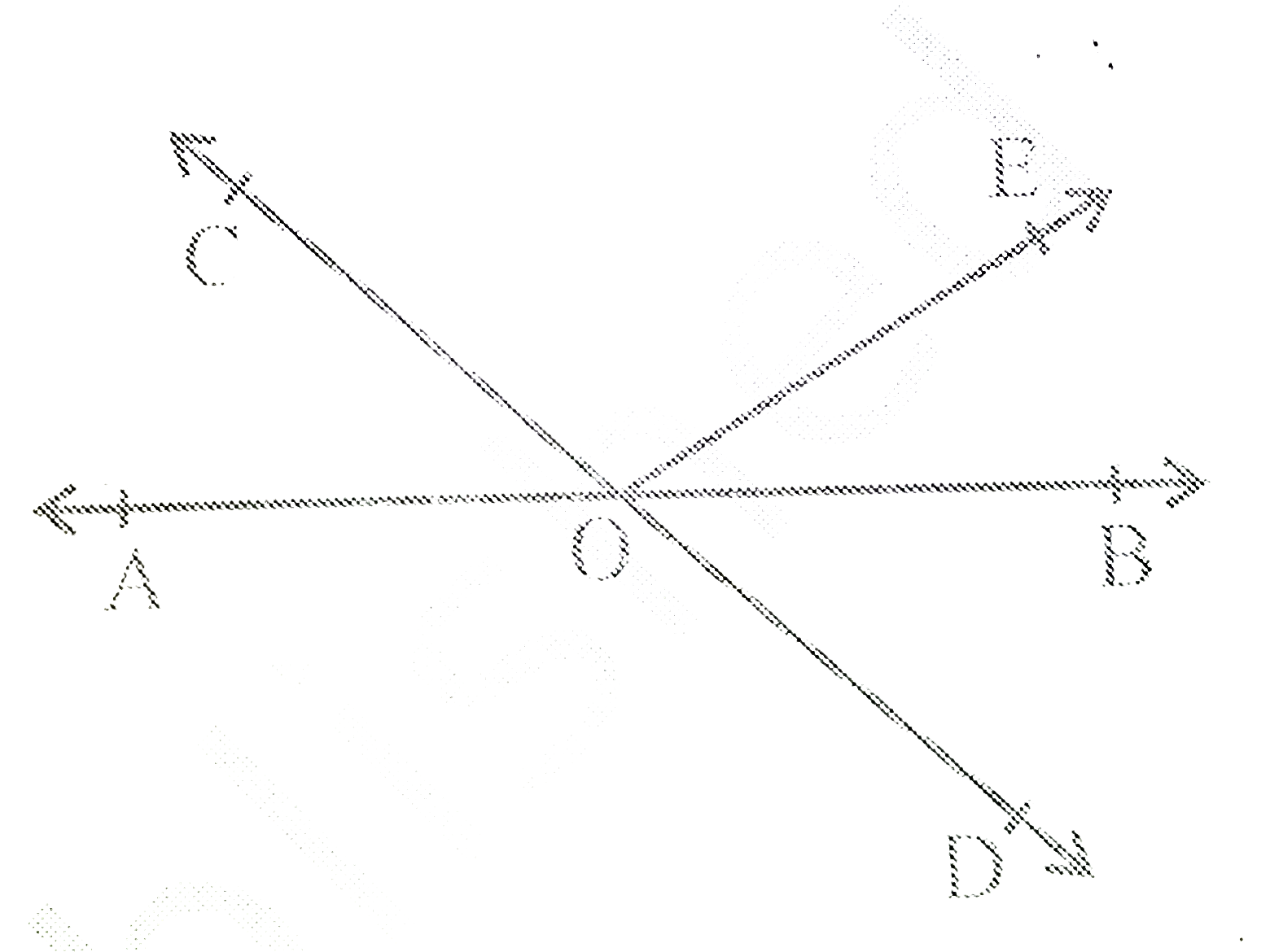In figure ray OE bisects ∠AOB and OF is the ray opposite OE. Show that ∠3=∠4
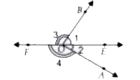
The correct Answer is:∠4
Topper's Solved these Questions
- LINES AND ANGLESBook:MTG IIT JEE FOUNDATIONChapter:LINES AND ANGLESExercise:NCERT SECTION( EXERCISE 6.1)
Explore 6 Videos
- LINES AND ANGLESBook:MTG IIT JEE FOUNDATIONChapter:LINES AND ANGLESExercise:NCERT SECTION( EXERCISE 6.2)
Explore 6 Videos
- LINES AND ANGLESBook:MTG IIT JEE FOUNDATIONChapter:LINES AND ANGLESExercise:OLYMPIAD/HOTS CORNER
Explore 20 Videos
- LINEAR EQUATIONS IN TWO VARIABLES Book:MTG IIT JEE FOUNDATIONChapter:LINEAR EQUATIONS IN TWO VARIABLES Exercise:OLYMPIAD/HOTS CORNER
Explore 5 Videos
- NUMBER SYSTEMSBook:MTG IIT JEE FOUNDATIONChapter:NUMBER SYSTEMSExercise:Olympiad/HOTS Corner
Explore 20 Videos
Similar Questions
Knowledge Check
In the given figure, rays OA, OB, OC and OD are such that ∠AOB = 50∘ , ∠BOC = 90∘, ∠COD=70∘ and ∠AOD=x∘. Then, the value of x is
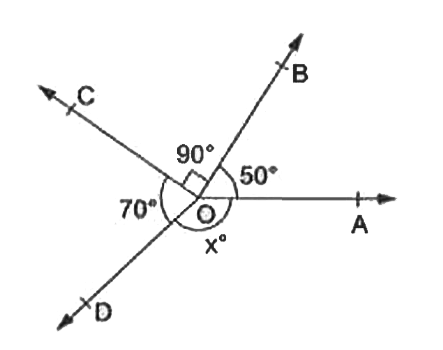 A50∘B70∘C150∘D90∘
A50∘B70∘C150∘D90∘
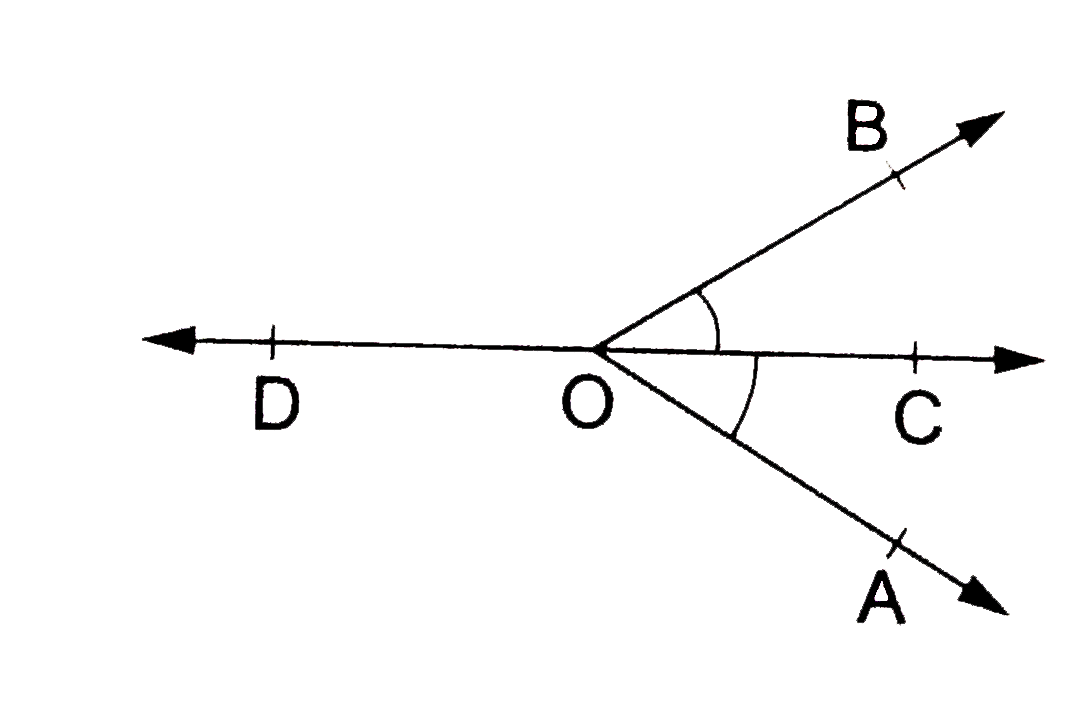
In the given figure, ray OC is the bisector of ∠AOB and OD is the ray opposite to OC. Show that ∠AOD=∠BOD.
View SolutionOA = OBand OD = OC. Show that (i)ΔAOD≅ΔBOC and (ii)AD ∣∣ BC
View SolutionIn the figure, OD is the bisector of ∠AOC, OE is the bisector of ∠BOC and OD ⊥ OE. Show that the points A, O and B are collinear.
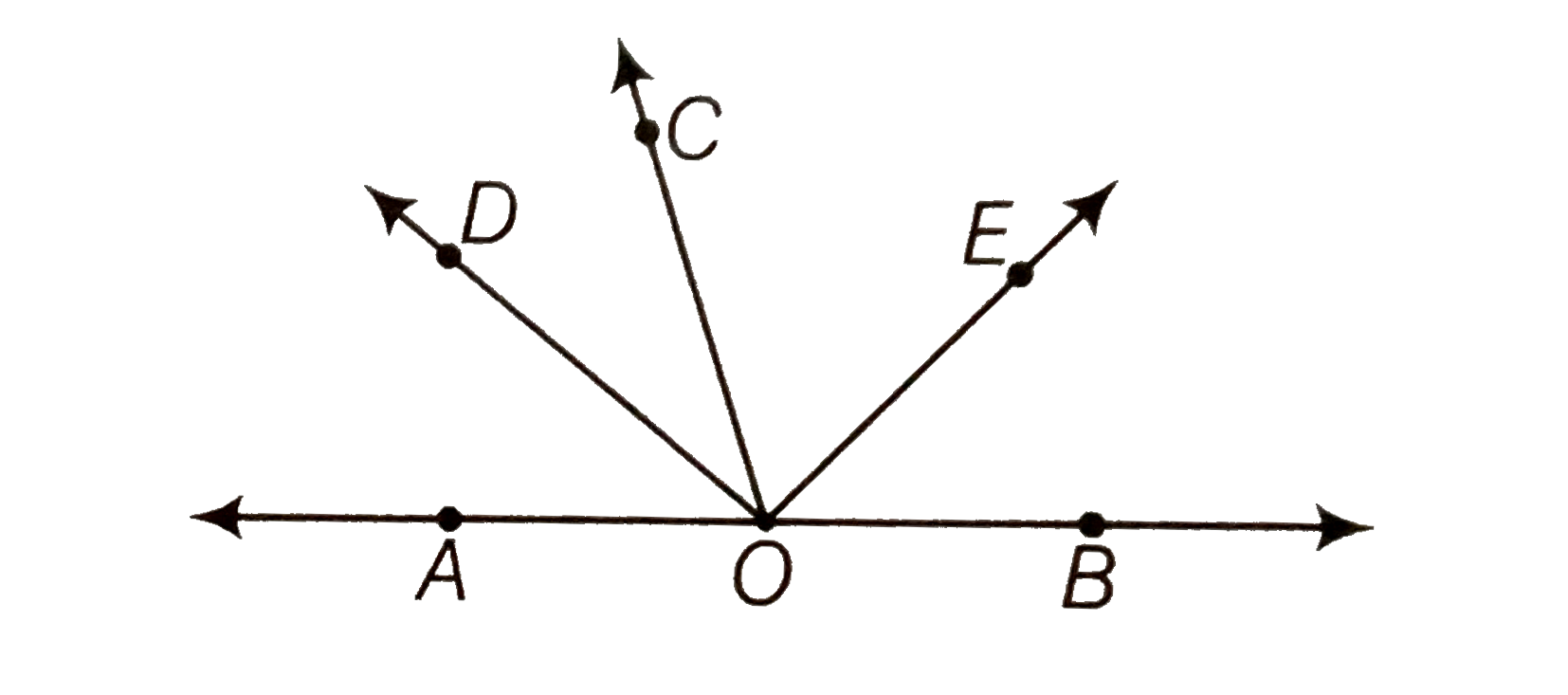
View SolutionIn the figure, OD is the bisector of ∠AOC, OE is the bisector of ∠BOC and OD ⊥ OE. Show that the points A, O and B are collinear.

View SolutionIn the figure, seg AB≅ seg AC, ray CE bisects ∠ACB , ray BD bisects ∠ABC. Prove that ray ED∣∣ side BC.
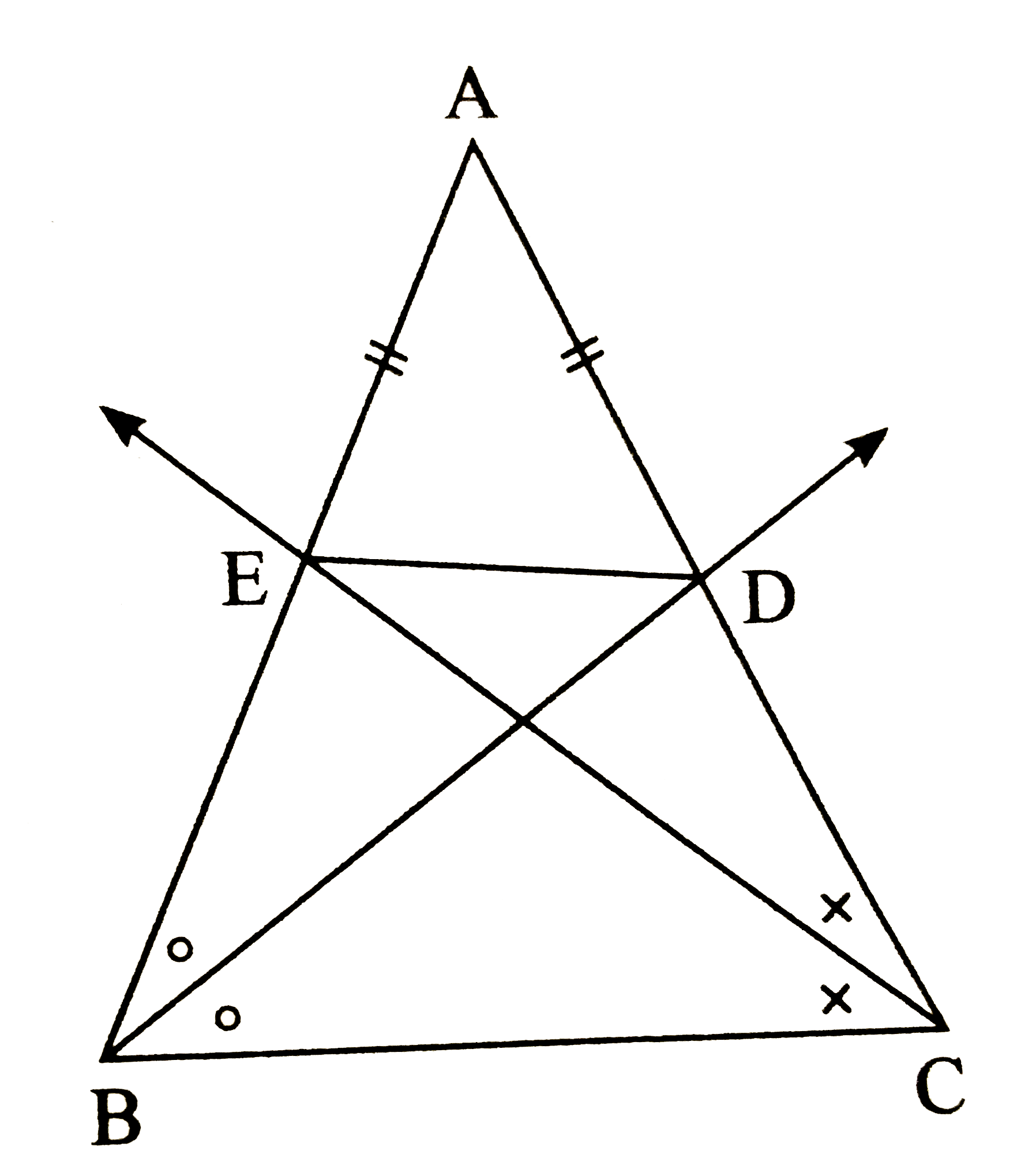
View SolutionIn ΔABC,ray BD bisects ∠ABC and ray CE bisects ∠ACB. If seg AB ≅seg AC, then prove that ED || BC.
View SolutionIn the figure, ray QS bisects ∠PQR. PQ=7, QR=5, SR=2.5 and PS=a then the value of a is ……….
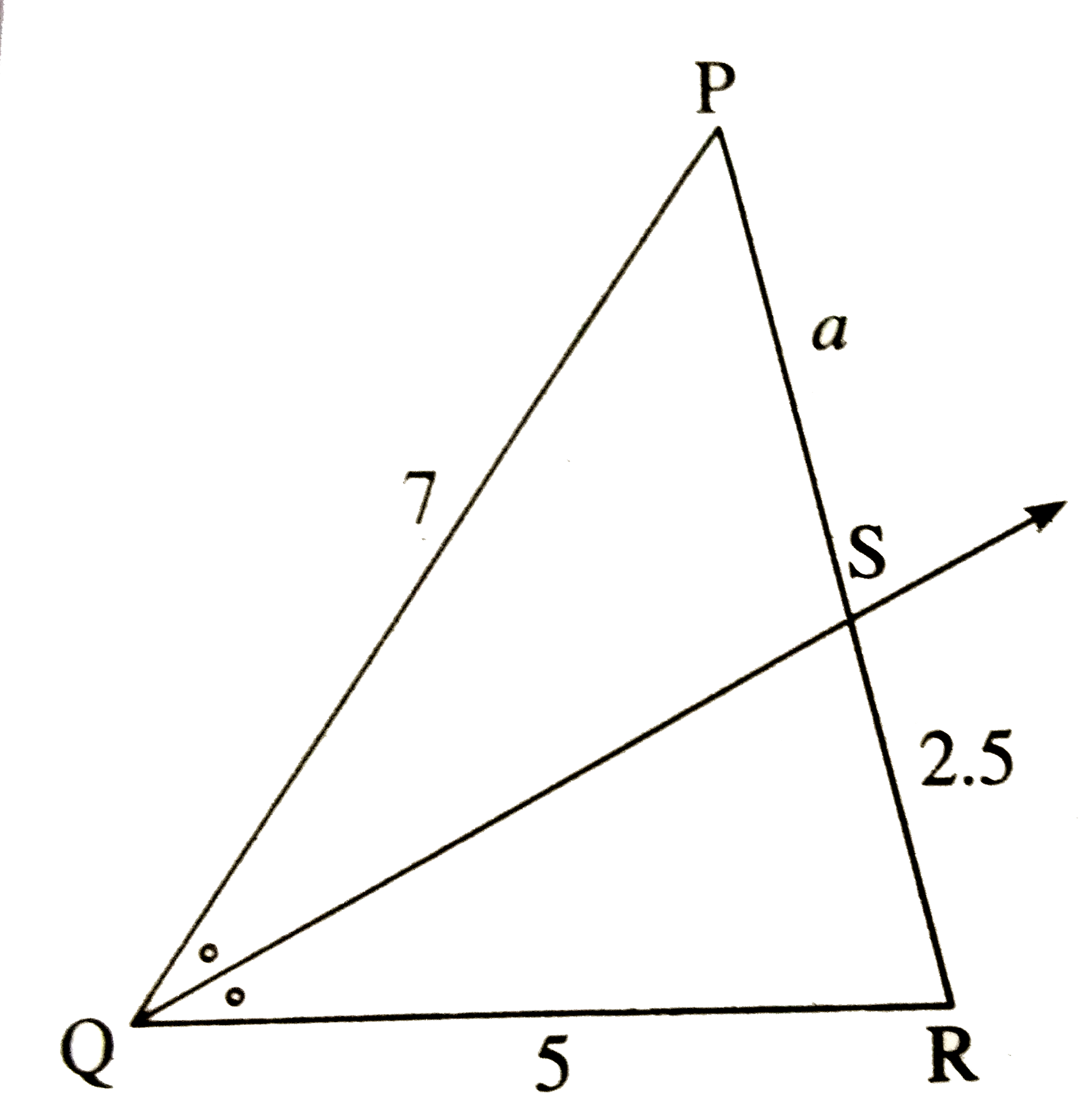
View Solution