If a chord is drawn through the point of a tangent to circle, then the angles which this chord makes with the given tangent are respectively equal to the angles formed in the corresponding alternate segment.
In the given fig, PQ is a tangent to the circle at A angleBAQ =50^@ and angleBAC =35^@," then "angleABC = (##MTG_FOU_COU_MAT_X_C10_E02_047_Q01.png" width="80%">
50∘
35∘
85∘
95∘
The correct Answer is:D
Topper's Solved these Questions
- CIRCLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CIRCLESEXERCISE - Exercise (Comprehension (Passage-Ii))
4 Videos
- CIRCLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CIRCLESEXERCISE - Exercise (Comprehension (Passage-III))
4 Videos
- CIRCLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CIRCLESEXERCISE - Exercise (Assertion & Reason)
6 Videos
- ARITHMETIC PROGRESSIONS BOOK - MTG IIT JEE FOUNDATIONCHAPTER - ARITHMETIC PROGRESSIONS EXERCISE - OLYMPIAD/HOTS CORNER
15 Videos
- CO-ORDINATE GEOMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Olympiad/HOTS Corner
40 Videos
Similar Questions
If a chord is drawn through the point of contact of a tangent to a circle; then the angle which this chord makes with the given tangent are equal respectively to the angles formed in the corresponding alternate segments.
View SolutionIn the given figure. A is the centre of the circle , ABCD is a parallelogram and CDE is a straight line.
Prove that :∠BCD=2∠ABE
(##SEL_RKB_ICSE_MAT_X_C17_E02_036_Q01.png" width="80%">
View Solution
Knowledge Check
In the given figure, chords AB and CD are equal. If ∠OBA=55∘ then ∠COD is :
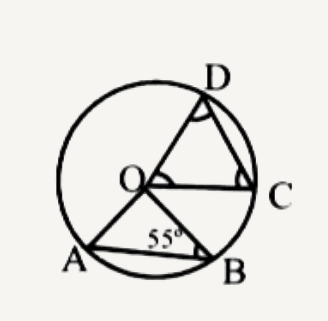 A65∘B55∘C70∘D50∘
A65∘B55∘C70∘D50∘In the figure , CP and CQ are tangent to a circle with centre O.ARB is another tangent touching the circle R . If CP =11 and BC = 7 cm then what is the length of BR?
(##CEN_JEE_MAT_X_C14_E01_043_Q01.png" width="80%">A2 cmB4 cmC6 cmD8 cmIn the given figure, AB is a chord of the circle with centre O and PQ is a tangent at point B of the circle. If ∠AOB=110∘, then ∠ ABQ is
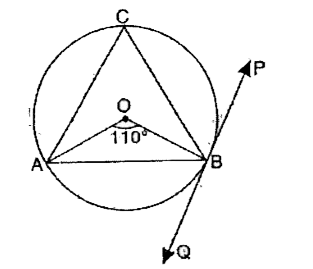 A45∘B70∘C55∘D35∘
A45∘B70∘C55∘D35∘
Calculate the angles x,y and z if
x3=y4=z5
(##SEL_RKB_ICSE_MAT_X_C17_E02_042_Q01.png" width="80%">
View SolutionIn the given figure ∠BAD=60∘,∠ABD=70∘∠BDC=45∘
(i) prove that AC is a diameter of the circle
(ii) Find ∠ACB
(##SEL_RKB_ICSE_MAT_X_C17_E02_002_Q01.png" width="80%">
View SolutionIn the given figure. AB is the diameter of the circle with centre O.
(##SELRKBICSEMATX_C17E04012_Q01.png width=80%><br>If angle ADC = 32 ^(@) , find angle BOC.
View SolutionIn the given figure, PQL and PRM are tangents to the circle with centre 'O' at the points Q and R, respectively and S is a point on the circle such that ∠SQL=50∘and∠SRM=60∘. Then ∠QSR is equal to
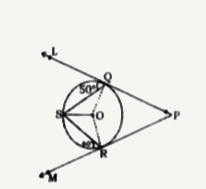
View SolutionIf the figure , CP and CQ are tangents from an external point C to a circle with centre O. AB is another tangent which touches the circle at R. If CP = 11 cm and BR = 4 cm , find the length of BC.
(##CEN_JEE_MAT_X_C14_E01_042_Q01.png" width="80%">
View Solution
