Assertion : If two tangents are drawn to a circle from an external point, then they subtend equal angles at the centre.
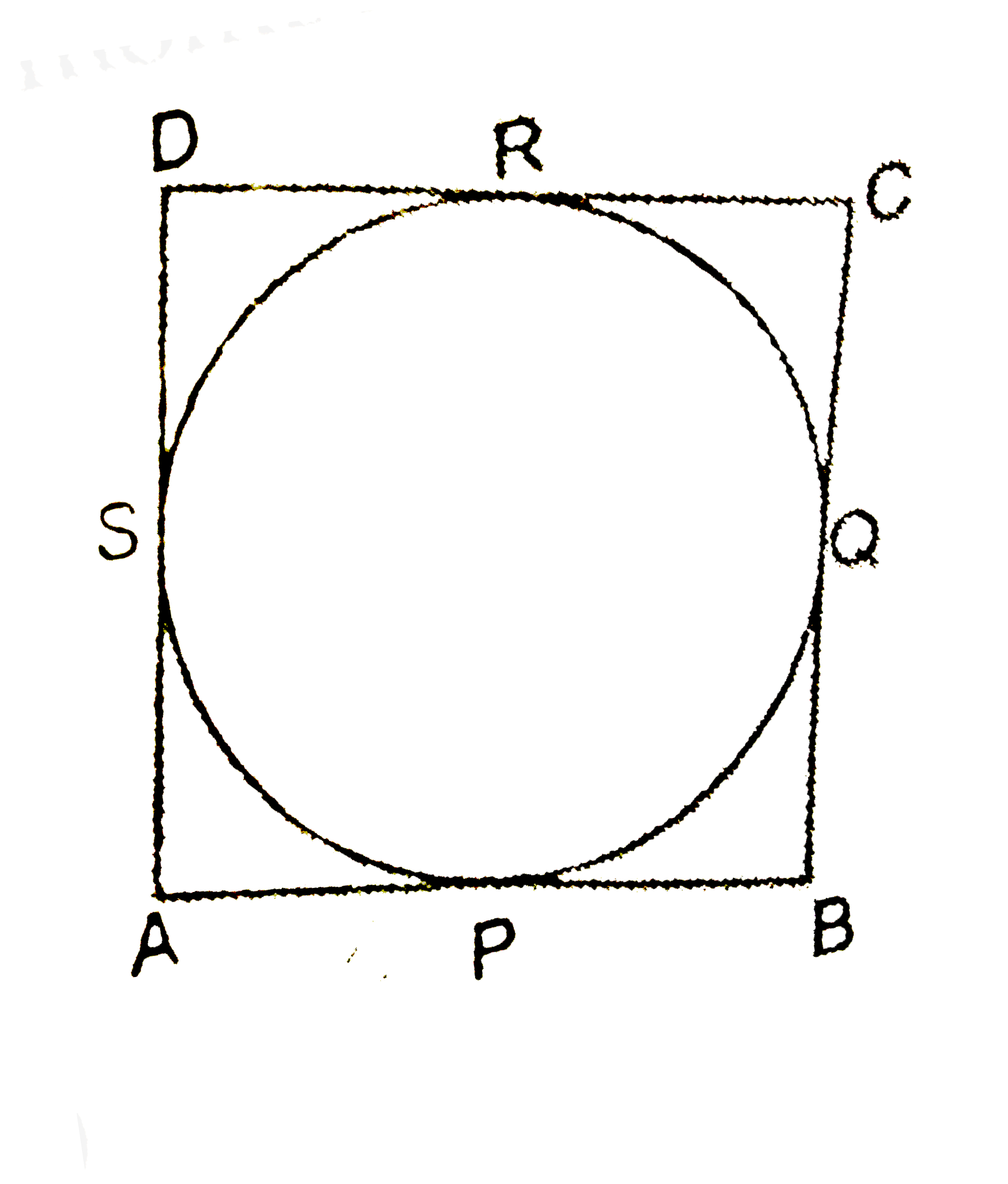
Reason : A parallelogram circumscribing a circle is a rhombus.
If both assertion and reason are true and reason is the correct explanation of assertion.
If both assertion and reason are true and reason is not the correct explanation of assertion.
If assertion is true but reason is false.
If assertion is false but reason is true.
The correct Answer is:B
Topper's Solved these Questions
- CIRCLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CIRCLESEXERCISE - Exercise (Comprehension (Passage-I))
4 Videos
- CIRCLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CIRCLESEXERCISE - Exercise (Comprehension (Passage-Ii))
4 Videos
- CIRCLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CIRCLESEXERCISE - Exercise (Match the following)
6 Videos
- ARITHMETIC PROGRESSIONS BOOK - MTG IIT JEE FOUNDATIONCHAPTER - ARITHMETIC PROGRESSIONS EXERCISE - OLYMPIAD/HOTS CORNER
15 Videos
- CO-ORDINATE GEOMETRYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Olympiad/HOTS Corner
40 Videos
Similar Questions
Knowledge Check
Assertion(A) If two tangents are drawn to a circle from an external point then they subtend equal angles at the centre.
Reason (R ) A parallelogram circumscribing a circle is a rhombusABoth Assertion (A) and Reason (R ) are true and Reason (R ) is a correct explanation of Asseration (A).BBoth Assertion (A) and Reason (R ) are true but Reason (R ) is not a correct explanation of Asseration (A).CAssertion(A) is true and Reason (R ) is false.DAssertion(A) is false and Reason (R ) is true.Choose the CORRECT option about the two tangents drawn to the circle from the external point?
1. The tangents subtend equal angles at the centre of the circle.
2. The tangents drawn from the external point to a circle are equal in length.
3. The tangents are equally inclined to the segment which joins the centre to that point.A1 and 2 onlyB2 and 3 onlyC1, 2 and 3D1 and 3 only
Prove that the parallelogram circumscribing a circle is a rhombus.
View SolutionProve that the parallelogram circumscribing a circle is a Rhombus.
View SolutionProve that the parallelogram circumscribing a circle is a Rhombus.
View SolutionTheorem:If two tangents are drawn to a circle from an external point ; then (i) they subtend equal angles at the centre. (ii) they are equally inclined to the line segments ; joining the centre to that point.
View SolutionIf two tangents are drawn to a circle from an external point ; then (i) they are equal (ii) they subtends equal angles at the centre. (iii) they are equally inclined to the segment ; joining the centre to that point .
View SolutionIf a parallelogram circumscribes a circle then prove that it must be a rhombus.
View Solution
