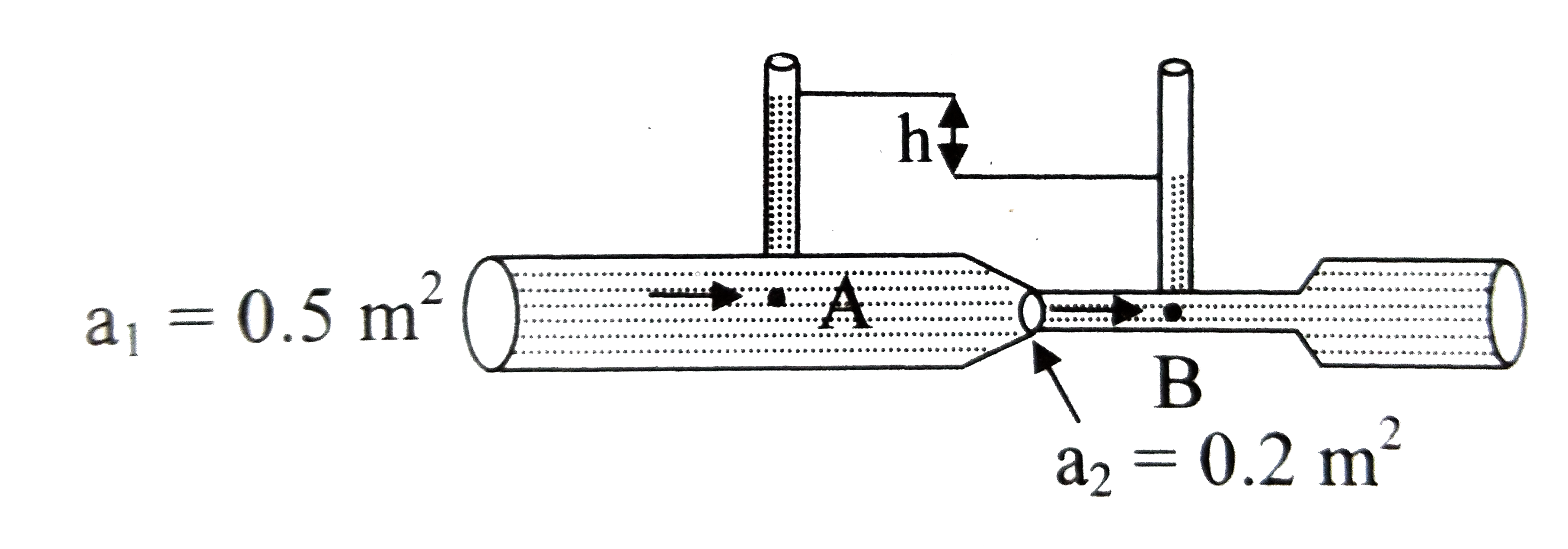
A liquid flows through a horizontal tube. The velocities of the liquid in the two sections, which have areas of cross section and are and respectively. The difference in the levels of the liquid in the two vertical tubes is . Then

The volume of the liquid flowing through the tube in unit time is
The energy per unit mass of the liquid is the same in both sections of the tube
The correct Answer is:A, C, D
According to equation of continuity -
correct
According to Bernoulli.s theorem
correct
According to Bernoulli.s theorem correct
Similar Questions
An incompressible, non-viscous liquis of density r flows through a horizontal tube as shown below. The flow condition is steady. Area of cross section at A and B are a1anda2 respectively. The height difference of liquid in two tubes inserted at A and B is h.
View SolutionA liquid flows through a horizontal tube. The velocities of the liquid in the two sections, which have areas of cross - section A_(1) and A_(2) are upsilon_(1)andupsilon_(2) respectively. The difference in the levels of the liquid in the two vertical tubes is h. a) The volume of the liquid flowing through the tube in unit time is A_(1)upsilon_(1) . b) upsilon_(2)-upsilon_(1)=sqrt(2gh) c) upsilon_(2)^(2)-upsilon_(1)^(2)=2gh d) The energy per unit mass of the liquid is the same in both sections of the tube.
View SolutionA liquid flows through a horizontal tube. The velocities of the liquid ind the two sections, which have areas of cross -section A_(1) and A_(2) are v_(1) and v_(2) respectively. The difference in the levels of the liquid in the two vertical tubes is h. a. The volume of the liquid flow through the tube in unit tims is A_(1)v_(1) b. v_(2)-v_(1)=sqrt(2gh) c. v_(2)^(2)-v_(1)^(2)=2gh d. The energy per unit mass of the liquid is the same in both sections of the tube.
View SolutionA liquid flows through a horizontal tube as shown in figure. The velocities of the liquid in the two sections, which have areas of cross-section A1 and A2 and v1 and v2 respectively. The differnece in the levels of the liquid in the two vertical tubes is h. then

View SolutionIf cross- sectional area of limb I is A1 and that of limb II is A2 then velocity of the liquid in the tube will be, (cross- sectional area of tube is very small)

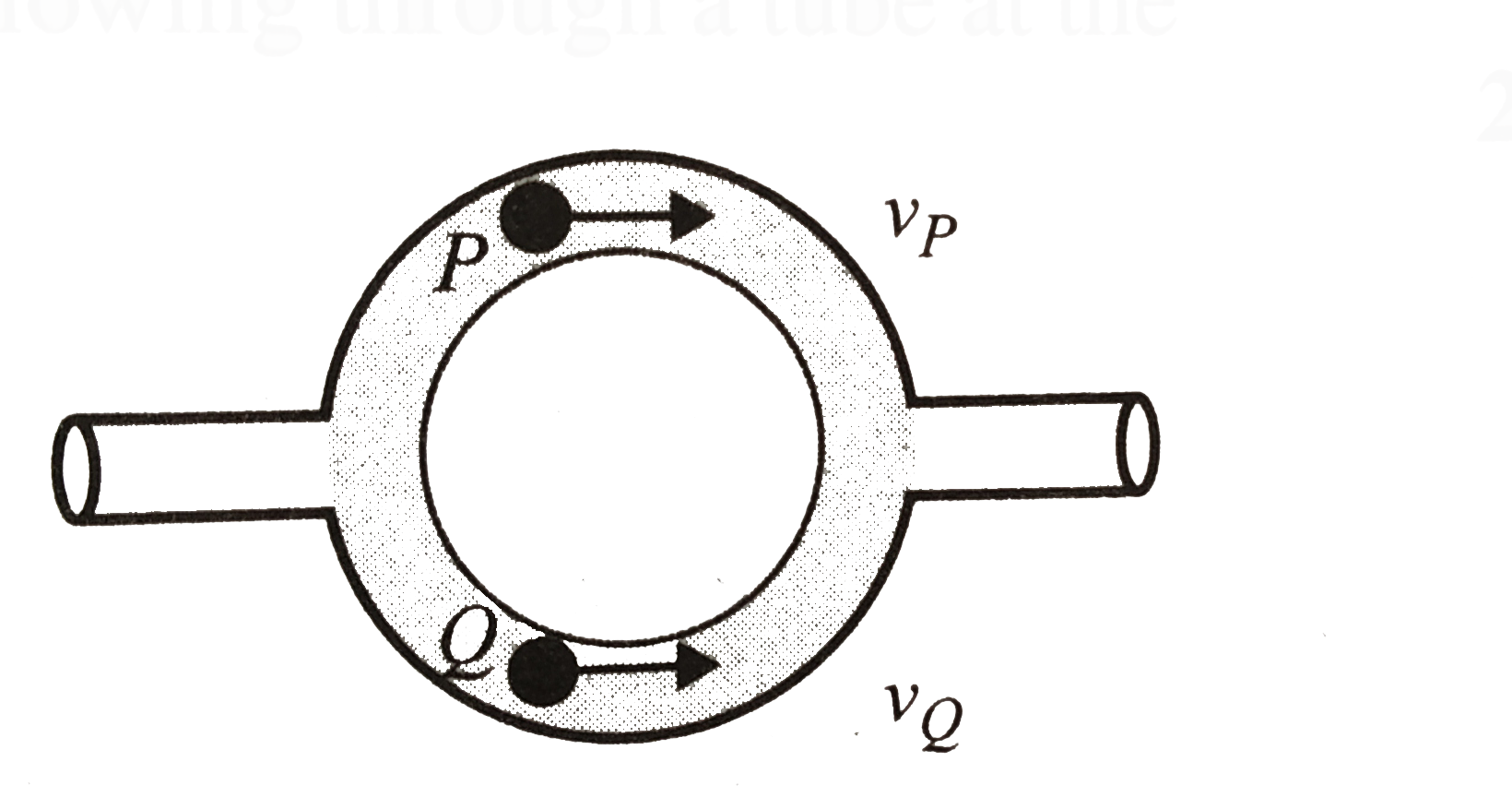
View SolutionFigure shows a liquid flowing through a tube at the rate of 0.1m3/s. The tube is branched into two semicircular tubes of cross-sectional area A/3 and 2A/3. The velocity of liquid at Q is (the cross section of the main tube is A=10−2m2 and vp=20m/s)
View SolutionA horizontal tube has different cross sections at points A and B . The areas of cross section are a_(1) and a_(2) respectively, and pressures at these points are p_(1)=rhogh_(1) and p_(2)=rhogh_(2) where rho is the density of liquid flowing in the tube and h_(1) and h_(2) are heights of liquid columns in vertical tubes connected at A and B . If h_(1)-h_(2)=h , then the flow rate of the liquid in the horizontal tube is
View SolutionA liquid flows through a horizontal tube. The velocities of the liquid in the two sections, which have areas of cross section A_(1) and A_(2) are v_(1) and v_(2) respectively. The difference in the levels of the liquid in the two vertical tubes is h . Then
View Solution
