Doubtnut Promotions Sticky

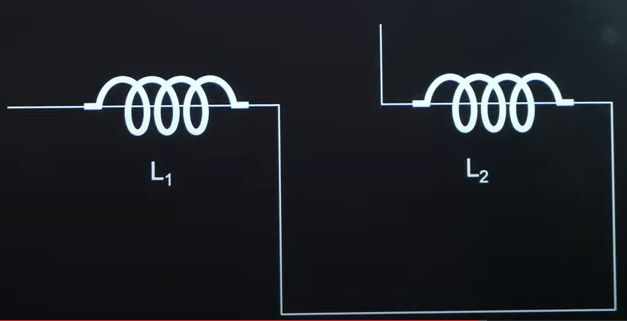
The equivalent relation is The equivalent relation is

A

1L1+1L2

B

L1+L2−2m

C

L1+L2+2M

D

L1−L2−M

Video Solution
Doubtnut Promotions Banner
|
Answer

Step by step video & image solution for The equivalent relation is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023
Class 12PHYSICSQUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    Statement-1: The relation R on the set N×N defined by (a, b) R (c, d) ⇔ a+d = b+c for all a, b, c, d ∈ N is an equivalence relation.
    Statement-2: The union of two equivalence relations is an equivalence relation.

    AStatement-1 is True, Statement-2 is true, Statement-2 is a correct explanation for statement-1.
    BStatement-1 is True, Statement-2 is true, Statement-2 is not a correct explanation for Statement-1.
    CStatement-1 is True, Statement-2 is False
    DStatement-1 is False, Statement-2 is True.
  • Question 2 - Select One

    Let P(x,y) be a point which is a constant distance from the origin. Then equivalence relation of (1,−1) is

    AA={(x,y)∣∣x2+y2=1∣∣}
    BA={(x,y)∣∣x2+y2=2∣∣}
    CA={(x,y)∣∣x2+y2=3∣∣}
    DA={(x,y)∣∣x2+y2=4∣∣}
  • Question 3 - Select One

    Which of the following are equivalence relations on A={4,6,8} ?

    A{(4,4),(6,6),(8,8),(4,6),(6,8)}
    B{(4,4),(6,6),(8,8),(4,6),(4,8)}
    C{(4,4),(6,6),(8,8),(4,8),(4,6)}
    D{(4,4),(6,6),(8,8),(4,8),(8,4)}