A square loop of side length l is placed at centre of another square loop of side length L. (L>>l). Mutual Inductance of loops is equal to
2√2μ0l2πl
μ0l2πl
μ0l22√2πl
√2μ0l2πl
To find the mutual inductance M of two square loops, we can use the formula for mutual inductance between two loops. The mutual inductance M can be calculated using the following steps:
Step 1: Understand the Configuration
We have two square loops: a larger loop with side length L and a smaller loop with side length l. The smaller loop is placed at the center of the larger loop.
Step 2: Calculate the Area of the Loops
The area A of a square loop is given by the formula:
A=side length2
Thus, the area of the larger loop AL is:
AL=L2
And the area of the smaller loop Al is:
Al=l2
Step 3: Use the Formula for Mutual Inductance
The mutual inductance M between two loops can be expressed as:
M=μ0⋅N1⋅N2⋅A2d
where:
- μ0 is the permeability of free space,
- N1 and N2 are the number of turns in the loops (for single loops, N1=N2=1),
- A2 is the area of the second loop (the smaller loop in this case),
- d is the distance between the loops.
However, since the smaller loop is centrally placed in the larger loop, we can simplify the calculation.
Step 4: Apply the Simplified Formula
For two square loops, the mutual inductance can be approximated as:
M=k⋅Al
where k is a constant that depends on the geometry of the loops. For square loops, k can be derived from the geometry and is often approximated as:
k≈μ0π⋅ln(Ll)
Step 5: Final Expression for Mutual Inductance
Thus, the mutual inductance M can be expressed as:
M=μ0π⋅ln(Ll)⋅l2
Conclusion
The mutual inductance M of the two square loops is given by:
M=μ0⋅l2π⋅ln(Ll)
We have two square loops: a larger loop with side length L and a smaller loop with side length l. The smaller loop is placed at the center of the larger loop.
The area A of a square loop is given by the formula:
A=side length2
Thus, the area of the larger loop AL is:
AL=L2
And the area of the smaller loop Al is:
Al=l2
The mutual inductance M between two loops can be expressed as:
M=μ0⋅N1⋅N2⋅A2d
where:
- μ0 is the permeability of free space,
- N1 and N2 are the number of turns in the loops (for single loops, N1=N2=1),
- A2 is the area of the second loop (the smaller loop in this case),
- d is the distance between the loops.
For two square loops, the mutual inductance can be approximated as:
M=k⋅Al
where k is a constant that depends on the geometry of the loops. For square loops, k can be derived from the geometry and is often approximated as:
k≈μ0π⋅ln(Ll)
Thus, the mutual inductance M can be expressed as:
M=μ0π⋅ln(Ll)⋅l2
The mutual inductance M of the two square loops is given by:
M=μ0⋅l2π⋅ln(Ll)
Topper's Solved these Questions
Similar Questions
A small square loop of side 'a' and one turn is placed inside a larger square loop of side b and one turn (b>>a). The two loops are coplanar with their centres coinciding. If a current I is passed in the square loop of side 'b', then the coefficient of mutual inductance between the two loops is :
View SolutionA small square loop of wire of side l is placed inside a large squre loop of wire of side L(>>l). The loops are coplanar and their centre coincide. What is the mutual inductance of the system ?
View Solution
Knowledge Check
A square loop of side a carries a current I. The magnetic field at the center of the loop is
A2μ0I√2πaBμ0I√2πaC4μ0I√2πaDμ0IπaA small square loop of wire of side l is placed inside a large square loop of wire of side L(L>>l). The loops are co-planer and their centres coincide. The mutual inductance of the system is proportional to
AlLBl2LCLlDL2lA square conducting loop of side L is situated in gravity free space. A small conducting circular loop of redius r(r<<L) is placed at the center of the square loop, with its plane perpendicular to the plane of the square loop. The mutual inductance of the two coils is
A2√2μ0ILr2B√2μ0I0Lr2C0DNone of these
A small square loop of wire of side l is placed inside a large square loop of wire of side (L>>l). The loops are coplanar and their centres coincide. If the mutual inductance of the system is p√qμ0πl2L, then find (p+q).
View SolutionA small square loop of wire of side l is placed inside a large square loop of wire of side L(L>>l) .The loops are coplanar and their centers coincide.The mutual inductance of the system is proportional to
View SolutionA small square loop of n1 turns and side l is placed at the centre of a large coplanar, circular loop of radius r( r gt gt l) and n2 tuns. The mutual inductacne of the combination is
View SolutionFind magnetic field at centre P if length of side of square loop is 20 cm.
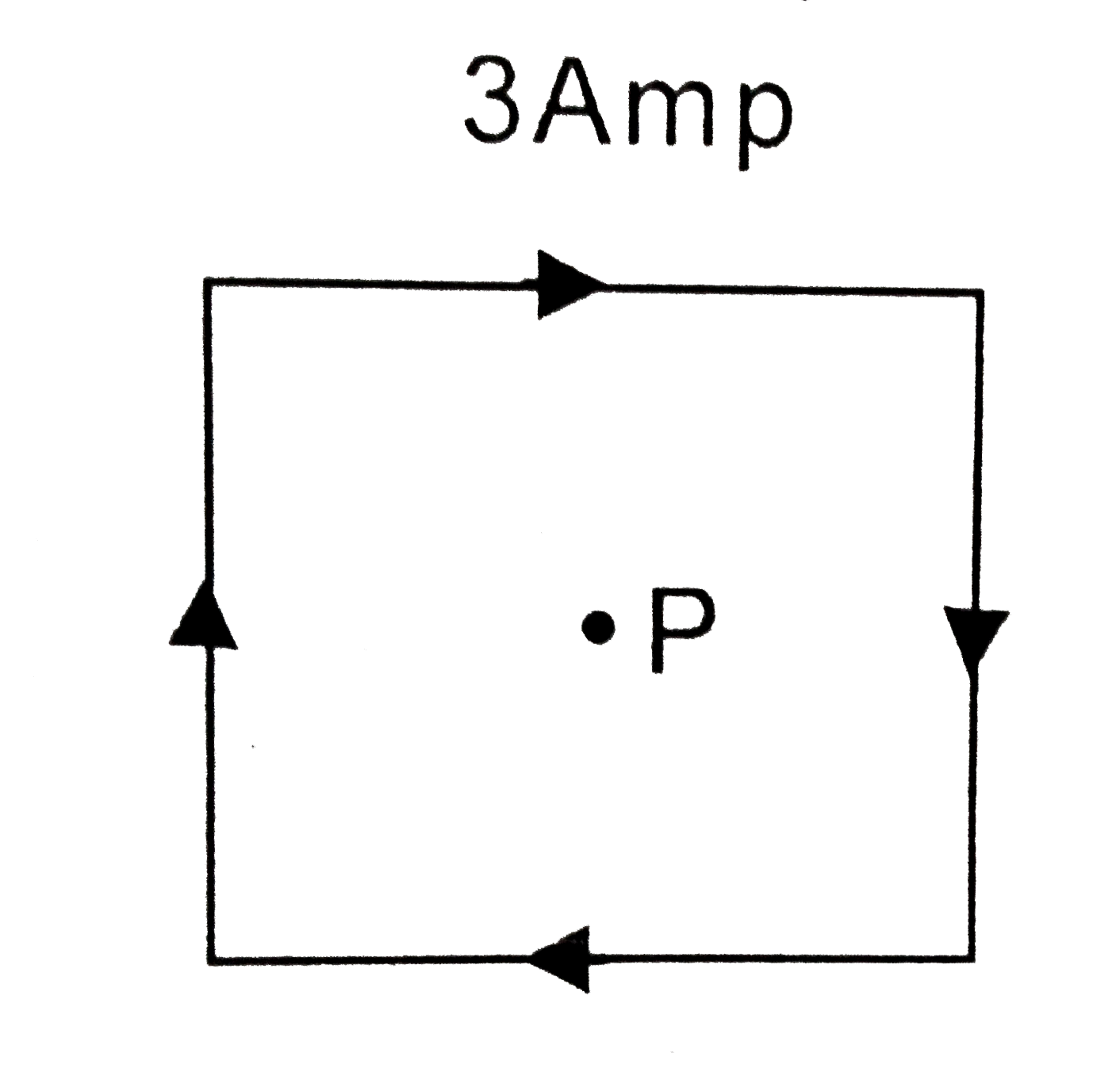
View SolutionA square loop of side L carries a current I. Another smaller square loop of side l(l<<L) carrying a current i is placed inside the bigger loop such that they are coplanar with their centre coinciding. If the currents in the loops are in the same direction, the magnitude of the torque on the smaller loop is
View Solution