Coordinate Of A Particle After A Given Time t|Velocity And Direction O

The velocity time graph of the motion of a car is given here.

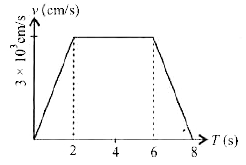AThe distance travelled by the car in the first six seconds is 150 m.
BThe deceleration of the car during the last two seconds are $- 15 \frac{m}{s^{2}}$ .
CThe distance travelled by the car in the first six second is 220 m.
DThe deceleration of the car during the last two second is $- 20 \frac{m}{s^{2}}$
A particle moves so that s=6+48t- $t^{3}$ . The direction of motion reverses after moving a distance of
A63
B104
C134
D288
Projectile motion is a combination of two one-dimensional motion: one in horizontal and other in vertical direction. Motion in 2D means in a plane. Necessary condition for 2D motion is that the velocity vector is coplanar to the acceleration vector. In case of projectile motion, the angle between velocity and acceleration will be $0^{\circ} < θ < 180^{\circ}$ . During the projectile motion, the horizontal component of velocity ramains unchanged but the vertical component of velocity is time dependent. Now answer the following questions:
A particle is projected from the origin in the x-y plane. The acceleration of particle in negative y-direction is $α$ . If equation of path of the particle is $y = a x - b x^{2}$ , then initial velocity of the particle is
A $\sqrt{\frac{α}{2 b}}$
B $\sqrt{\frac{α (1 + a^{2})}{2 b}}$
C $\sqrt{\frac{α}{a^{2}}}$
D $\sqrt{\frac{α b}{a^{2}}}$

Coordinate Of A Particle After A Given Time t|Velocity And Direction Of Motion After A Given Time|Velocity And Direction Of Motion At Given Height|Exercise Questions|Equation Of Trajectory