Let $l_{1}$ and $l_{2}$ be the lines ${\to r}_{1} = γ (ˆ i + ˆ j + ˆ k)$ and ${\to r}_{2} = (ˆ j - ˆ k) + μ (ˆ i + ˆ k)$ , respectively. Let X be the set of all the planes H that contain the line $l_{1}$ .For a plan H ,let d (H) denote the smallest possible distance between the points of $l_{2}$ and H . Let $H_{0}$ be a plane in X for which $d (H_{0})$ is the maximum value of d H( ) as H varies over all planes in X .

Match each entry in List-I to the correct entries in List-II.

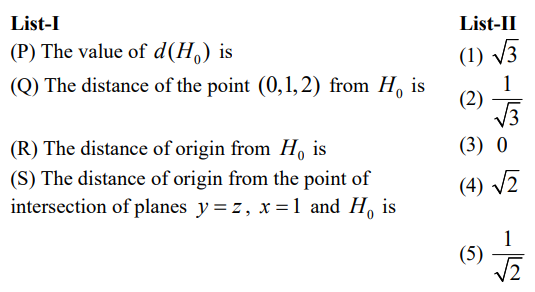
The correct option is:

$(P) \to (2) (Q) \to (4) (R) \to (5) (S) \to (1)$

$(P) \to (5) (Q) \to (4) (R) \to (3) (S) \to (1)$

$(P) \to (2) (Q) \to (1) (R) \to (3) (S) \to (2)$

$(P) \to (5) (Q) \to (1) (R) \to (4) (S) \to (2)$

Video Solution

Answer

Step by step video solution for Let l_1 and l_2 be the lines vec r_1= gamma (hati+ hatj +hat k ) and vecr_2 = (hatj -hat k) +mu(hati+hat k ), respectively. Let X be the set of all the planes H that contain the line l_1 .For a plan H ,let d (H) denote the smallest possible distance between the points of l_2 and H . Let H_0 be a plane in X for which d(H_0) is the maximum value of d H( ) as H varies over all planes in X . Match each entry in List-I to the correct entries in List-II. The correct option is: by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS JEE ADVANCED 2023

Topper's Solved these Questions

Knowledge Check

Let $L_{1}$ be the line ${\to r}_{1} = 2 ˆ i + ˆ j - ˆ k + λ (ˆ i + 2 ˆ k)$ and let $L_{2}$ be the line ${\to r}_{2} = 3 ˆ i + ˆ j - ˆ k + μ (ˆ i + ˆ j + ˆ k)$ . Let $π$ be the plane which contains the line $L_{1}$ and is parallel to $L_{2}$ . The distance of the plane $π$ from the origin is
A $\sqrt{2 / 7}$
B $1 / 7$
C $\sqrt{6}$
Dnone
If $\to a = ˆ i + 2 ˆ j + 2 ˆ k and \to b = 3 ˆ i + 6 ˆ j + 2 ˆ k$ then the vector in the direction of $\to a$ and having mgnitude as $∣ ∣ \to b ∣ ∣$ is
A $7 (ˆ i + 2 ˆ j + 2 ˆ k)$
B $\frac{7}{9} (ˆ i + 2 ˆ j + 2 ˆ k)$
C $\frac{7}{3} (ˆ i + 2 ˆ j + 2 ˆ k)$
Dnone of these
Let $L_{1}$ be the line $r_{1} = 2 ˆ i + ˆ j - ˆ k + λ (ˆ i + 2 ˆ k)$ and let $L_{2}$ be the another line $r_{2} = 3 ˆ i + ˆ j + μ (ˆ i + ˆ j - ˆ k)$ . Let $ϕ$ be the plane which contains the line $L_{1}$ and is parallel to the $L_{2}$ . The distance of the plane $ϕ$ from the origin is
A $\sqrt{\frac{2}{7}}$
B $\frac{1}{7}$
C $\sqrt{6}$
DNone of these

JEE ADVANCED PREVIOUS YEAR-JEE ADVANCED 2023-Question

Home
Ask Doubt
Profile

Topper's Solved these Questions

Similar Questions

Knowledge Check

Let L1 be the line →r1=2ˆi+ˆj−ˆk+λ(ˆi+2ˆk) and let L2 be the line →r2=3ˆi+ˆj−ˆk+μ(ˆi+ˆj+ˆk). Let π be the plane which contains the line L1 and is parallel to L2. The distance of the plane π from the origin is

If →a=ˆi+2ˆj+2ˆkand→b=3ˆi+6ˆj+2ˆk then the vector in the direction of →a and having mgnitude as ∣∣→b∣∣ is

Let L1 be the line r1=2ˆi+ˆj−ˆk+λ(ˆi+2ˆk) and let L2 be the another line r2=3ˆi+ˆj+μ(ˆi+ˆj−ˆk). Let ϕ be the plane which contains the line L1 and is parallel to the L2. The distance of the planeϕ from the origin is

JEE ADVANCED PREVIOUS YEAR-JEE ADVANCED 2023-Question

If $\to a = ˆ i + 2 ˆ j + 2 ˆ k and \to b = 3 ˆ i + 6 ˆ j + 2 ˆ k$ then the vector in the direction of $\to a$ and having mgnitude as $∣ ∣ \to b ∣ ∣$ is