Let z be a complex number satisfying |z|3+2z2+4¯z−8=0 , where ¯z denotes the complex conjugate of z . Let the imaginary part of z be nonzero
Match each entry in List-I to the correct entries in List-II.
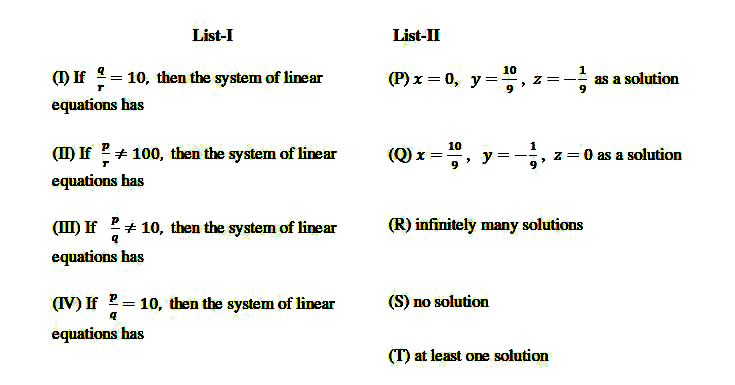
The correct option is:
(P)→(1)(Q)→(4)(R)→(5)(S)→(4)
(P)→(2)(Q)→(1)(R)→(3)(S)→(5)
(P)→(2)(Q)→(4)(R)→(5)(S)→(1)
(P)→(2)(Q)→(3)(R)→(5)(S)→(4)
Step by step video solution for Let z be a complex number satisfying |z|^3 +2z^2 +4barz -8 =0 , where barz denotes the complex conjugate of z . Let the imaginary part of z be nonzero Match each entry in List-I to the correct entries in List-II. The correct option is: by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
Let z be a complex number satisfying |z+16|=4|z+1|. Then
A|z|=4B|z|=5C|z|=6D3<|z|<68z is a complex number satisfying z4+z3+2z2+z+1=0, then |z| is equal to
A12B34C1Dnone of theseIf z is a complex number satisfying |z|2+2(z+2)+3i(z−¯z)+4=0, then complex number z+3+2i lies on
Acircle witih center 1-5i and radius 4Bcircle with center 1+5i and radius 4Ccircle with center 1+5i and radius 3Dcircle with center 1-5i and radius 3
Let z be a complex number satisfying 2z+|z|=2+6i .Then the imaginary part of z is
View SolutionThe nonzero complex number Z satisfying Z=iZ2 is
View SolutionLet z be a complex number satisfying |z−5i|≤1 such that amp(z) is minimum, then z is equal to
View SolutionLet z be a complex number satisfying |z|=3|z−1|. Then prove that ∣∣∣z−98∣∣∣=38
View SolutionLet α,βandγ be real numbers. Consider the following system of linear equations
x+2y+z=7
x+αz=11
2x−3y+βz=γ
Match each entry in List-I to the correct entries in List-II.
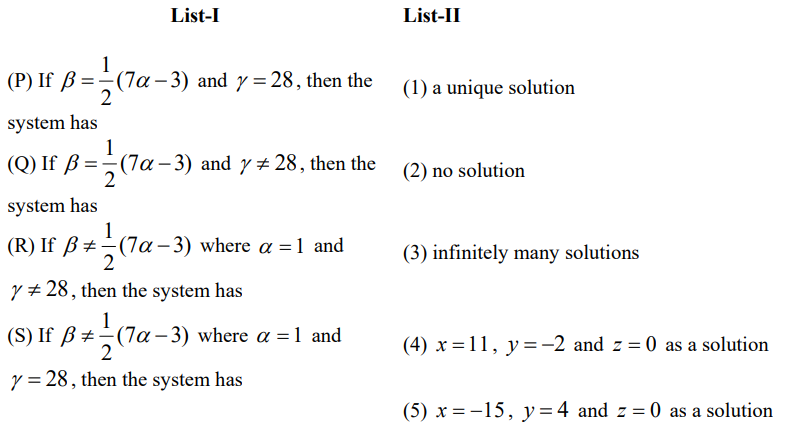
The correct option is:
View Solution
