Cube
Introduction
A particular 3-D figure with six identical square faces joined along their corner points is called the cube. Such identical square faces of each are joined together at three edges at every corner to form a vertex. This sort of plain cube can also be called as a regular hexahedron. And also it is one of the five regular polyhedrons. And these are also sometimes considered as Platonic solids. A particular region of space that is created by using a total number of six identical square faces which are joined along the edges is called a cube.
In order to form a vertex, three singular edges join at each corner. Cube is also one of the five different kinds of regular polyhedrons. And these are also in many kinds referred to as the Platonic solids. A considerable amount of space formed by six different square faces that are joined along the edges is formed as a cube. And for this cube, three edges are joined up together at every corner to form as a vertex.
Parts of a Cube
First-term that is referred to as a part of a cube is the face. The face of a cube is also called as facets or sides. A particular cube will be having a total of six faces, and each and every face of such cube is square-shaped. Among these six faces of the cube, each and every face will have four equal sides. And each of these six faces of the cube will be having a total of all four interior angles. And all these four angles are right angles. The first part of a cube that needs to be discussed is the face of the cube. The face of the cube is also called as the facet or side of the cube.
The second term that is referred to as another part of the cube is the edge. Whenever any two edges meet a line segment is formed. For each and every cube, there will be a total of 12 edges. For each and every edge meeting in a cube, there will be a line segment that is formed. Another part of the cube that needs to be discussed is the edge of the cube. For every two edges meeting at the corner of a cube, a line segment is formed. For any cube-shaped object or a particular cube, there will be a total number of 12 edges.
Vertex
A vertex is another part of the edge that needs to be discussed. When the three edges meet for a cube, a vertex is formed. When for any solid cube, a point of intersection is formed with the joining of three edges, it is called a vertex. For any cube or for that matter for any sort of cube-shaped object, there will be a total of 8 vertices.
Related Videos:
- Surface area of cube
- Lateral surface area of cube
- Cuboid
- Volume of a cuboid
- Surface area of a cuboid
- Lateral surface area of a cuboid
Face Diagonals
The line segments of a cube that are making the joining of the opposite corners for every face of the cube are known as face diagonals. For every face of the cube out of 12 faces in a cube, there will be 2 face diagonals for every face of the cube. And for that matter, there will be a total of 12 face diagonals in every cube, making it a complete cube-shaped object. For each face of the cube, there will be a total of 12 face diagonals in the cube. The formula for calculating the length of a face diagonal is finding out by using the following formula:
Face Diagonal = Sq Root of (2s square)
Space Diagonals
The line segments that are formed through the linking of the opposite corners of a cube and that which cut through the interiors of the cube are called as the space diagonals. For every volume of cube or for that matter for any cube-shaped object also there will be a total of 4 space diagonals. The lines that are formed by linking the opposite corners of a cube that are went cutting through the interiors of the cube are called as the line segments that are also called as the space diagonals. The length of the space diagonal is calculated by using the following formula:
Length of space diagonal = Square root of (3s square)
Volume of Cube
Volume of a cube is the space that contained in a cube. In order to understand the formula of the volume of cube formula, we need to concentrate on it in the best way. Let’s for example, the volume calculates the object if we need to immerse a cubical shape object in the water. Here, the formula of the volume is:
Volume of cube = a x a x a = a3
Important Chapters:
Similar Questions
Knowledge Check
- Question 1 - Select One
Following frightening diagrams show identical cubes such that edge of cube 2 lies exactly in the middle of one of the faces of Cube 1 and Cube 4 has a corner at the body centre of the cube 3 . Find the contribution (in fraction) of the spheres shown to each of the cube 1 ,2 , 3 and 4 respectively is
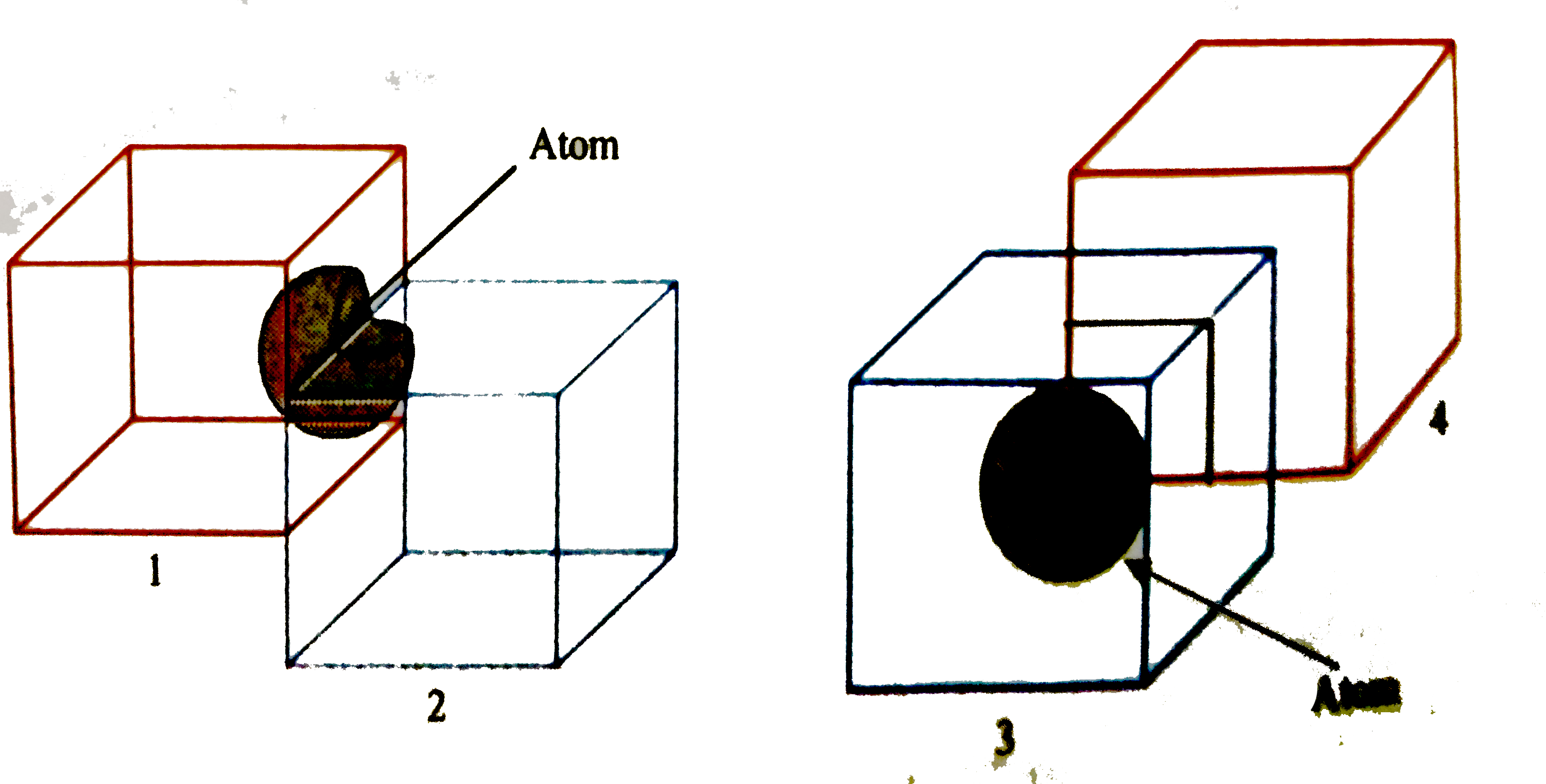 A12,14,1,18B12,14,18,1C18,14,1,12D18,14,12,1
A12,14,1,18B12,14,18,1C18,14,1,12D18,14,12,1 - Question 2 - Select One
Statements :
All spheres are cones
Some cubes are cones
All circles are cubes
Conclusions:
(i) No cube is sphere
(ii) All spheres may be cubes
(iii) Some cones are not circles
(iv) At least some cubes are circles
(v) Some cones which are cubes may be circlesA(i) & (ii) does not followB(i) & (iii) does not followC(ii)& (v) does not followD(iii) & (iv) does not follow


