Consider the inequation $9^{x} - a 3^{x} - a + 3 \leq 0$ , where a is real parameter.
The given inequality has at least one real solutions for $a \in$ .

A

$(- \infty, - 2)$

B

$[3, \infty)$

C

$(2, \infty)$

D

$[- 2, \infty)$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Given that $9^{x} - a 3^{x} - a + 3 \leq 0$
Let $t = 3^{x}$ . Then,
$t^{2} - a t - a + 3 \leq 0$
or $t^{3} + 3 \leq a (t + 1) (1)$
where $t \in R^{+}, \forall x \in R$
Let $f_{1} (t) = t^{2} + 3$ and
$f_{2} (t) = a (t + 1)$ .
For at least one posititve solution, $t \in (1, \infty)$ . That means graphs of $f_{1} (t) = t^{2} + 3 and f_{2} (t) = a (t + 1)$ should meet at least once is $t \in (1, \infty)$ . If $a = 2$ , both the curves touch each other at $(1, 4)$ .
Hence, the required range is $a \in (2, \infty)$

Updated on:21/07/2023

|

Class 12 MATHS QUADRATIC EQUATIONS

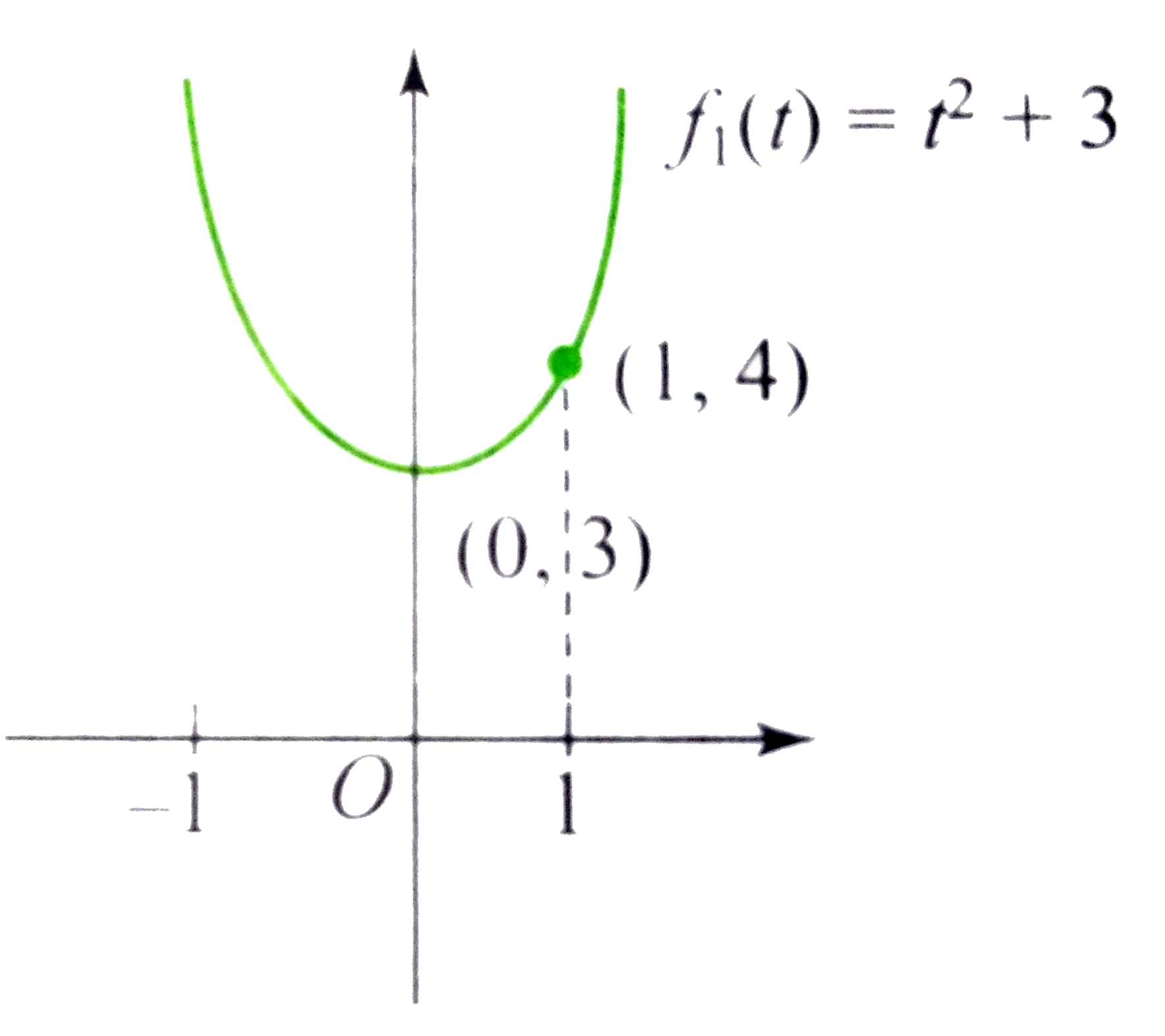
Topper's Solved these Questions

Similar Questions

Knowledge Check

Consider the inequation $9^{x} - a 3^{x} - a + 3 \leq 0$ , where a is real parameter.
The given inequality has at least one negative soluiton for $a \in$
A $(- \infty, 2)$
B $(3, \infty)$
C $(- 2, \infty)$
D $(2, 3)$
Consider the inequation $x^{2} + x + a - 9 < 0$
The values of the real parameter a so that the given inequations has at least one negative solution.
A $(- \infty, 9)$
B $(\frac{37}{4}, \infty)$
C $(- \infty, \frac{37}{4})$
Dnone of these
Consider the inequation $x^{2} + x + a - 9 < 0$
The values of the real parameter a so that the given inequaiton has at least one positive solution:
A $(- \infty, 37 / 4)$
B $(- \infty, \infty)$
C $(3, \infty)$
D $(- \infty, 9)$

CENGAGE-THEORY OF EQUATIONS-Exercise (Comprehension)

Home
Ask Doubt
Profile