If (x+2) is a common factor of (px2+qx+r) and (qx2+px+r) then a) p=q or p+q+r=0 b)p=r or p+q+r=0 c) q=r or p+q+r=0 d)p=q=−12r
A
|d|≤|D|
B
|d|≥|D|
C
|d|=|D|
D
None of these
Video Solution
Text Solution
The correct Answer is:1
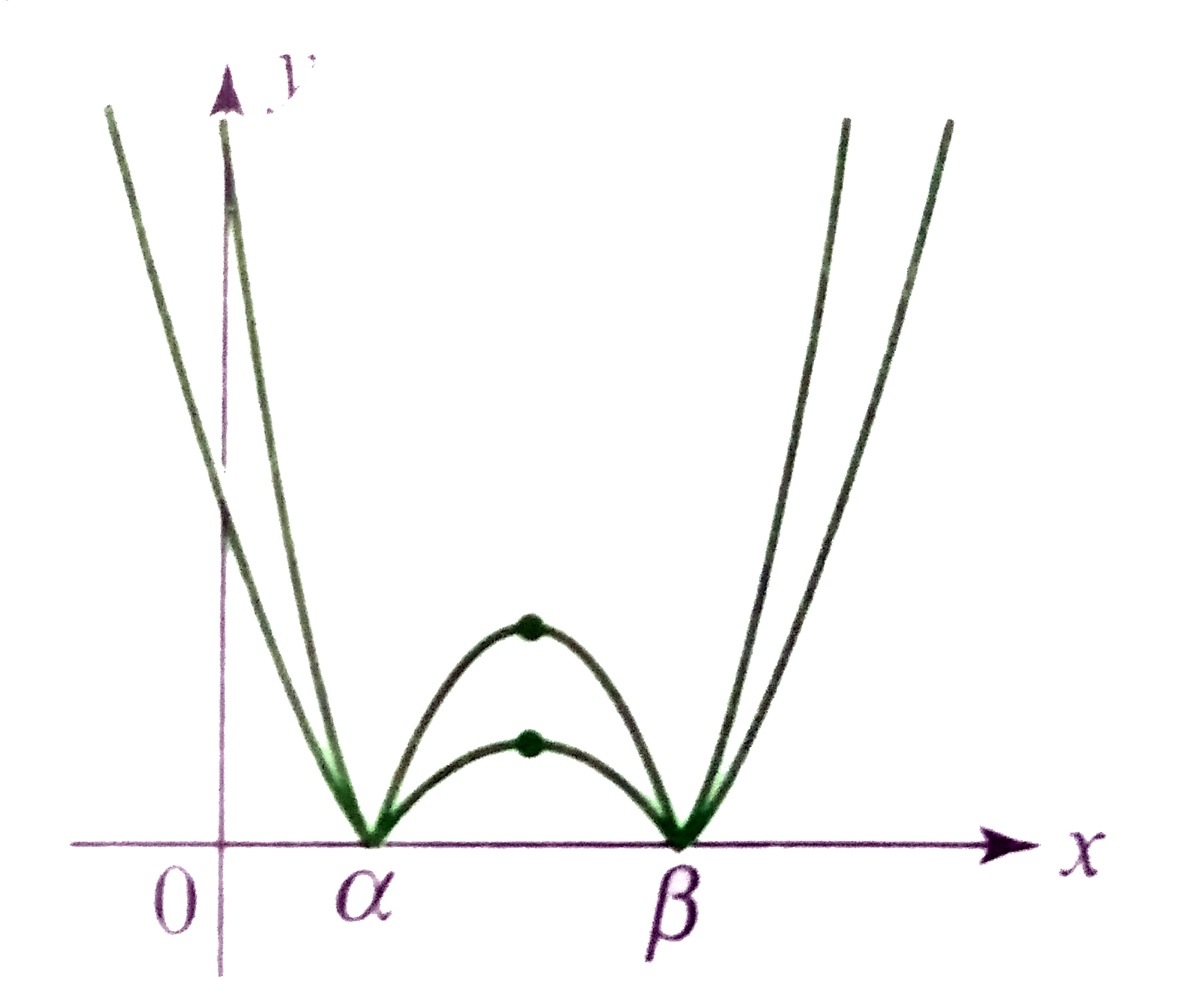
∣∣px2+qx+r∣∣≤∣∣Px2+Qx+R∣∣∀x∈R (1)
Form the graph we can see that this is possible only when both equations have same roots.
Thus, α and β are roots of Px2+Qx+R=0 and also of
px2+qx+r=0
So, from (1),
|p||x−α||x−β|≤|P||x−α||x−β|
⇒|p|≤|p|
Also ∣∣∣4pr−q24p∣∣∣≤∣∣∣4PR−Q24p∣∣∣
⇒|d|≤∣∣∣pP∣∣∣|D|
⇒|d|≤|D| (∵|p|≤|P|)
|
Topper's Solved these Questions
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Matrix)
6 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Numerical)
43 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Multiple)
38 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - JEE Advanced Previous Year
4 Videos
- THREE DIMENSIONAL GEOMETRYBOOK - CENGAGECHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - Question Bank
20 Videos
Similar Questions
Knowledge Check
If 3 - 4i is a root of x2−px+q=0 where p, q ∈R. then value 2p−qp+q is
A−12/31B−13/31C−15/31Dnone of thesepq−qr=(p+q)r−q, where 1<q<r<p<10 then the value of p+q+r is :
A31B21C15D12
Add p(p−q),q(q−r) and r(r−p)
View SolutionIf the equations px2+2qx+r=0 and px2+2rx+q=0 have a common root then p+q+4r=
View SolutionIf r is the geometric mean of p and q, then the line px + qy + r = 0
View SolutionIf the roots of x3+px2+qx+r=0 are in GP find the relation between p,qandr
View SolutionThe points (p,q +r) , (q, r+p) and (r, q+p) are _______.
View SolutionIf px3+qx2+rx+s=0 is a R.E .of second type then
p=s,q=r
p=-s,q=-r
p=s,q=-r
p=-s ,q=r
View Solution
