A solid sphere is projected along an inclined plane according to diagram. If coefficient of friction is μ=0.5 and radius R, then find
(a) Time when linear velocity becomes zero
(b) Angular velocity at that instant
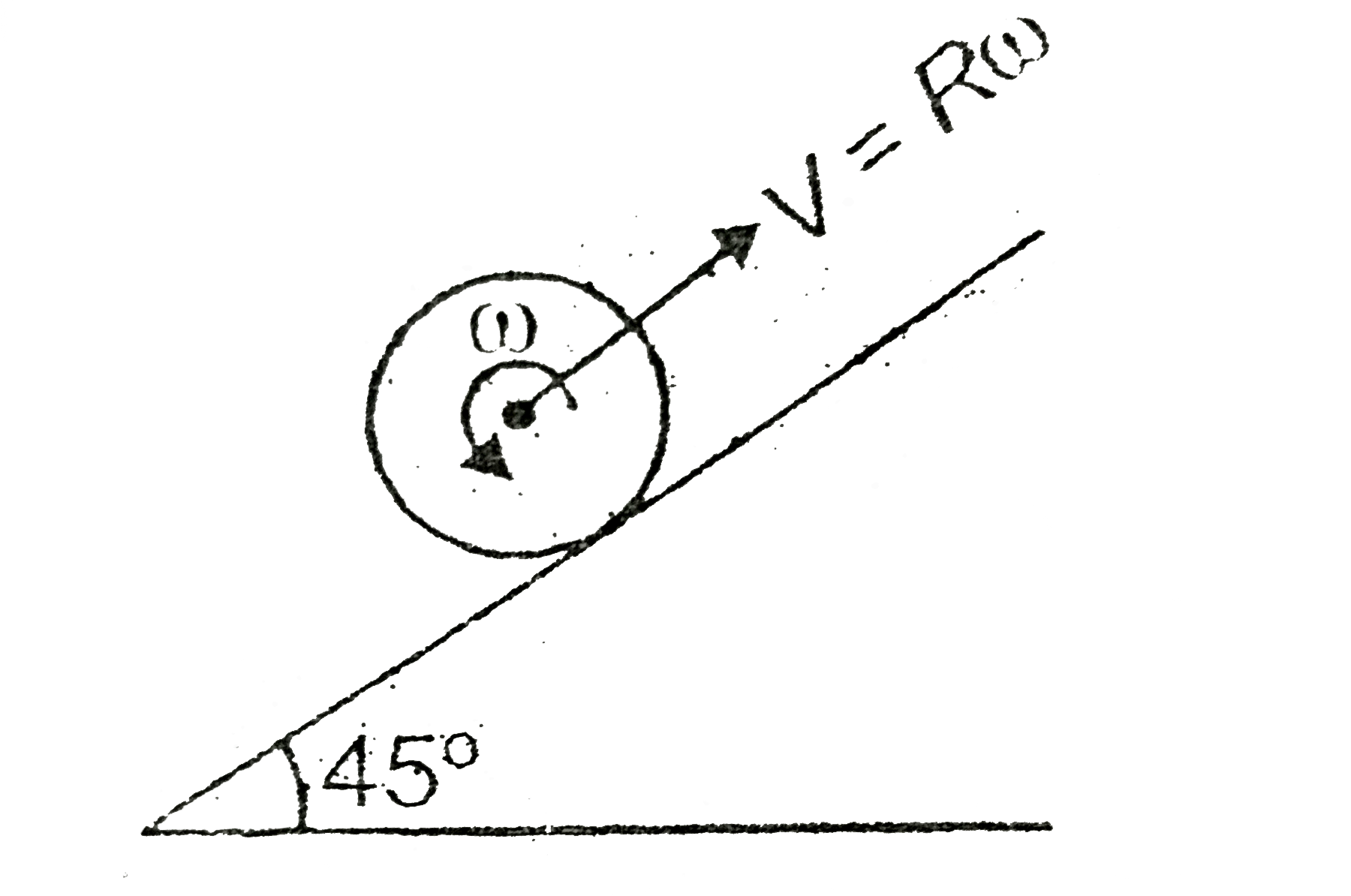
Velocity of lower most point is not zero hence it is not the case of pure rolling and friction is kinetic friction.
Since velocity of lower most point in forward direction therefore friction will act in backward direction.
Let retradation is a and angular retardation is α.
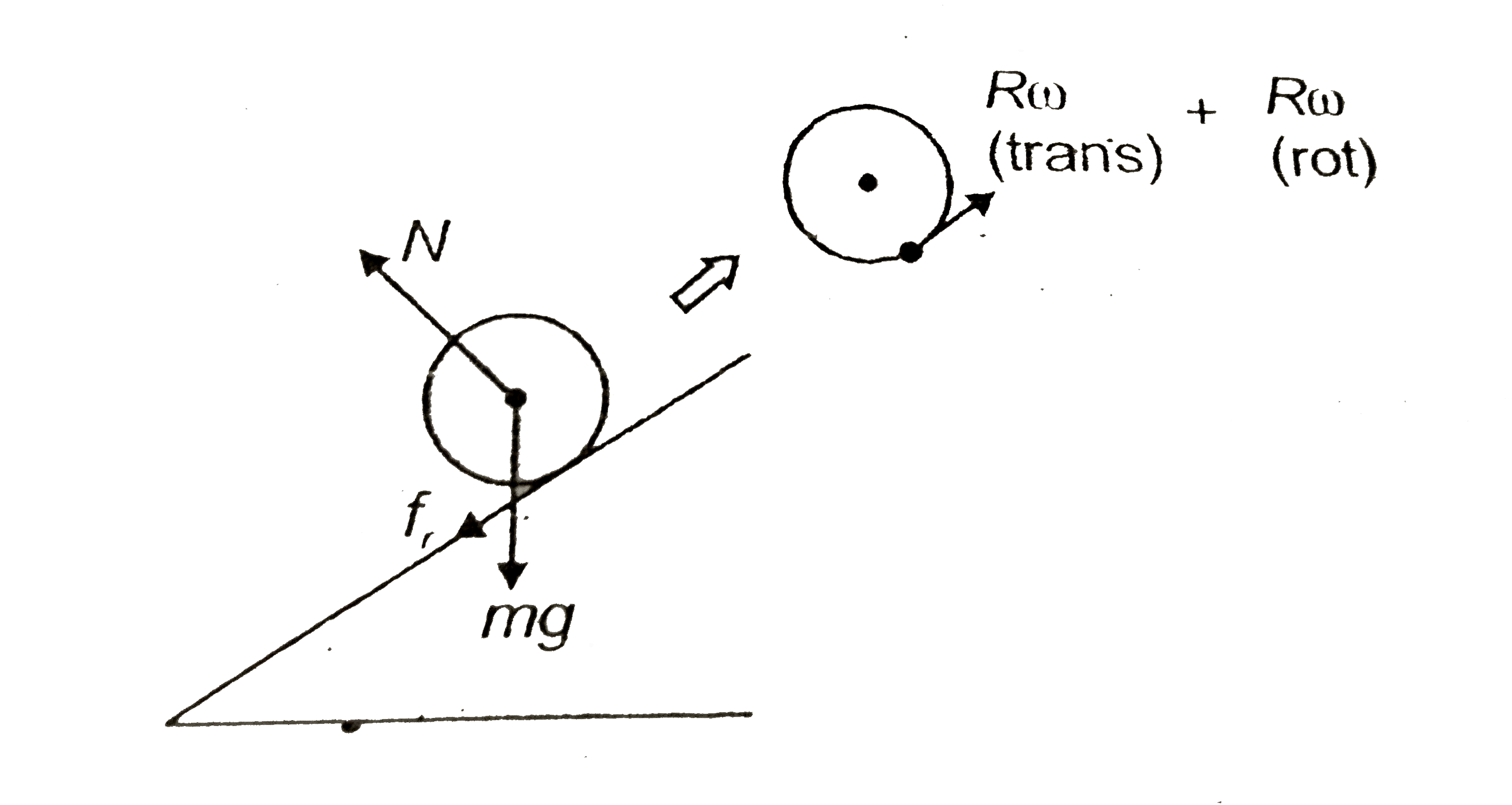
Free body diagram of sphere
⇒mgsinθ+μmgcosθ=ma
⇒a=g(sinθ+μcosθ)=g√2(1+0.5)=1.5g√2.........(i)
Now
τ due to friction =R(μmgcosθ)
⇒α=(μmgcosθ)25mR2=5gμcosθ2R=5×0.5×g×1√22R=5g4R⋅1√2=1.25g√2R.......(ii)
For translatory moion
V=V−at
=Rω−at
When velocity V becomes zero
V=0⇒t=Rωa=Rω√21.5g=√21.5(Rωg)
For rotatory motion,
ω'=ω−αt
⇒t=ωα=ω1.25g√2R=Rω√21.25g=√21.25Rωg
Linear velocity of sphere becomes zero before angular velocity become zero
Angular velocity at time t when linear velocity is zero.
ω'=ω−1.25g√2R×√2Rω1.5g
⇒ω'=ω−1.25ω1.5=ω6
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTECHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Illustration4videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - AAKASH INSTITUTECHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - A) Objective Type Questions (One option is correct)62videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - AAKASH INSTITUTECHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))5videos
SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITS
- BOOK - AAKASH INSTITUTECHAPTER - TEST 1EXERCISE - EXERCISE9videos
TEST 1
Similar Questions
solid sphere of radius r is gently placed on a rough horizontal ground with an initial angular speed ω0 and no linear velocity. If the coefficient of friction is μ, find the time t when the slipping stops. in addition state the linear velocity v and angular velocity ω at the end of slippingView SolutionAn inclined plane of angule a is fixed onto a horizontal turntable with its line of greater slope in same plane as a diameter of forntable .A small block is placed on the inclined plane a distance r from the axis the coefficient of fraction between the block and the inclined plane is μ The turntable along with incline plane spins about its axis with constant minimum angular velocity ω
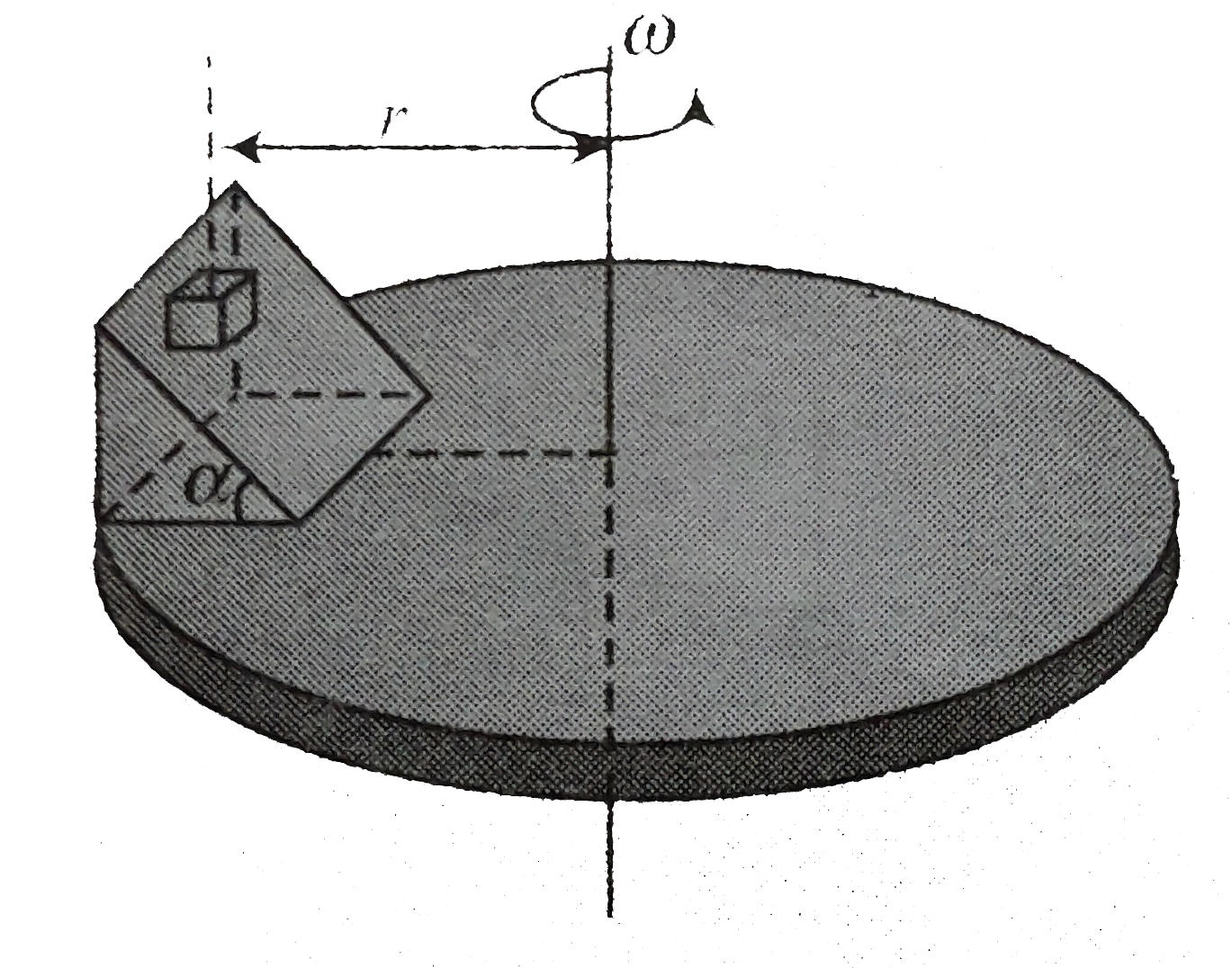
a. Find an capression for the minimum angular velocity ω to prevent the block from sliding down the plane in term of g,r,μ and the plane ω
b. Now a block of mass but having coefficient friction (with inclined plane)2μ is kept instead of the original block . find the radius of friction force acting between block and incline now to the friction force active in part (a)View Solution
Knowledge Check
- Question 1 - Select One
A body is projected up along a rough inclined plane of inclination 45∘ . The coefficient of friction is 0.5. Then the retardation of the block is
Ag2√2Bg2C3g2√2Dg√2 - Question 2 - Select One or More
A sphere is projected npon a rough inclined plane. The friction coefficient between the solid sphere and the incline isμ. The centre of the sphere is given an initial upward' velocity at t=0 without imparting any initial angular velocity. Then, which of the following statement (s) is/are true?
APure rolling will defin!tely begin before the sphere reaches the highest point and the sphere will continue to roll purely after that, even while coming down.BPure rolling will definitely begin before the sphere reaches the highest point but the sphere will continue to roll purely after that (even while coming down) only· if μ is greater- than a certain value.CThe sphere will be rolling purely while coming down if μ>(2tanα)7DThe sphere cannot roll purely while moving up the incline - Question 3 - Select One
A solid sphere is rolling on a rough surface, whose centre of mass is at C at a certain instant. Find at that instant it has angular velocity ω. Its radius is R. Find the angular acceleration at that instant mass of sphere is m
 A52gdR2B57gdR2C52d(g+ω2R)R2D57d(g+ω2R)R2
A52gdR2B57gdR2C52d(g+ω2R)R2D57d(g+ω2R)R2
A block of height h is projected along a rough surface of coefficient of friction μ. Find the point of application of the normal force on the block for μk=0.5.
View SolutionA solid cylinder of mass m and radius r is rolling on a rough inclined plane of inclination θ. The coefficient of friction between the cylinder and incline is μ. Then.
View SolutionA cylinder of mass m radius R is spined to a clockwise angular velocity ωo and then gently placed on an inclined plane for which coefficient of friction μ=tanθ,θ is the angle of inclined plane with the horizontal. The centre of mass of cylinder will remain stationary for time
 View Solution
View SolutionA car is taking turn on a circular path of radius R. If the coefficient of friction between the tyres and road is μ, the maximum velocity for no slipping is
View SolutionA sphere is projected up an inclined plane with a velocity v0 and zero angular velocity as shown. The coefficient of friction between the sphere and the plane is μ=tanθ.. Find the total time of rise of the sphere.
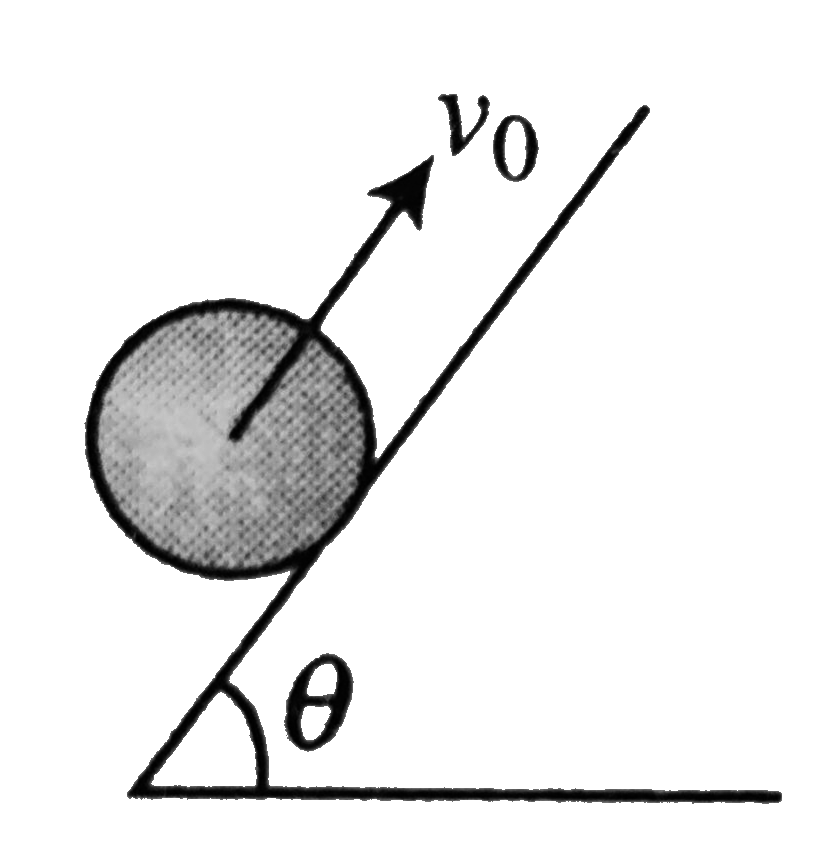 View Solution
View Solution

