P is a point on the median AD of the triangle ABC. Prove that triangle APB = triangle APC.
Step by step video & image solution for P is a point on the median AD of the triangle ABC. Prove that triangle APB = triangle APC. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
X is a point on median AL of triangle ABC. Prove that triangle ABX is equal in area.
View SolutionAD is a median of triangle ABC. Prove that : AB+AC>2AD
View SolutionIn the adjoining figure, E is a point on the median AD of a ΔABC. Show that ar (ΔABE)=(ΔACE).
View SolutionAD is a median of triangle ABC. Prove that : AB+BC+AC>2AD
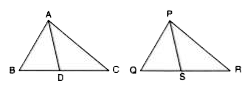
View SolutionThe sides AB and BC and the median AD of triangle ABC are equal to the sides PQ and QR and the median PM of triangle PQR respectively. Prove that the triangles ABC and PQR are congruent.
View SolutionThe sides AB and BC and the median AD of triangle ABC are equal to the sides PQ and QR and the median PM of triangle PQR respectively. Prove that the triangles ABC and PQR are congruent.
View SolutionLet A(5,−3),B(2,7)andC(−1,2) be the vertices of a △ABC. If P is a point inside the triangle ABC such that the triangle APC,APB and BPC have equal areas, then length of the line segment PB is:
View SolutionLet A(5,−3),B(2,7)andC(−1,2) be the vertices of a △ABC. If P is a point inside the triangle ABC such that the triangle APC,APB and BPC have equal areas, then length of the line segment PB is:
View SolutionIn the following figures, the sides AB and BC and the median AD of triangle ABC are respectively equal to the sides PQ and QR and median PS of the triangle PQR. Prove that ΔABCandΔPQR are congruent.
View SolutionLet A(1,0),B(6,2) and C(32,6) be the vertices of a triangle ABC. If P is a point inside the triangle ABC such that the triangles APC, APB and BPC have equal areas , then the length of the line segment PQ, where Q is the point (−76,−13), is _________.
View Solution
