consider the triangle OAB in the xy- plane where O=(0,0),A=(6,0),B=(√2,3). A square PQRS is inscribed in the square with P,Q on OA, R on AB and S on BO. Then the side of the square equals:
Step by step video & image solution for consider the triangle OAB in the xy- plane where O=(0,0),A=(6,0), B=(sqrt(2),3). A square PQRS is inscribed in the square with P,Q on OA, R on AB and S on BO. Then the side of the square equals: by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
A square is inscribed in the circle x2+y2−10x−6y+30=0. One side of the square is parallel to y=x+3, then one vertex of the square is
A(3, 3)B(7, 3)C(6,3−√3)D(6,3+√3)The area of ΔABC with vertices A(a, 0), O(0, 0) and B(0, b) in square units is
AabB12abC12a2b2D12b2ABC is an equilateral triangle and PQRS is a square inscribed in the triangle is such a way that P and Q lie on AB and R , S lie on BC and AC respectively . What is the value of RC: RB ?
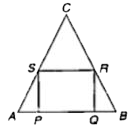 A1:√2B1:√3C√3:2D1:2
A1:√2B1:√3C√3:2D1:2
a square is inscribed in the circle x2+y2−10x−6y+30=0. One side of the square is parallel to y=x+3, then one vertex of the square is :
View SolutionConsider triangle AOB in the x−y plane, where A≡(1,0,0),B≡(0,2,0)andO≡(0,0,0). The new position of O, when triangle is rotated about side AB by 900 can be a. (45,35,2√5) b. (−35,√25,2√5) c. (45,25,2√5) d. (45,25,1√5)
View SolutionA square is inscribed in a circle x2+y2−4x−6y+5=0 whose sides are parallel to coordinate axis then the vertex of the square is/are
View SolutionFind the coordinates of the vertices of a square inscribed in the triangle with vertices A(0,0),B(2,1)andC(3,0), given that two of its vertices are on the side AC.
View SolutionConsider a triange ABC, where B and C are (−a,0)and(a,0) respectively . A be any point (h,k) P,Q,R divides the sides of this triangle in same ratio. Then centroid of ΔPQR is
View Solution
