Given an are PQ of a circle subtending angles POQ at the centre PAQ at a point A on the remaining part of the circle. We need to prove that ∠POQ=2∠PAQ.
Step by step video solution for Given an are PQ of a circle subtending angles POQ at the centre PAQ at a point A on the remaining part of the circle. We need to prove that anglePOQ=2 anglePAQ. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
(A) Choose the correct alternative :
O is a centre of a circle, Tangents TP and TQ of the circles itersect at point T in the exterior of the circle. Points P and Q lie on the circle . If ∠POQ=120∘ then ∠PTQ=?A120∘B30∘C60∘D90∘
Prove that equal chords of a circle subtend equal angles at the centre.
View SolutionThe angle subtended by an arc of a circle at the centre is double the angle subtended by it any point on the remaining part of the circle.
View SolutionProve Equal chords of congruent circles subtend equal angles at the centre.
View SolutionTheorem 10.8 : The angle subtended by an arc at the centre is double the angle subtended by it at any point on the remaining part of the circle.
View SolutionTheorem 10.8 : The angle subtended by an arc at the centre is double the angle subtended by it at any point on the remaining part of the circle.
View SolutionProve that if chords of congruent circles subtend equal angles at their centres, then the chords are equal.
View SolutionIn the figure given below, SPT is a tangent to the circle at P and O is the centre of the circle. If ∠QPT=α, then what is ∠POQ equal to ?
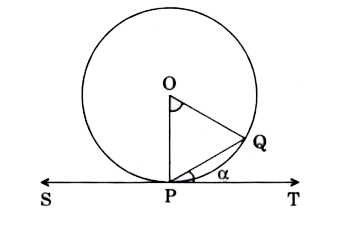
View Solution
