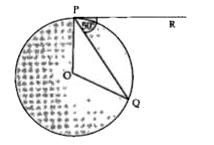
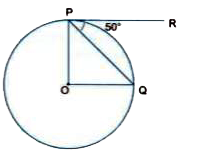
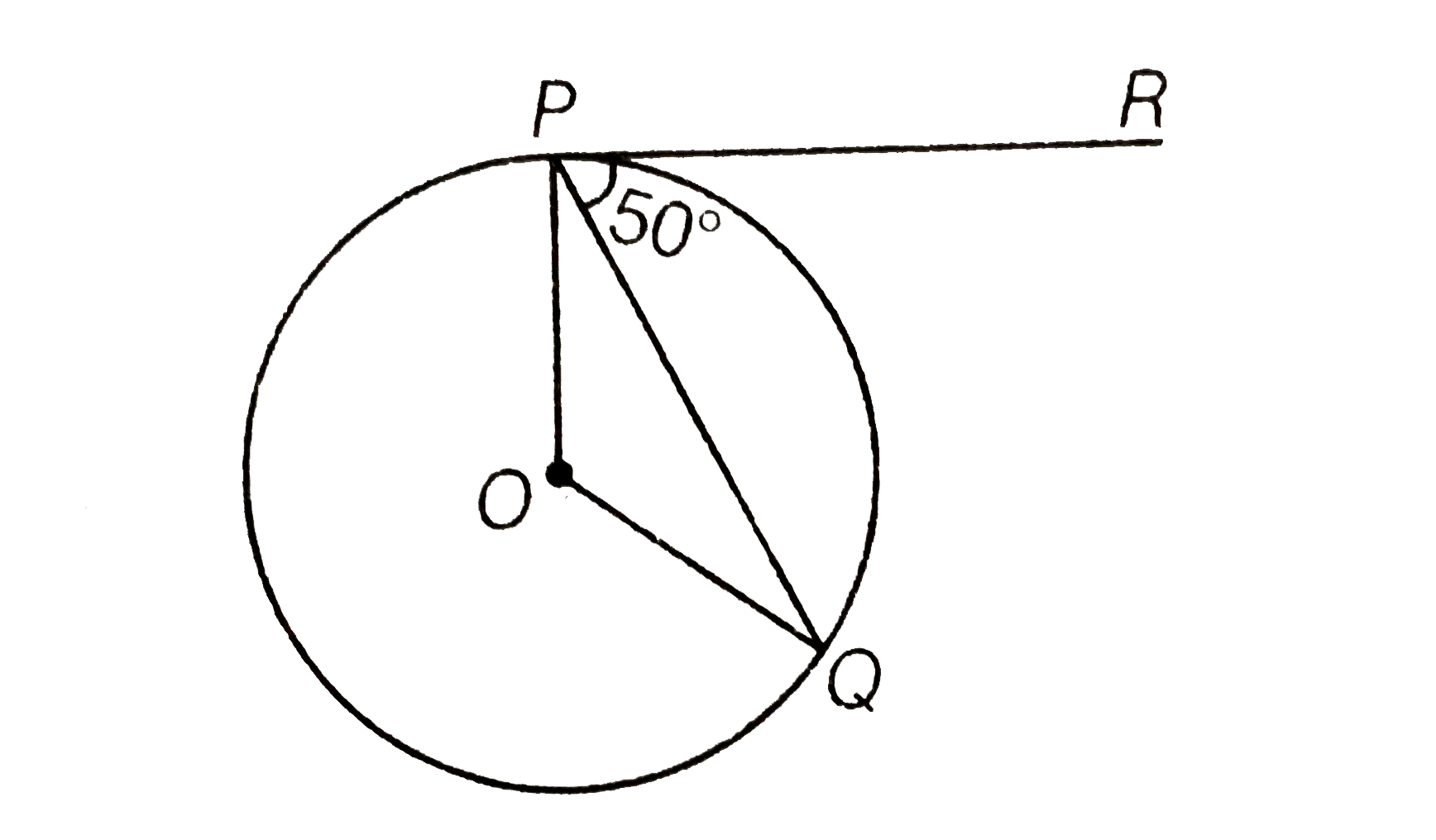
In figure, if is the centre of a circle, PQ is a chord and the tangent PR at P makes an angle of with PQ, then is equal to
A
B
C
D
Video Solution
Text Solution
The correct Answer is:A
Given,
We know that, the tangent at any point of a circle is perpendicular to the through the point of contact.
[from figure]
Now, OP = OQ = Radius of circle
[since, angle opposite to equal sides are equal]
In ,
[since, sum of angles of a triangle ]
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
In figure, if O is the centre of a circle, PQ is a chord and the tangent PR at P makes an angle of 50∘ with PQ, then ∠POQ is equal to
 A100∘B80∘C90∘D75∘
A100∘B80∘C90∘D75∘ - Question 2 - Select One
In the figure , PQ is a chord of a circle and PT is a tangent at P such that ∠QPT=60∘, then ∠PRQ =
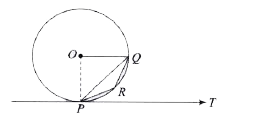 A100∘B110∘C120∘D150∘
A100∘B110∘C120∘D150∘ - Question 3 - Select One
In figure, if PQR is the tangent to a circle at Q whose centre is O, AB is a chord parallel to PR and ∠BQR=70∘ then ∠AQB is equal to
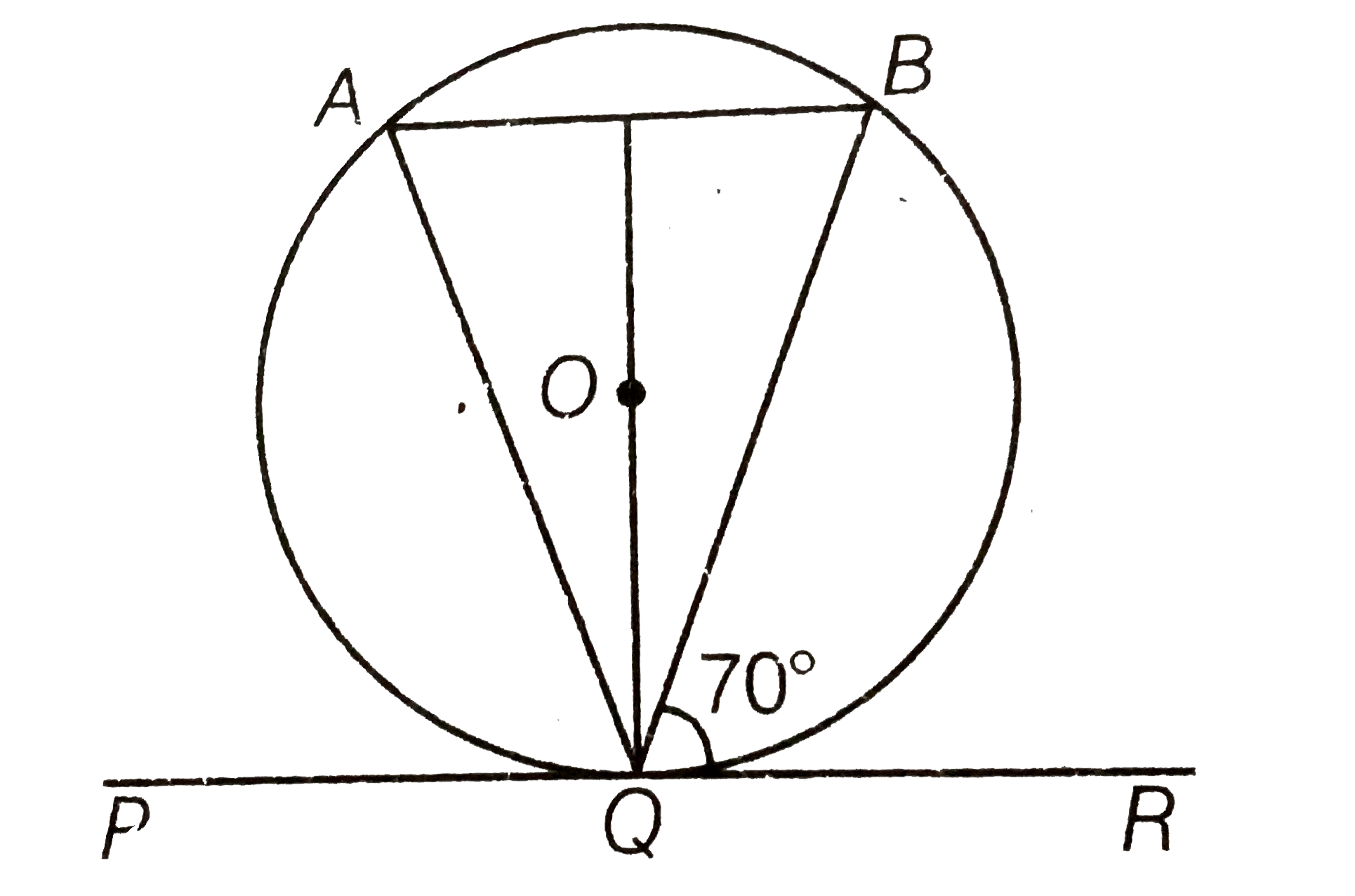 A20∘B40∘C35∘D45∘
A20∘B40∘C35∘D45∘
In Figure 1, O is the centre of a circle, PQ is a chord and PT is the tangent at P. If angle POQ = 70°, then angleTPQ is equal to
View SolutionIn the adjoining figure 'O' is the centre of the circle and PQ, PR and ST are the three tangents. ∠QPR=50∘, then the value of ∠SOT is :
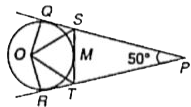 View Solution
View SolutionIn figure, O is the centre of a circle. PT and PQ are tangents to the circle from an external point P. If ∠TPQ=70∘, find ∠TRQ.
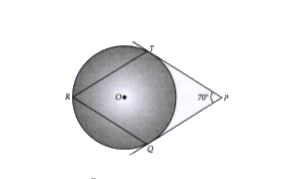 View Solution
View SolutionIn the figure,O is the centre of the circle PQ is the tangent at point P then what is the measure of ∠OPQ ?Why ?
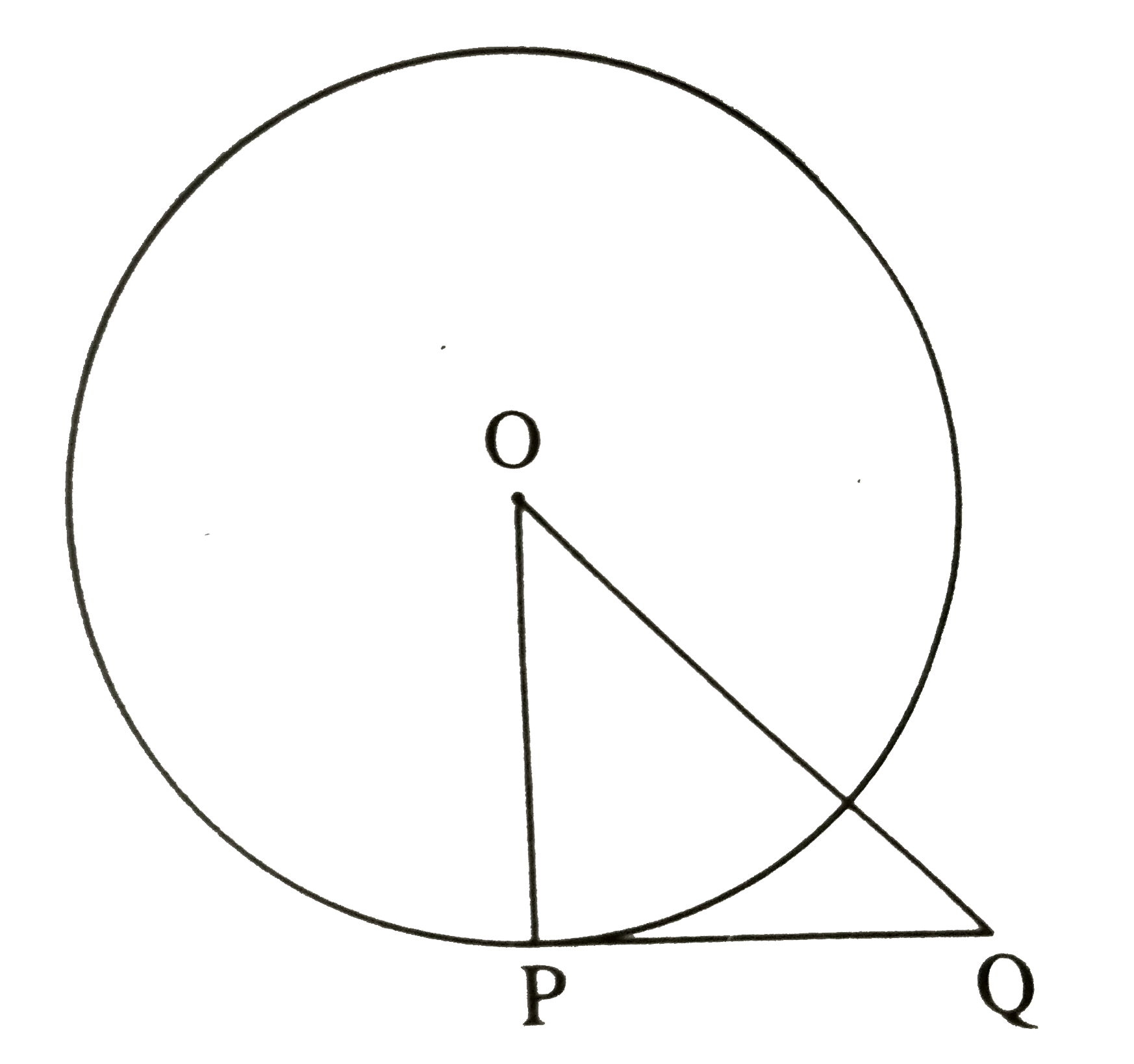 View Solution
View SolutionIn the figure, O is the centre of the circle and LN is a diameter. If PQ is a tangent to the circle at K and ∠KLN=30∘, find ∠PKL.
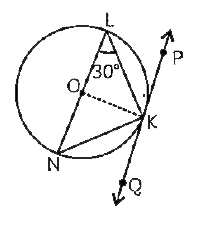 View Solution
View Solution