Let circle c1, be inscribed in a square with side length 1. As shown in figure smaller circle c1 is inscribed in the lower right corner of the square so that c1 is tangent to c2 and the two sides of the square then the area of the c2 is
Step by step video & image solution for Let circle c_1, be inscribed in a square with side length 1. As shown in figure smaller circle c_1 is inscribed in the lower right corner of the square so that c_1 is tangent to c_2 and the two sides of the square then the area of the c_2 is by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Consider three circles C1,C2andC3 such that C2 is the director circle of C1,andC3 is the director circle of C2. Tangents to C1, from any point on C3 intersect C2, at PandQ. Find the angle between the tangents to C2 at P and Q. Also identify the locus of the point of intersec- tion of tangents at PandQ.
View SolutionA circle is inscribed in an equilateral triangle of side a. The area of any square inscribed in this circle is
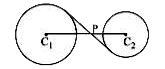
View Solutionदिखाए गये चित्रानुसार दोनो वृतों के केंद्रों C1 तथा C2 के मध्य दुरी 35 है अर्थात C1C2=35 तथा C1 व C2 केंद्रों वाले वृतों की त्रिज्याएँ क्रमशः 12 व 9 है । यदि वृतों की उभयनिष्ठ आंतरिक स्पर्श रेखा तथा C1C2 का प्रतिच्छेद बिंदु P है, तो l(C1P) का मान होगा
(i) 18 (ii) 20 (iii) 12 (iv) 15
View SolutionIn the figure given, two circles with centres Ct and C2 are 35 units apart, i.e. C1C2=35. The radii of the circles with centres C1 and C2 are 12 and 9 respectively. If P is the intersection of C1C2 and a common internal tangent to the circles, then l(C1P) equals
View SolutionLet C1and C2 be two circles with C2 lying inside C1 circle C lying inside C1 touches C1 internally andexternally. Identify the locus of the centre of C
View SolutionIn the figure given, two circles with centres Ct and C2 are 35 units apart, i.e. C1C2=35. The radii of the circles with centres C1 and C2 are 12 and 9 respectively. If P is the intersection of C1C2 and a common internal tangent to the circles, then l(C1P) equals
View SolutionLet C_1 and C_2 be two circles with C_2 lying inside C_1 A circle C lying inside C_1 touches C_1 internally and C_2 externally. Identify the locus of the centre of C
View SolutionLet C1and C2 be two circles with C2 lying inside C1 A circle C lying inside C1 touches C1 internally and C2 externally. Identify the locus of the centre of C
View Solution