An elevator is descending with uniform acceleration.To measure the acceleration, a person in the elevator drops a coin at momen the elevator strts. The coin is 6 ft asbove the floor of the elevator at the time it is dropped. The person observes that the coin strikes the floor in 1 second. Calculate these dta the acceleration of the elevator.
To solve the problem of the descending elevator and the dropped coin, we can follow these steps:
Step 1: Understand the scenario
We have an elevator descending with uniform acceleration a. A coin is dropped from a height of 6 feet above the floor of the elevator at the moment the elevator starts moving. The coin takes 1 second to hit the floor of the elevator.
Step 2: Define the variables
- Let a be the acceleration of the elevator (downward).
- The acceleration due to gravity g is approximately 32.2ft/s2 (downward).
- The initial velocity of both the elevator and the coin is 0ft/s since they start from rest.
- The distance the coin falls relative to the elevator is −6ft (since it falls downwards).
Step 3: Write the equations of motion
Using the equation of motion for the coin with respect to the elevator:
xe/c=ue/c⋅t+12(ae−ac)t2
Where:
- xe/c=−6ft (the displacement of the coin relative to the elevator)
- ue/c=0ft/s (initial velocities are zero)
- ae=a (acceleration of the elevator)
- ac=g=32.2ft/s2 (acceleration of the coin)
Substituting the values into the equation gives:
−6=0+12(a−32.2)(12)
Step 4: Simplify the equation
This simplifies to:
−6=12(a−32.2)
Multiplying both sides by 2:
−12=a−32.2
Step 5: Solve for a
Rearranging the equation to solve for a:
a=−12+32.2
a=20.2ft/s2
Step 6: Conclusion
The acceleration of the elevator is 20.2ft/s2 downward.
---
We have an elevator descending with uniform acceleration a. A coin is dropped from a height of 6 feet above the floor of the elevator at the moment the elevator starts moving. The coin takes 1 second to hit the floor of the elevator.
- Let a be the acceleration of the elevator (downward).
- The acceleration due to gravity g is approximately 32.2ft/s2 (downward).
- The initial velocity of both the elevator and the coin is 0ft/s since they start from rest.
- The distance the coin falls relative to the elevator is −6ft (since it falls downwards).
Using the equation of motion for the coin with respect to the elevator:
xe/c=ue/c⋅t+12(ae−ac)t2
Where:
- xe/c=−6ft (the displacement of the coin relative to the elevator)
- ue/c=0ft/s (initial velocities are zero)
- ae=a (acceleration of the elevator)
- ac=g=32.2ft/s2 (acceleration of the coin)
−6=0+12(a−32.2)(12)
This simplifies to:
−6=12(a−32.2)
Multiplying both sides by 2:
−12=a−32.2
Rearranging the equation to solve for a:
a=−12+32.2
a=20.2ft/s2
The acceleration of the elevator is 20.2ft/s2 downward.
Topper's Solved these Questions
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Objective 2
10 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMACHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Exercises
34 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises
86 Videos
Similar Questions
An elevator (lift) ascends with an upward acceleration of 1.2ms−2. At the instant when its upward speed is 2.4ms−1, a loose bolt drops from the ceiling of the elevator 2.7m above the floor of the elevator. Calculate (a) the time of flight of the bolt from the ceiling to the floor and (b) the distance it has fallen relaative to the elevator shaft.
View SolutionAn elevator is moving with an upward acceleration a,A coin is dropped from rest from the roof of the elevator, frlative to you. After what time the coin will strike the base of the elevator?
View Solution
Knowledge Check
The force exerted by the floor of an elevator on the foot of a person standing there is more than the weight of the person if the elevator is :
AGoing up and slowing downBGoint up and speeding upCGoing down and slowing downDGoing down and speeding upAn elevator is moving vertically up with a acceleration a the force exerted on the floor by a passenger of mass m is
AmgBmaCmg - maDmg + ma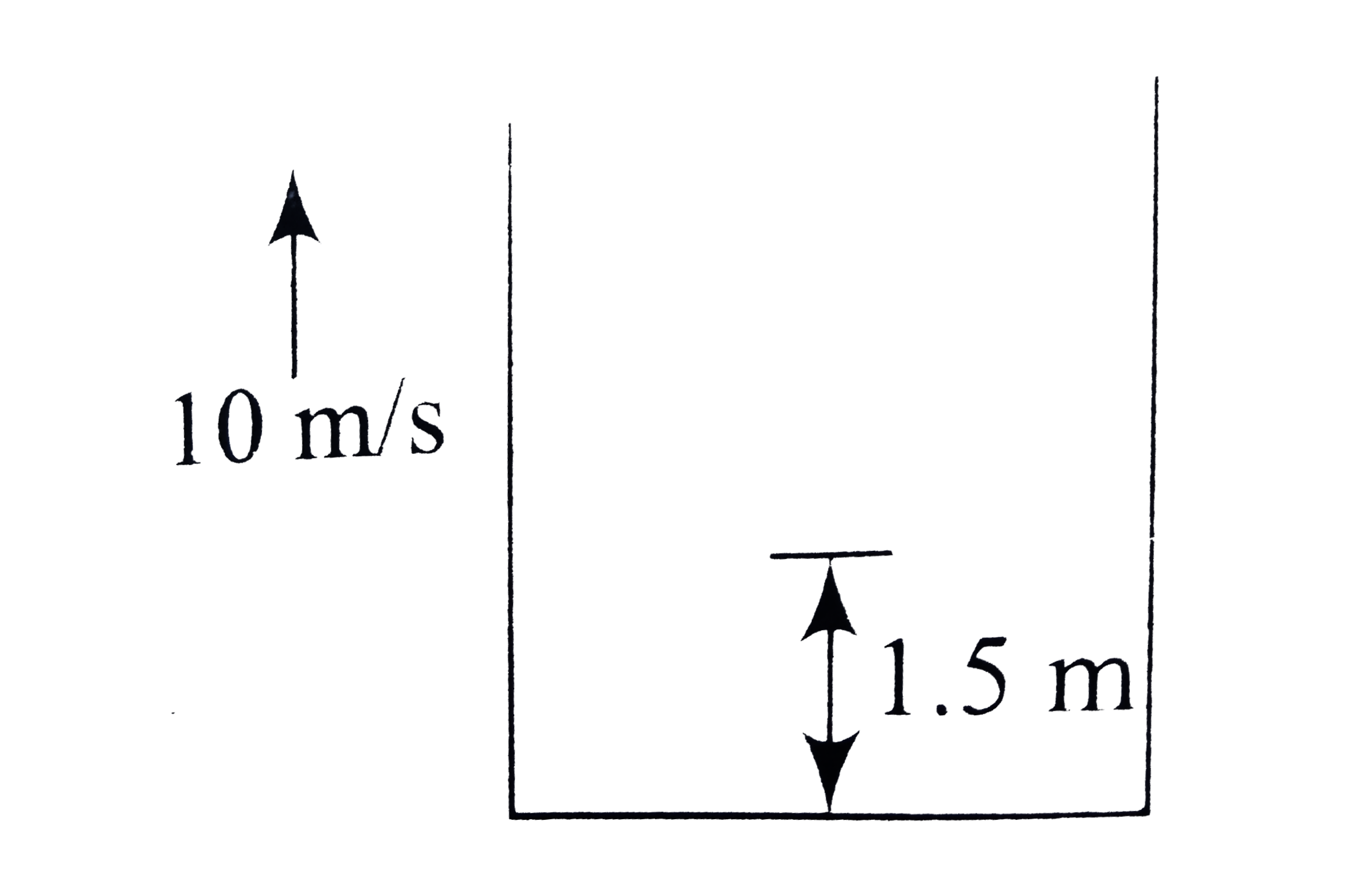
A boy in the elevator shoots a bullet in a vertical upward direction from a height of 1.5 m above the floor of the elevator. There is no roof in the elevator. The floor of the elevator is at 50 m from ground at the instant when velocity of the elevator is 10 m/s in upward direction. The bullet strikes the floor of the elevator in 2 seconds. The initial speed of the bullet is 15 m/s relative to the elevator.
Q. Find the acceleration of the elevator in upward direction, (mss2):A5B5.25C5.75D6.25
An elevator (lift) ascends with an upward acceleration of 1.2 ms−2.At the instant when its upward speed is 2.4 ms−1, a loose bolt drops from the ceiling of the elevator 2.7 m above the floor of the elevator . Calculate (a) the time of flight of the bolt from the ceiling to the floor and (b)the distance it has fallen relative to the elevator shaft.
View SolutionA person standing oin the floor of an elevator drops as coin. The coin reaches the floor of the elevator in a time t1 if the elevator is stationary and in the t2 if it is moving uniformly. Then
View SolutionAn elevator is accelerating downwards with an acceleration of 4.9 ms−2. A barometer placed in this elevator reads 75 cm of Hg. Calculate the air pressure inside the elevator.
View SolutionAn elecator in which a man is standing is moving upwards with a speed of 10ms−1 if the man drops a coin from a height of 2.45 m from the floor of elevator it reaches the floor of the elevator after time (g=9.8ms−2)
View SolutionA train is moving forward with a horizontal acceleration a. A man sitting in the train drops a coin on the floor of the train . The acceleration of the coin w.r.t man is
View Solution
