A block of mass m moving at a speed v compresses as spring thrugh a distance x before its speed is halved. Find the spring constant of the spring.
The correct Answer is:B, C, D
To find the spring constant k of the spring when a block of mass m compresses the spring through a distance x before its speed is halved, we can use the principle of conservation of energy. Here’s a step-by-step solution:
Step 1: Understand the initial and final states
- Initially, the block is moving with speed v and has kinetic energy given by:
KEinitial=12mv2
- When the block compresses the spring by a distance x, its speed becomes v2. The kinetic energy at this point is:
KEfinal=12m(v2)2=12m⋅v24=18mv2
Step 2: Write the energy conservation equation
- According to the conservation of energy, the initial kinetic energy minus the final kinetic energy is equal to the potential energy stored in the spring:
KEinitial−KEfinal=PEspring
- The potential energy stored in the spring when compressed by distance x is given by:
PEspring=12kx2
Step 3: Set up the equation
- Substitute the expressions for kinetic energy into the energy conservation equation:
12mv2−18mv2=12kx2
Step 4: Simplify the equation
- Combine the kinetic energy terms on the left:
12mv2−18mv2=48mv2−18mv2=38mv2
- Now the equation becomes:
38mv2=12kx2
Step 5: Solve for the spring constant k
- Rearranging the equation to isolate k:
k=3mv24x2
Final Answer
The spring constant k is given by:
k=3mv24x2
- Initially, the block is moving with speed v and has kinetic energy given by:
KEinitial=12mv2
- When the block compresses the spring by a distance x, its speed becomes v2. The kinetic energy at this point is:
KEfinal=12m(v2)2=12m⋅v24=18mv2
- According to the conservation of energy, the initial kinetic energy minus the final kinetic energy is equal to the potential energy stored in the spring:
KEinitial−KEfinal=PEspring
- The potential energy stored in the spring when compressed by distance x is given by:
PEspring=12kx2
- Substitute the expressions for kinetic energy into the energy conservation equation:
12mv2−18mv2=12kx2
- Combine the kinetic energy terms on the left:
12mv2−18mv2=48mv2−18mv2=38mv2
- Now the equation becomes:
38mv2=12kx2
- Rearranging the equation to isolate k:
k=3mv24x2
The spring constant k is given by:
k=3mv24x2
Topper's Solved these Questions
Similar Questions
A block of mass m moving at a speed v compresses a spring throgh a distance x before its speed is halved. Find the spring constant of the spring.
View SolutionA block of mass m moving at a speed v compresses a spring through a distance x before its speed is halved. The spring constant of the spring is 6mv2nx2. Find value of n.
View Solution
Knowledge Check
A block of mass m is moving with a speed v on a horizontal rough surface and collides with a horizontal mounted spring of spring constant k as shown in the figure .The coefficient of friction between the block and the floor is μ The maximum compression of the spring is
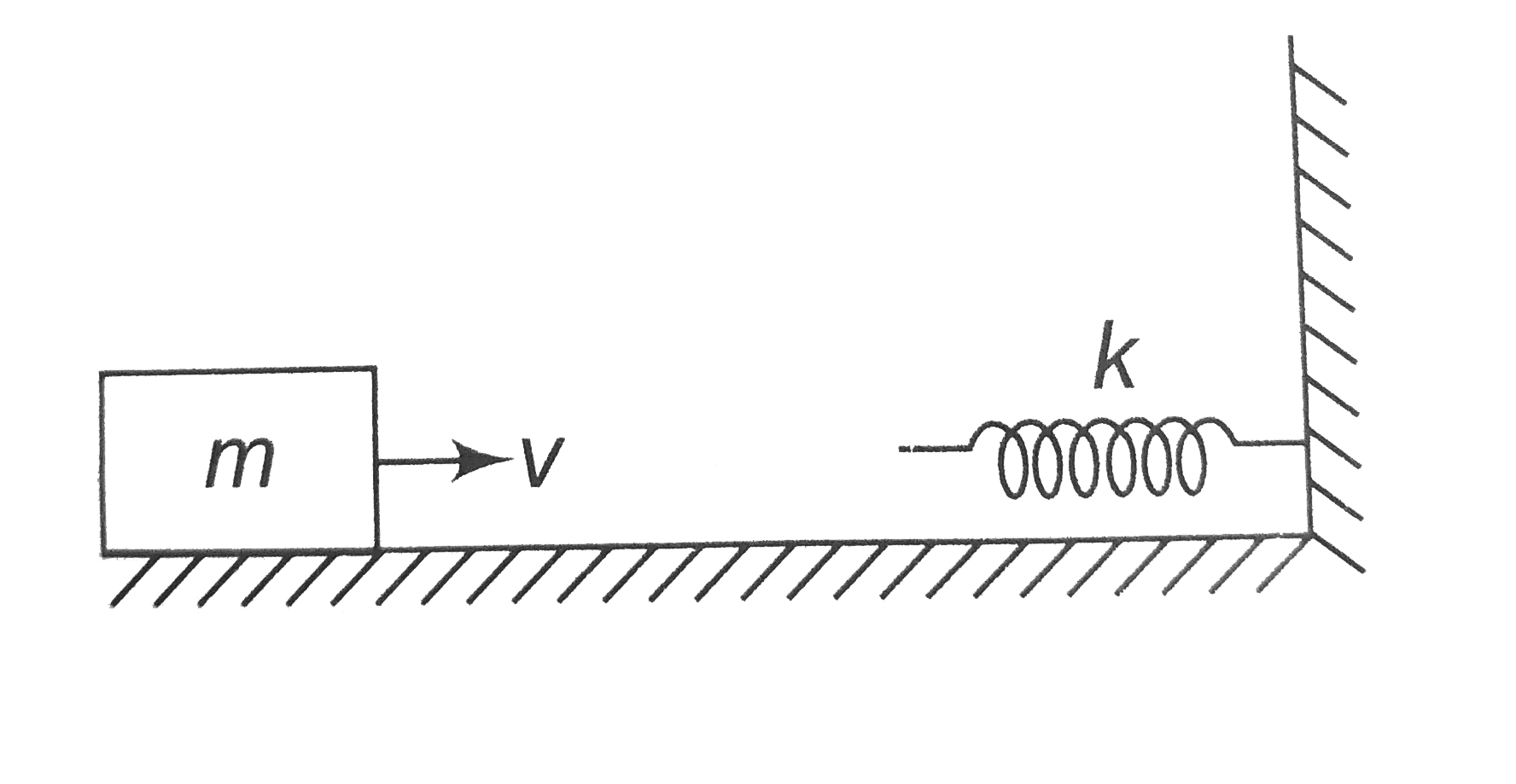 A−μmgk+1k√(μmg)2+mkv2Bμmgk+1k√(μmg)2+mkv2C−μmgk+1k√(μmg)2−mkv2Dμmgk+1k√(μmg)2+mkv2
A−μmgk+1k√(μmg)2+mkv2Bμmgk+1k√(μmg)2+mkv2C−μmgk+1k√(μmg)2−mkv2Dμmgk+1k√(μmg)2+mkv2A block of 200 g mass is dropped from a height of 2 m on to a spring and compress the spring to a distance of 50 cm. The force constant of the spring is
A20Nm−1B40Nm−1C30Nm−1D60Nm−1A block of mass m is pushed up against a spring, compressing it a distance x, and the block is then released. The spring projects the block along a frictionaless horizontal surface, grving the block a speed v. The same spring projects a second block of mass 4m, giving it a speed 3v. What distance was the spring compressed in the second case ?
A6xBx6C36xD12x
A block of mass m moving at a speed v0 compresses a spring of spring constant k as shown in the figure. Find (a) the maximum compression of spring and (b) speed of the block when the spring is compressed to half of maximum compression.
View SolutionBlock of mass m is pushed towards a horizontal spring as shown in figure , with initial speed u .

Spring constant of the spring is K and floor is smooth . Calculate maximum possible compression of the spring .
View SolutionA block of mass m is released from a height h from the top of a smooth surface. There is an ideal spring of spring constant k at the bottom of the track. Find the maximum compression in the spring (Wedge is fixed)

View Solution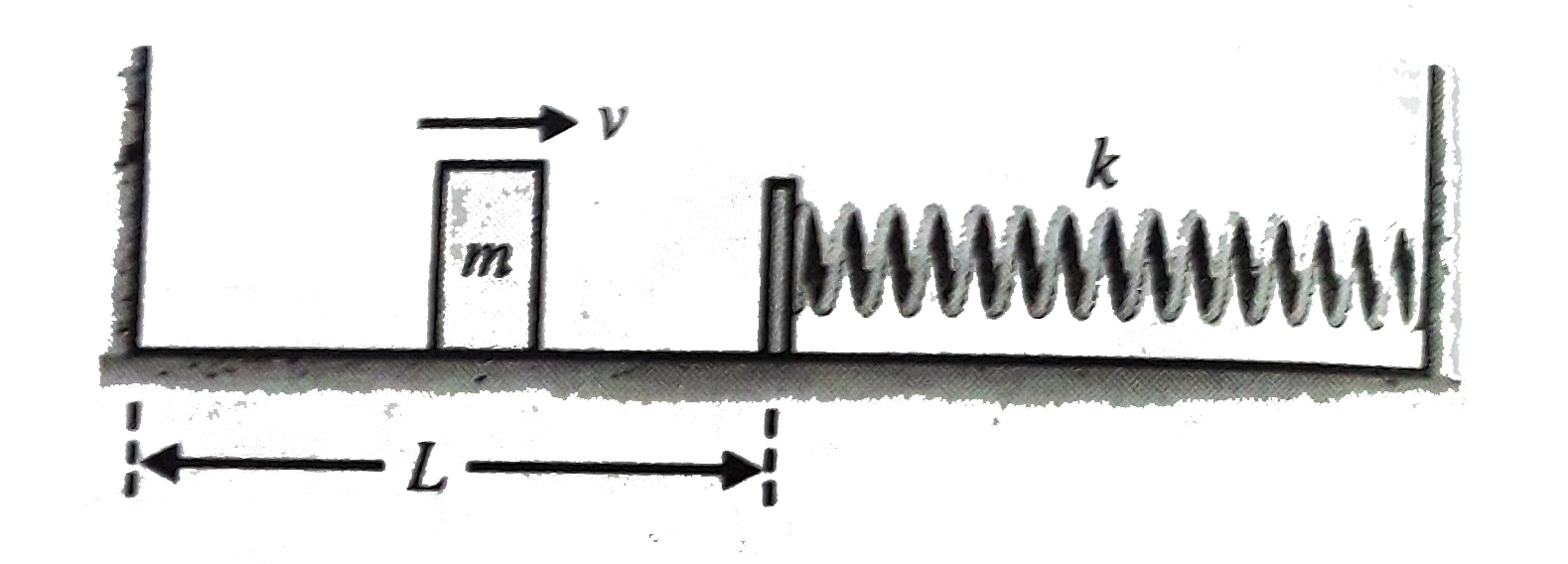
A spring having a spring constant k is fixed to a vertical wall as shown in Fig. A block of mass m moves with velocity v torards the spring from a parallel wall opposite to this wall. The mass hits the free end of the spring compressing it and is decelerated by the spring force and comes to rest and then turns the spring is decelerated by the spring force and comes to rest and then turns back till the spring acquires its natural length and contact with the spring is broken. In this process, it regains its angular speed in the opposite direction and makes a perfect elastice collision on the opposite left wall and starts moving with same speed as before towards right. The above processes are repeated and there is periodic oscillation.
Q. What is the time period of oscillation ?
View Solution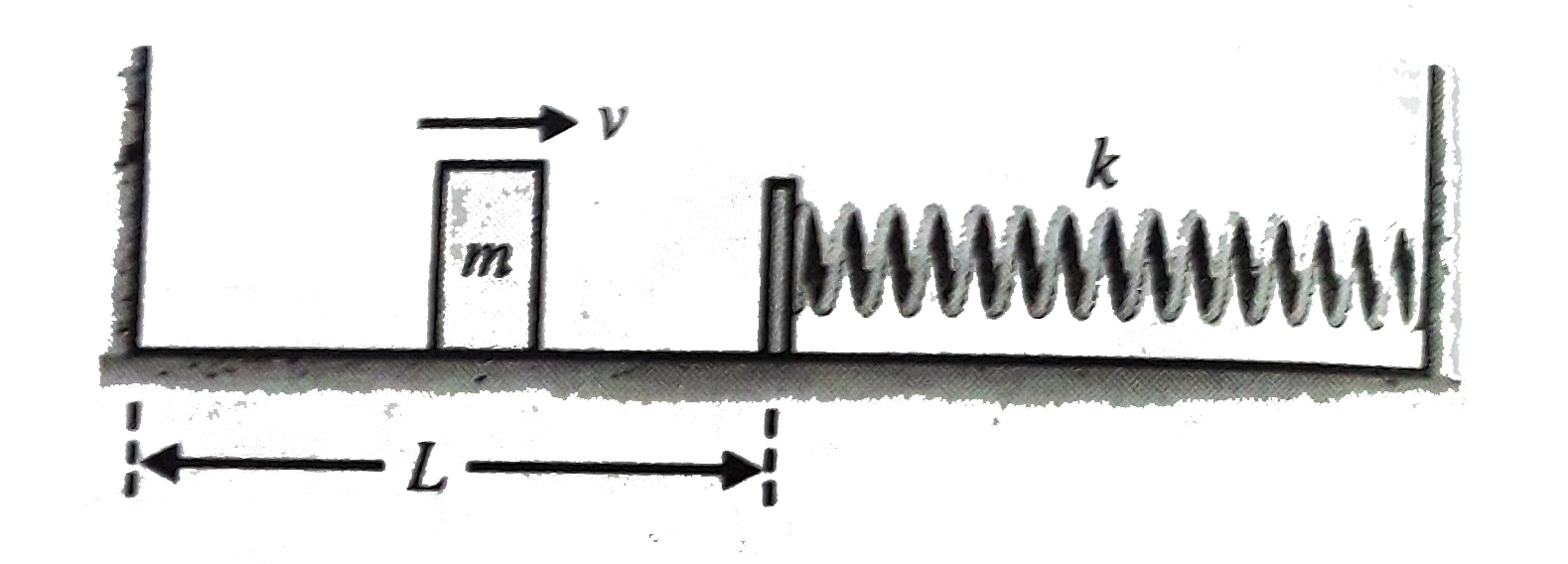
A spring having a spring constant k is fixed to a vertical wall as shown in Fig. A block of mass m moves with velocity v torards the spring from a parallel wall opposite to this wall. The mass hits the free end of the spring compressing it and is decelerated by the spring force and comes to rest and then turns the spring is decelerated by the spring force and comes to rest and then turns back till the spring acquires its natural length and contact with the spring is broken. In this process, it regains its angular speed in the opposite direction and makes a perfect elastice collision on the opposite left wall and starts moving with same speed as before towards right. The above processes are repeated and there is periodic oscillation.
Q. What is the maximum compression produced in the spring?
View Solution