The bob of a stationary pendulum is given a sharp hit to impart it a horizontal speed of √3gl. Find the angle rotated by the string before it becomes slack.
The correct Answer is:A, C
To solve the problem of finding the angle rotated by the string of a pendulum before it becomes slack after being given a horizontal speed of √3gl, we can follow these steps:
Step 1: Understand the Initial Conditions
The pendulum bob is initially at rest and is given a horizontal speed of v=√3gl. We need to find the angle θ at which the tension in the string becomes zero.
Step 2: Apply Conservation of Energy
At the initial point (point A), the bob has kinetic energy and no potential energy (since we take the reference level at the height of the bob). The kinetic energy KEA at point A is given by:
KEA=12mv2=12m(√3gl)2=32mgl
At point B, when the bob has rotated to angle θ, it has both kinetic energy and potential energy. The potential energy PEB at point B is:
PEB=mgh=mg(l−lcosθ)=mg(l(1−cosθ))
The kinetic energy KEB at point B is:
KEB=12mv2
By conservation of energy, we have:
KEA=KEB+PEB
Substituting the expressions we found:
32mgl=12mv2+mg(l(1−cosθ))
Step 3: Simplify the Energy Equation
Cancelling m from both sides (assuming m≠0):
32gl=12v2+g(l(1−cosθ))
Rearranging gives:
32gl−g(l−lcosθ)=12v2
This simplifies to:
32gl−gl+glcosθ=12v2
12gl+glcosθ=12v2
Step 4: Express v2 in Terms of θ
From the centripetal force requirement when the tension is zero, we have:
mgcosθ=mv2l
Cancelling m gives:
gcosθ=v2l
Thus,
v2=glcosθ
Step 5: Substitute v2 into the Energy Equation
Substituting v2 back into the energy equation:
12gl+glcosθ=12(glcosθ)
This leads to:
12gl+glcosθ=12glcosθ
Rearranging gives:
12gl=12glcosθ−glcosθ
12gl=−12glcosθ
1=−cosθ
This gives:
cosθ=13
Step 6: Find the Angle Rotated
The angle θ is given by:
θ=cos−1(13)
The angle rotated before the string becomes slack is:
Angle rotated=π−θ=π−cos−1(13)
Final Answer
Thus, the angle rotated by the string before it becomes slack is:
π−cos−1(13)
The pendulum bob is initially at rest and is given a horizontal speed of v=√3gl. We need to find the angle θ at which the tension in the string becomes zero.
At the initial point (point A), the bob has kinetic energy and no potential energy (since we take the reference level at the height of the bob). The kinetic energy KEA at point A is given by:
KEA=12mv2=12m(√3gl)2=32mgl
At point B, when the bob has rotated to angle θ, it has both kinetic energy and potential energy. The potential energy PEB at point B is:
PEB=mgh=mg(l−lcosθ)=mg(l(1−cosθ))
The kinetic energy KEB at point B is:
KEB=12mv2
KEA=KEB+PEB
Substituting the expressions we found:
32mgl=12mv2+mg(l(1−cosθ))
Cancelling m from both sides (assuming m≠0):
32gl=12v2+g(l(1−cosθ))
Rearranging gives:
32gl−g(l−lcosθ)=12v2
This simplifies to:
32gl−gl+glcosθ=12v2
12gl+glcosθ=12v2
From the centripetal force requirement when the tension is zero, we have:
mgcosθ=mv2l
Cancelling m gives:
gcosθ=v2l
Thus,
v2=glcosθ
Substituting v2 back into the energy equation:
12gl+glcosθ=12(glcosθ)
This leads to:
12gl+glcosθ=12glcosθ
Rearranging gives:
12gl=12glcosθ−glcosθ
12gl=−12glcosθ
1=−cosθ
This gives:
cosθ=13
The angle θ is given by:
θ=cos−1(13)
The angle rotated before the string becomes slack is:
Angle rotated=π−θ=π−cos−1(13)
Thus, the angle rotated by the string before it becomes slack is:
π−cos−1(13)
Topper's Solved these Questions
Similar Questions
The bob of a simple pendulum is given a sharp hit impart it a horizontal speed of √3gL. Find an angle made by the string with the upper verticle before it becomes slack. Also, calculate the maximum height attained by the bob above the point of suspension. L is length of the string.
View SolutionThe bobo of a pendulum at rest is given a sharp hit to impart a horizontal velocity u where l is the length of the pendulum. Find the angle rotated by the string before it becomes slack.
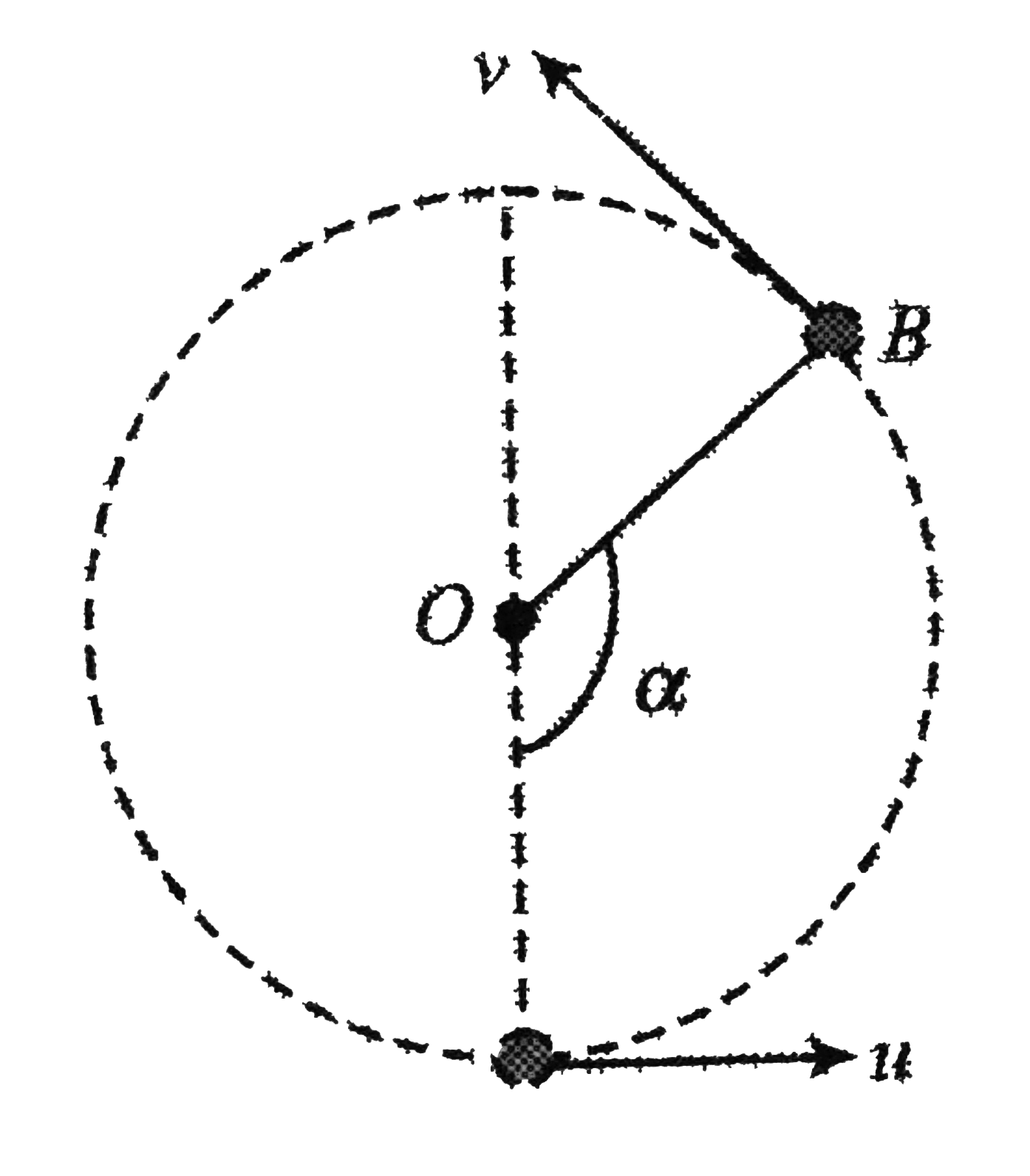
View Solution
Knowledge Check
A particle of mass m is attached to one end of a light inextensible string and the other end of the string is fixed in vertical plane as shown. Particle is given a horizontal velocity u=√52gl

The maximum angle made by the string with downward vertical isAcos−1(14)Bsin−1(14)Cπ2+cos−1(14)Dπ−cos−1(14)
The bob of a simple pendulum of length l is given a sharp hit to impart it a horizontal speed of √3gl . When it was at its lowermost position. Find
angle α shown of the string from upside of vertical and speed of the particle when the string becomes slack.
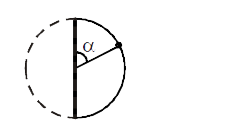
View SolutionThe bob of pendulum is project with horizontal velocity of √3gf I is the length of string. Find the angular displacement of string before it becomes slack.

View SolutionThe bob of a pendulum at rest is given a sharp hit to impat a horizontal velocity √10gl where l is the length of the pendulum. Find the tension in the string when a. the string is horizontal. B. The bob is at its highest point and c. the string makes an angle of 600 with teh upward vertical.
View SolutionA particle of mass m is suspended by a string of length l from a fixed rigid support. Particle is imparted a horizontal velocity u=√2gl. Find the angle made by the string with the vertical when the acceleration of the particle is inclined to the string by 45∘?
View SolutionA heavy particle is usspended by a 1.5 m long string . It is given a horizontal velocityof √57ms. a. Find the angle made by the string with the upward vertical, when it becomes slack. B. Find the speed of the particle at this instant. c.Find the maximum height reached by the particle over the point of suspension. Take g=10ms2
View SolutionA bob of mass m is suspended by a light inextensible string of length l from a fixed point such that it is free to rotate in a vertical plane. The bob is given' a speed of √4gl horizontally. The, height of the bob from the lower most point where the string just becomes slacked is nl3. Find n.
View SolutionBob of the simple pendulum is at rest at its lowest position , Sharp hit imparts a speed of √9gl to the pendium bob. Calculate tension of the thread when it makes an angle 60∘ with the vertical .
View Solution
