One end of a uniform rod of mas m and length l is clamped. The rod lies on a smooth horizontal surface and rotates on it about the clamped end at a unifrom angular velocity ω. Theforce exerted by the clamp on the rod has a horizontal component
mω2l
zero
mg
12mω2l
The correct Answer is:D
To solve the problem of finding the horizontal component of the force exerted by the clamp on a rotating uniform rod, we can follow these steps:
Step 1: Understand the System
We have a uniform rod of mass m and length l that is clamped at one end. The rod rotates about the clamped end with a uniform angular velocity ω. The goal is to determine the horizontal component of the force exerted by the clamp on the rod.
Hint: Visualize the rod and its rotation. Remember that each point along the rod is moving in a circular path about the clamped end.
Step 2: Identify Forces Acting on the Rod
The rod experiences a gravitational force acting downward (its weight) and a horizontal force due to the rotation. The clamp must exert a force to counteract the centrifugal effect caused by the rotation of the rod.
Hint: Consider the forces acting on a small segment of the rod to analyze the centrifugal effect.
Step 3: Calculate the Centrifugal Force on a Small Element
Consider a small element of the rod at a distance R from the clamped end with a small length dR. The mass of this small element dM can be expressed as:
dM=mldR
The centrifugal force dF acting on this small element due to its rotation is given by:
dF=dM⋅R⋅ω2=(mldR)⋅R⋅ω2
Hint: Use the formula for centrifugal force and remember that the force depends on the distance from the axis of rotation.
Step 4: Integrate to Find Total Horizontal Force
To find the total horizontal force F exerted by the clamp on the rod, integrate dF from R=0 to R=l:
F=∫l0dF=∫l0(mlRω2dR)
This simplifies to:
F=mω2l∫l0RdR
The integral ∫l0RdR evaluates to l22:
F=mω2l⋅l22=mlω22
Hint: Make sure to perform the integration correctly and apply the limits properly.
Step 5: Conclusion
The horizontal component of the force exerted by the clamp on the rod is:
F=mlω22
This means the correct option is mlω22.
Hint: Review the final expression to ensure it matches the physical context of the problem.
We have a uniform rod of mass m and length l that is clamped at one end. The rod rotates about the clamped end with a uniform angular velocity ω. The goal is to determine the horizontal component of the force exerted by the clamp on the rod.
The rod experiences a gravitational force acting downward (its weight) and a horizontal force due to the rotation. The clamp must exert a force to counteract the centrifugal effect caused by the rotation of the rod.
Consider a small element of the rod at a distance R from the clamped end with a small length dR. The mass of this small element dM can be expressed as:
dM=mldR
The centrifugal force dF acting on this small element due to its rotation is given by:
dF=dM⋅R⋅ω2=(mldR)⋅R⋅ω2
To find the total horizontal force F exerted by the clamp on the rod, integrate dF from R=0 to R=l:
F=∫l0dF=∫l0(mlRω2dR)
This simplifies to:
F=mω2l∫l0RdR
The integral ∫l0RdR evaluates to l22:
F=mω2l⋅l22=mlω22
The horizontal component of the force exerted by the clamp on the rod is:
F=mlω22
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective 2
15 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises
86 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Short Answer
22 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Exercises
51 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercises
58 Videos
Similar Questions
A uniform rod AB of mass m and length l is at rest on a smooth horizontal surface. An impulse J is applied to the end B perpendicular to the rod in horizontal direction. Speed of the point A of the rod after giving impulse is:
View SolutionA uniform of mass m and length L is tied to a vertical shaft. It rotates in horizontal plane about the vertical axis at angular velocity ω. How much horizontal force does the shaft exert on the rod?
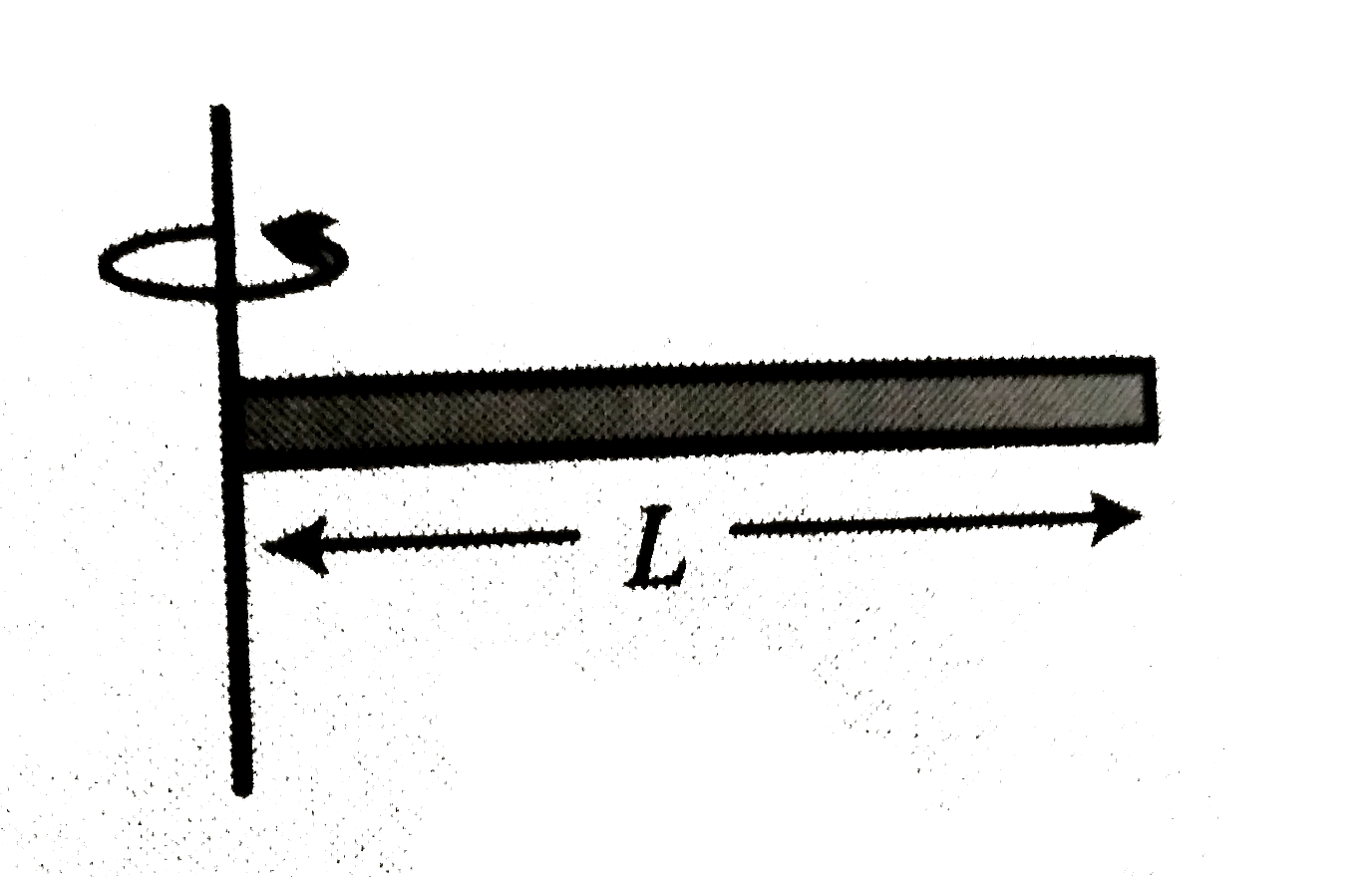
View Solution
Knowledge Check
A uniform rod of mass m and length l is at rest on smooth horizontal surface. An impulse P is applied to end B as shown.

Distance travelled by the centre of the rod, while rod rotates by 90∘Aπl7Bπl4Cπl12Dπl5A uniform rod of mass m and length L is at rest on a smooth horizontal surface. A ball of mass m, moving with velocity v0, hits the rod perpendicularly at its one end and sticks to it. The angular velocity of rod after collision is
 A6v05LB12v05LC2v05LD3v07L
A6v05LB12v05LC2v05LD3v07LA uniform rod of mass m, length l rests on a smooth horizontal surface. Rod is given a sharp horizontal impulse p perpendicular to the rod at a distance l/4 from the centre. The angular velocity of the rod will be
A3pmlBpmlCp2mlD2pml
A rod of length l is released from the vertical position on a smooth horizontal plane. What is the nature of motion of the free end of the rod ?
View SolutionA uniform rod of mass m and length l is on the smooth horizontal surface. When a constant force F is applied at one end of the rod for a small time t as shown in the figure. Find the angular velocity of the rod about its centre of mass.
View SolutionA uniform thin rod of mass m and length l is standing on a smooth horizontal surface. A slight disturbance causes the lower end to slip on the smooth surface and the rod starts falling. Find the velocity of centre of mass of the rod at the instant when it makes an angle θ with horizontal.
View SolutionA uniform rod of mass m and length l is at rest on smooth horizontal surface. An impulse P is applied to end B as shown.
The point about which rod rotates just after impulse being applied is located at
View SolutionA uniform metallic rod lies on a smooth horizontal surface. C is centre of mass of rod and A is a point on the rod. As the temperature increase
View Solution
