Let I1andI2 be the moments of inertia of two bodies of identical geometrical shapek, the first made of aluminium and the second of iron.
I1<IB
I1=I2
I1>I2
relation between I_1 and I_2 depends on the actual shapes of the bodies
The correct Answer is:A
Step by step video, text & image solution for Let I_1 and I_2 be the moments of inertia of two bodies of identical geometrical shapek, the first made of aluminium and the second of iron. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective 2
15 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises
86 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Short Answer
22 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Exercises
51 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercises
58 Videos
Similar Questions
If I1,I2 ,and I3 are moments of inertia of a disc about its geometric axis,diameter and a tangent in its plane,then
View SolutionTwo rods OA and OB of equal length and madd are lying on xy plane as shown in figure . Let Ix,IyandIz be the moment of inertias of both the rods about x,y and z axis respectively . Then moment of inertias of the combined rod system is
View Solution
Knowledge Check
Statement - 1 : Let I1andI2 be the moment of inertia of two bodies of identical geometrical shapes, the first made of aluminium and second of iron then I1<I2. Becaause
Statement - 2 : Moment of inertia does not depends on shapeAStatement - 1 is true, statement - 2 is true, statement - 2 is correct explanation for statement - 1BStatement - 1 is true, Statement - 2 is true, Statement - 2 is not a correct explanation for statement - 1Cstatement - 1 is true, statement - 2 is falseDstatement - 1 is false, statement - 2 is true.The moment of inertia of a ring about its geometrical axis is I, then its moment of inertia about its diameter will be
A1 IBI/2CIDI/4I1,I2 are moments of inertia of two solid spheres of same mass about axes passing through their centres. If first is made of wood and the second is made of steel, then
AI1=I2BI1tI2CI1>I2DI1≤I2
A solid sphere of mass M and radius R is divided into two unequal parts. The first parts has a mass of 7M8 and is converted into a uniform disc of radius 2R. The second part is converted into a uniform solid sphere. Let I1 be the moment of inertia of the uniform disc about its axis and I2 be the moment of inertia of the sphere made from remaining part about its axis. The ratio I1/I2 is 140x. Find the value of x.
View Solution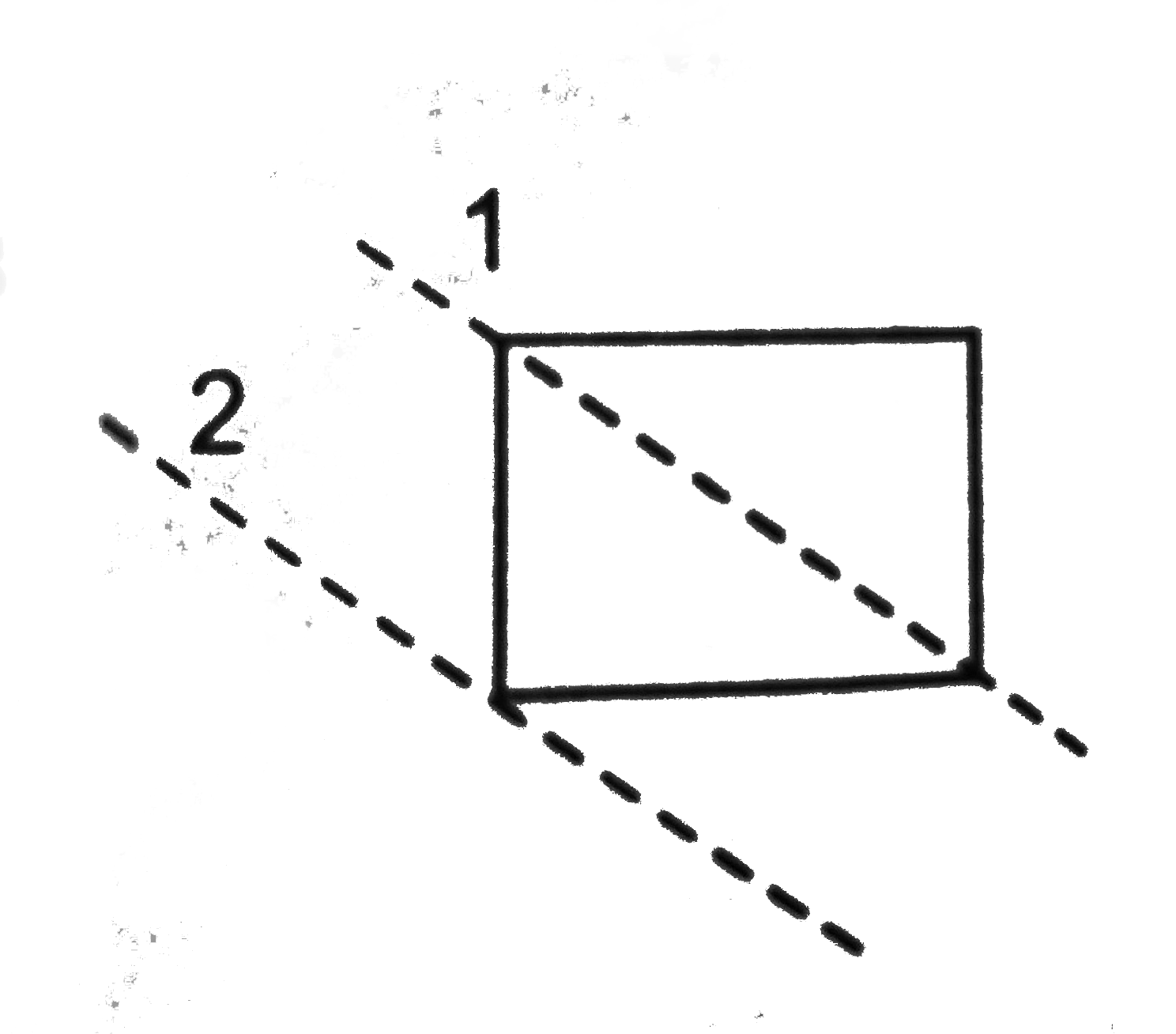
Let I1 and I2 be the moment of inertia of a uniform square plate about axes shown in the figure. Then the ratio I1:I2 is
View SolutionLet I1 and I2 be the moment of inertia of a uniform square plate about axes APC and OPO respectively as shown in the figure.P is centre of square. The ratio I1I2 moment of inertia is -
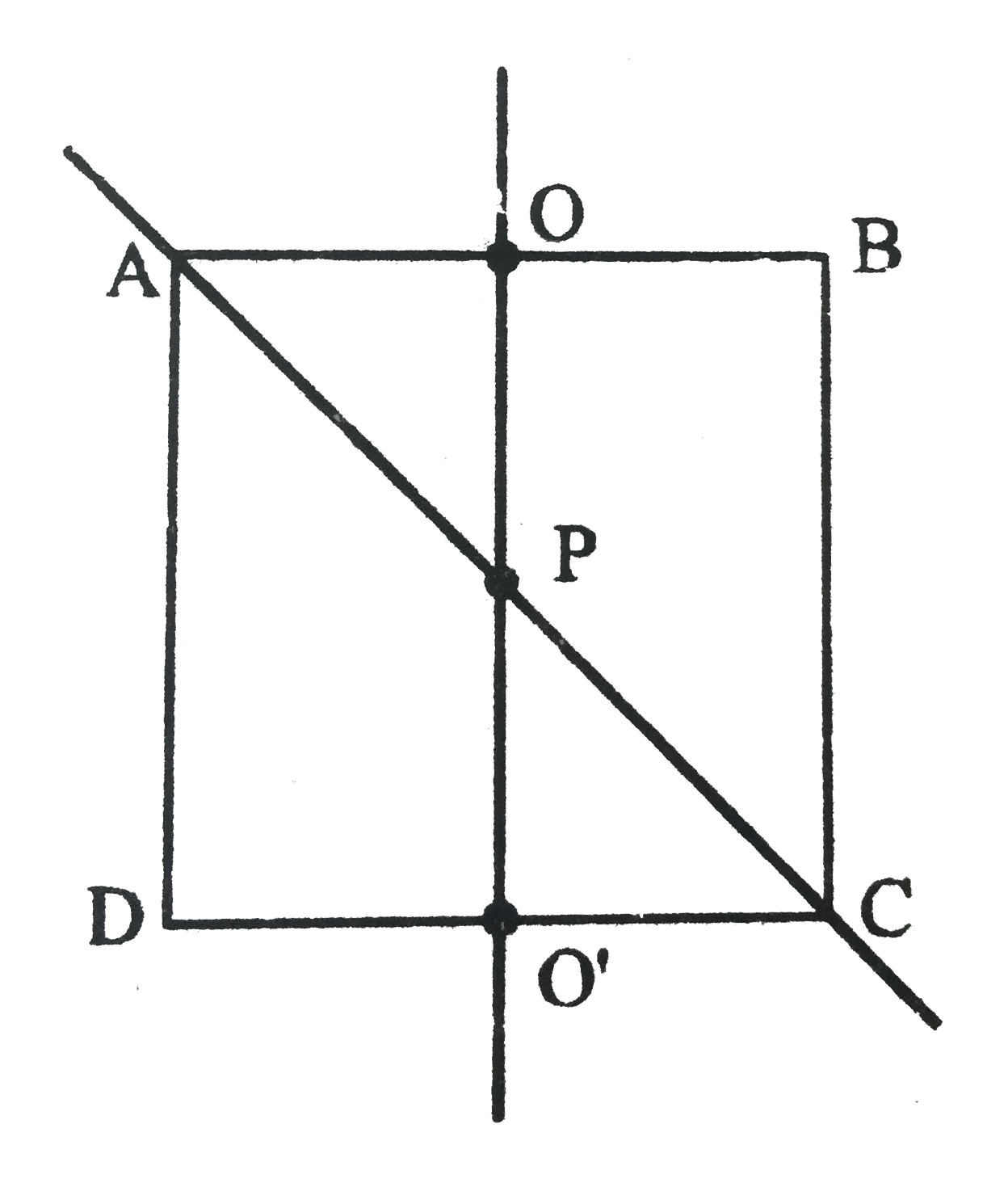 .
.
View SolutionA rigid body is rotating about a vertical axis. In t second, the axis gradually becomes horizontal. But the rigid body continues to make v rotations per second throughout the time interval of 1 second. If the moment of inertia I of the body about the axis of rotation can he taken as constant, then the torque acting on the body is
View SolutionLet IA and IB be moments of inertia of a body about two axes A and B respectively. The axis A passes through the centre of mass of the body but B does not
View Solution
