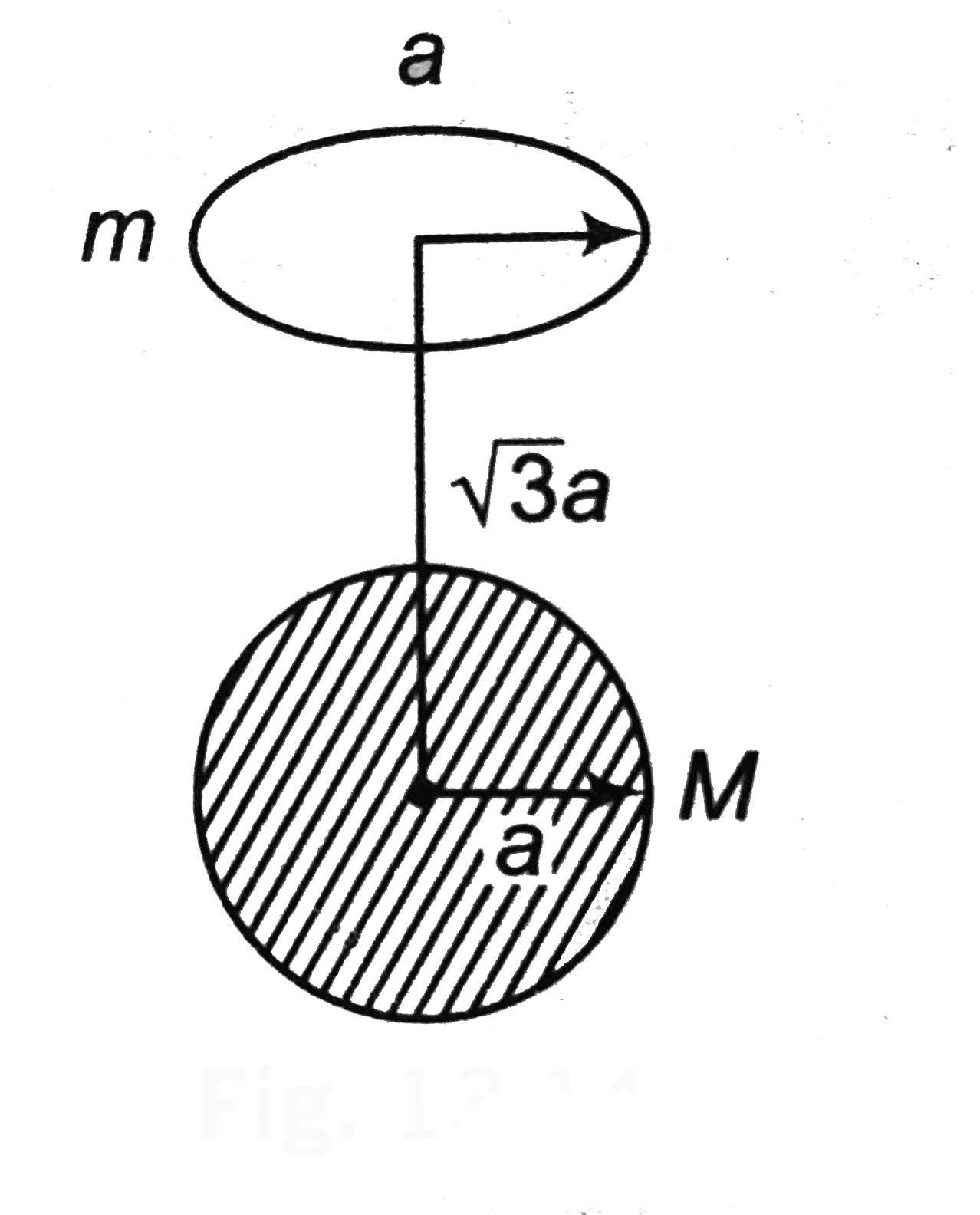
A uniform rig of mas m and radius a is placed directly above a uniform sphre of mass M and of equyal radius. The centre of the ring is at a distance $\sqrt{3}$ a from the centre of the sphere. Find the gravitational foerce exerted by the sphere on the ring.

Video Solution

Text Solution

Verified by Experts

The gravitatioN/Al field at any on the ring due to the sphere is equal to the field due to a isngle particle of mass M laced at the centre of the sphre.Thus, theforce on the ring due to the sphere is also equla to the force on it by a particle of mas M placed at this point.By Newton's third laaw it is equal to the force on the particle by the rig. Now the gravitatioN/Al field due to the ring at a distance $d = \sqrt{3}$ a on its axis is
$E = \frac{G m d}{{(a^{2} + d^{2})}^{\frac{3}{2}}}$ =(sqrt3Gm)/(8a^2) $< b r > < i m g a \leq ocr_image l o a d \in g = lazy s r c = https://d10lpgp6xz60nq.cloudfront.net/physics_images/HCV_VOL1_C11_S01_018_S01.png w h = 80% mx-auto s t y \leq = background:white; > < b r > T h e f or c e o n a a r t i c \leq o f m a s s s M p l a c e d h e r e i s < b r >$ F=ME $< b r >$ =(sqrt3GMm)/(8a^2)
This is also the force due to the sphere on the ring

|

Updated on:21/07/2023

Class 11 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A uniform ring of mass M and radius R is placed directly above a uniform sphere of mass 8M and of same radius R. The centre of the ring is at a distance of $d = \sqrt{3} R$ from the centre of the sphere. The gravitational attraction between the sphere and the ring is
A $\frac{G M^{2}}{R^{2}}$
B $\frac{3 G M^{2}}{2 R^{3}}$
C $\frac{2 G M^{2}}{\sqrt{2} R^{2}}$
D $\frac{\sqrt{3} G M^{2}}{R^{2}}$
Question 2 - Select One
A uniform ring of mass M and radius R is placed directly above a uniform sphere of mass 8M and of same radius R. The centre of the ring is at a distance of $d = \sqrt{3} R$ from the centre of the sphere. The gravitational attraction between the sphere and the ring is
A $\frac{G M^{2}}{R^{2}}$
B $\frac{3 G M^{2}}{R^{3}}$
C $\frac{2 G M^{2}}{\sqrt{2} R^{2}}$
D $\frac{\sqrt{3} G M^{2}}{R^{2}}$
Question 3 - Select One
A uiform ring of mass M and radius R is placed directly above a uniform sphere of mass 8M and same radius R. The centre of the sphere. The gravitational atraction between the sphere and the ring is
A $\frac{8 G M^{2}}{R^{2}}$
B $\frac{2 G M^{2}}{\sqrt{3} R^{2}}$
C $\frac{3 G M^{2}}{2 R^{2}}$
D $\frac{\sqrt{3}}{2} \frac{G M^{2}}{R^{2}}$

HC VERMA-GRAVITATION-Worked Out Examples

Home
Ask Doubt
Profile