In figure the upper wire is made of steel and the lower of copper. The wires have equal cross section. Find the ratio of the longitudinals strains developed in the two wires.

The correct Answer is:A, C, D
→(ΔLLsteel)FAYSteel
→((ΔL)/Lcu)=FAYcu

Strain in steel wire /Strain in copper wire=FAYSteel+AYcuF
(Acu)=ASteel
YcuYSteel=1320=0.65
Topper's Solved these Questions
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMACHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Objective 2
7 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercises
58 Videos
- SOUND WAVESBOOK - HC VERMACHAPTER - SOUND WAVESEXERCISE - Exercises
89 Videos
Similar Questions
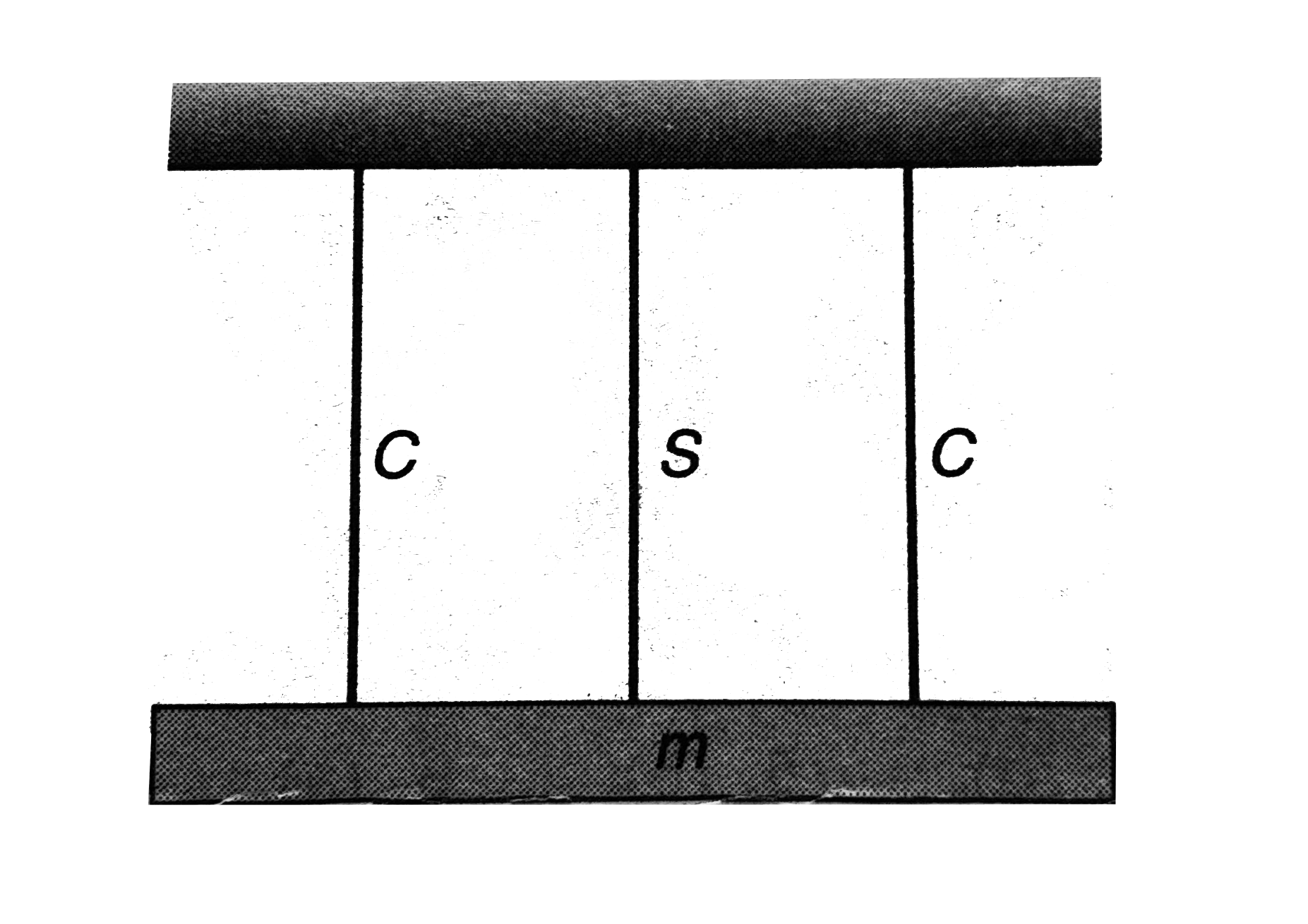
A homogeneous block with a mass m hangs on three vertical wires of equal length arranged symmetrically . Find the tensions of the wires if the middle wire is of steel and the other two are of copper . All the wires have the same cross - section . Consider the modulus of elasticity of steel to be double than that of copper .
View SolutionTwo wires of equal cross section but one made of steel and the other of copper, are joined end to end. When the combination is kept under tension, the elongations in the two wires are found to be equal. Find the ratio of the lengths of the two wires. Young modulus of steel =2.0×1011Nm−2 and that of copper =1.1×101Nm−2
View Solution
Knowledge Check
The ratio of lateral strain to the longitudinal strain of a wire is called
AcompressibilityBmodulus of rigidityCtensile strengthDPoisson's ratioIn the question number 55, the ratio of elongation of steel to the copper wires is
A52B25C32D23Two wires of equal cross-section but one made of steel and the other of copper are joined end to end. When the cobination is kept under tension, the elongations in the two wires are found to be equal elongations in the two wire are found to be equal. What is the ratio of the lengths of the two wires?
(Given, Young's modulus of steel =2×1011Nm−2 and young's modulus of copper =1.1×1011Nm−2)A2:11B11:2C20:11D11:20
A steel wire having cross-sectional area 2 mm^(2) is stretched by 20 N. Find the lateral strain produced in the wire Y_("steel") = 2 xx 10^(11) Nm^(-2), sigma = 0.391
View SolutionA steel wire and a copper wire of equal length and equal cross sectionasl area area joined end to end and the combination is subjected to as tension. Find the ratio of as. The stresses developed in the two wire and b. the strains developed. Y of steel =2×1011Nm−2. Y o copper =1.3×1011Nm−2.
View SolutionIn the above question the ratio of the elongation produced in the copper wire and steel wire are
View SolutionWith the reference of above problem, the ratio of the strains in the two wires is
View SolutionTwo copper wires A and B of length l and 2l respectively, have the same area of cross-section. The ratio of the resistivity of wire A to the resistivity of wire B is
View Solution
