Consider the wave y=(5mm)sin[(1cm−1)x−(60s−1)t]. Find the amplitude, b. the wave number, c the wavelenth d. the frequencey e. the time period and f. the wave velocity.
Comparing the given equation with equation (15.15, we find
a amplitude A=5mm
b. Wave number k=1cm−1
c. wavelength λ=2πk=2πcm
d=equencyv=ω2π602πHz
=30πHz
e. time period T=1v=π30s
f.wavevelocityu=vλ=60cms−1
Topper's Solved these Questions
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMACHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Worked Out Examples
10 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMACHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Short Answer
7 Videos
- THE FORCESBOOK - HC VERMACHAPTER - THE FORCESEXERCISE - Exercises
12 Videos
- WORK AND ENERGYBOOK - HC VERMACHAPTER - WORK AND ENERGYEXERCISE - Exercises
64 Videos
Similar Questions
Consider the wave y=(10mm)sin[(5πcm−1)x−(60πs−1)t+π4]. Find (a) the amplitude (b) the wave number (c) the wavelength (d) the frequency f the time period and (f) the wave velcity (g) phase constant of SHM of particle at x=0.
View SolutionConsider the wave y=(5mm)sin[1cm−1x−(60s−1)t]. Find (a) the ampitude, (b) the angular wave number, ( c ) the wavelength, (d) the frequency, (e) the time period and (f) the wave velocity.
View SolutionThe equation of a wave travelling on a string is y=(0.10mm)sin[3.14m−1)x+(314s−1)t]. (a) In which direction does the wave travel ? (b) Find the wave speed, the wavelength and the frequency of the wave. (c) What is the maximum displacement and the maximum speed of a portion of the string ?
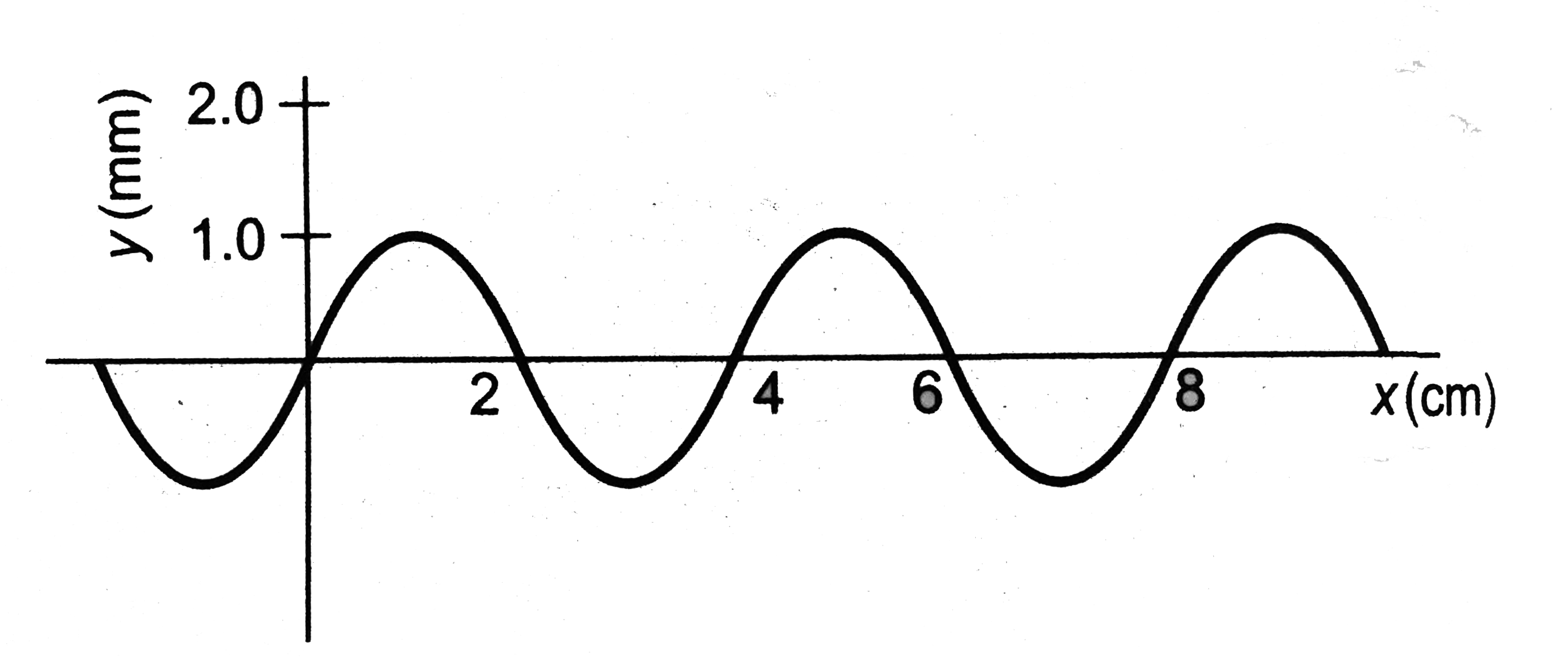
View SolutionFigure shows a plot of the transverse displacements of the particles of a string at t = 0 through which a travelling wave is passing in the positive x-direction. The wave speed is 20cms−1. Find (a) the amplitude, (b) the wavelength, (c) the wave number and (d) the frequency of the wave.
 .
.
View SolutionThe equation of a wave travelling on a string is y=(0.10mm)sin[(31.4m−1)x+(314s−1)t]
(a) In which direction does the travel?
(b) Find the wave speed, the wavelength and the frequency of the wave.
( c ) What is the maximum displacement and the maximum speed of a portion of the string?
View SolutionFigure shows a plot of the transverse displacement of the particle of a string at t=0 through which a travelling wave is passing in the positive in the positive x-direction. The wave speed is 20cm/s. Find (a) the amplitude (b) the wavelength (c) the wave number and (d) the frequency of the wave.
View SolutionThe equation of a plane wave is
s=6.0×10−6cos(1900t+5.72x)
Find the frequency, the wavelenght and the velocity of the wave. Compare the wavelenght with the amplitude of the oscillations and the wave velocity with the amplitude of the velocity of the oscillations.
View SolutionThe equation of a progressive wave is y=1.5sin(328t−1.27x). Where y and x are in cm and t is in second. Calcualte the amplitude, frequency, time period and wavelength of the wave.
View SolutionThe pressure in a traveling sound wave is given by the equation
Δp=(2.00Pa)sinπ(0.900m−1)x−(450s−1)t]
Find the (a) pressure amplitude, (b) frequency, (c ) wave length, and (d) speed of the wave.
View SolutionA wave is decribed by the equation y = (1.0mm) sin pi ((x)/(2.0cm) - (t)/(0.1s)). (a) Find the time period and the wavelength ? (b) Write the equation for the velocity of the particles. Find the speed of the particle at x = 1.0cm at time t = 0.01s, (c ) What are the speeds of the particles at x =3.0 cm 5.0 cm aned 7.0cm at t = 0.01 ? (d) What are the speeds of the particles at x = 1.0 cm at t= 0.011, 0.012, and 0.013s ?
View Solution
