A string of length 40 cm and weighing 10 g is attached to a spring at one end and to a fixed wall at the other end. The spring has a spring constant of 160Nm−1 and is stretched by 1-0 cm. If a wave pulse is produced on the string near the wall, how much time will it take to reach the spring ?
To solve the problem, we need to determine how long it takes for a wave pulse to travel along a string from the wall to the spring. We will follow these steps:
Step 1: Convert the given measurements to standard units
- Length of the string (L) = 40 cm = 0.4 m
- Mass of the string (m) = 10 g = 0.01 kg
- Spring constant (k) = 160 N/m
- Stretch of the spring (x) = 1 cm = 0.01 m
Step 2: Calculate the linear mass density (μ) of the string
The linear mass density (μ) is given by the formula:
μ=mL
Substituting the values:
μ=0.01kg0.4m=0.025kg/m
Step 3: Calculate the tension (T) in the string
The tension in the string due to the spring can be calculated using Hooke's Law:
T=k⋅x
Substituting the values:
T=160N/m×0.01m=1.6N
Step 4: Calculate the velocity (v) of the wave on the string
The velocity of the wave on the string can be calculated using the formula:
v=√Tμ
Substituting the values:
v=√1.6N0.025kg/m=√64=8m/s
Step 5: Calculate the time (T) it takes for the wave to travel the length of the string
The time taken for the wave to travel the length of the string can be calculated using the formula:
T=Lv
Substituting the values:
T=0.4m8m/s=0.05s
Final Answer
The time it takes for the wave pulse to reach the spring is 0.05 seconds.
---
- Length of the string (L) = 40 cm = 0.4 m
- Mass of the string (m) = 10 g = 0.01 kg
- Spring constant (k) = 160 N/m
- Stretch of the spring (x) = 1 cm = 0.01 m
The linear mass density (μ) is given by the formula:
μ=mL
Substituting the values:
μ=0.01kg0.4m=0.025kg/m
The tension in the string due to the spring can be calculated using Hooke's Law:
T=k⋅x
Substituting the values:
T=160N/m×0.01m=1.6N
The velocity of the wave on the string can be calculated using the formula:
v=√Tμ
Substituting the values:
v=√1.6N0.025kg/m=√64=8m/s
The time taken for the wave to travel the length of the string can be calculated using the formula:
T=Lv
Substituting the values:
T=0.4m8m/s=0.05s
The time it takes for the wave pulse to reach the spring is 0.05 seconds.
Topper's Solved these Questions
Similar Questions
A string of linear mass density 0.5gcm−1 and a total length 30 cm is tied to a fixed wall at one end and to a frictionless ring at the other end. The ring can move on a vertical rod. A wave pulse is produced on the string which moves towards the ring at a speed of 20cms−1. The pulse is symmetric about its maximum which is located at a distance of 20 cm from the end joined to the ring. (a) Assuming that the wave is reflected from the ends without loss of energy, find the time taken by the string to regain its shape. (b) The shape of the string changes periodically with time. Find this time period. (c) What is the tension in the string ?

View SolutionA rubber cord of force constant k = 100 N/m and l=1m is attached to a particle of mass m = 1kg at one end and fixed to a vertical wall at the other. The body is displaced by x0 = 25cm so as to stretch the cord and then released. Calculate the time particle takes to reach the wall.
View Solution
Knowledge Check
A string of length 10.0 m and mass 1.25kg stretched with a tension of 50N. If a transverse pulse is created at one end of the string, how long does it take to reach the other end ?
A0.5sB1.0sC1.5sD2.0sA spring of spring constant 5×102Nm is streched initially by 5cm from the unstriched position . Then the work required to streach is further by another 5cm is
A12.50 NmB18.75 NmC25.00 NmD6.25 NmA spring of spring constant 5×103N/m is stretched initially by 5 cm from the upstretched position. The work required to further stretch the spring by another 5 cm is .
A6.25N−mB1250N−mC18.75N−mD25.00N−m
A spring has spring constant k=200N/m. How much work does it take to stetch the spring
10 cm from equilibrium ?
View SolutionOne end of a spring of force constant k1 is attached to the ceiling of an elevator. A block of mass 1.5kg is attached to the other end. Another spring of force constant k2 is attached to the bottom of the mass and to the floor of the elevator as shown in figure. At equilibrium, the deformation in both the spring is equal and is 40cm. If the elevator moves with constant acceleration upward, the additional deformation in both the spring is 8cm. Find the elevator's accelerationn ( g=10ms−2).
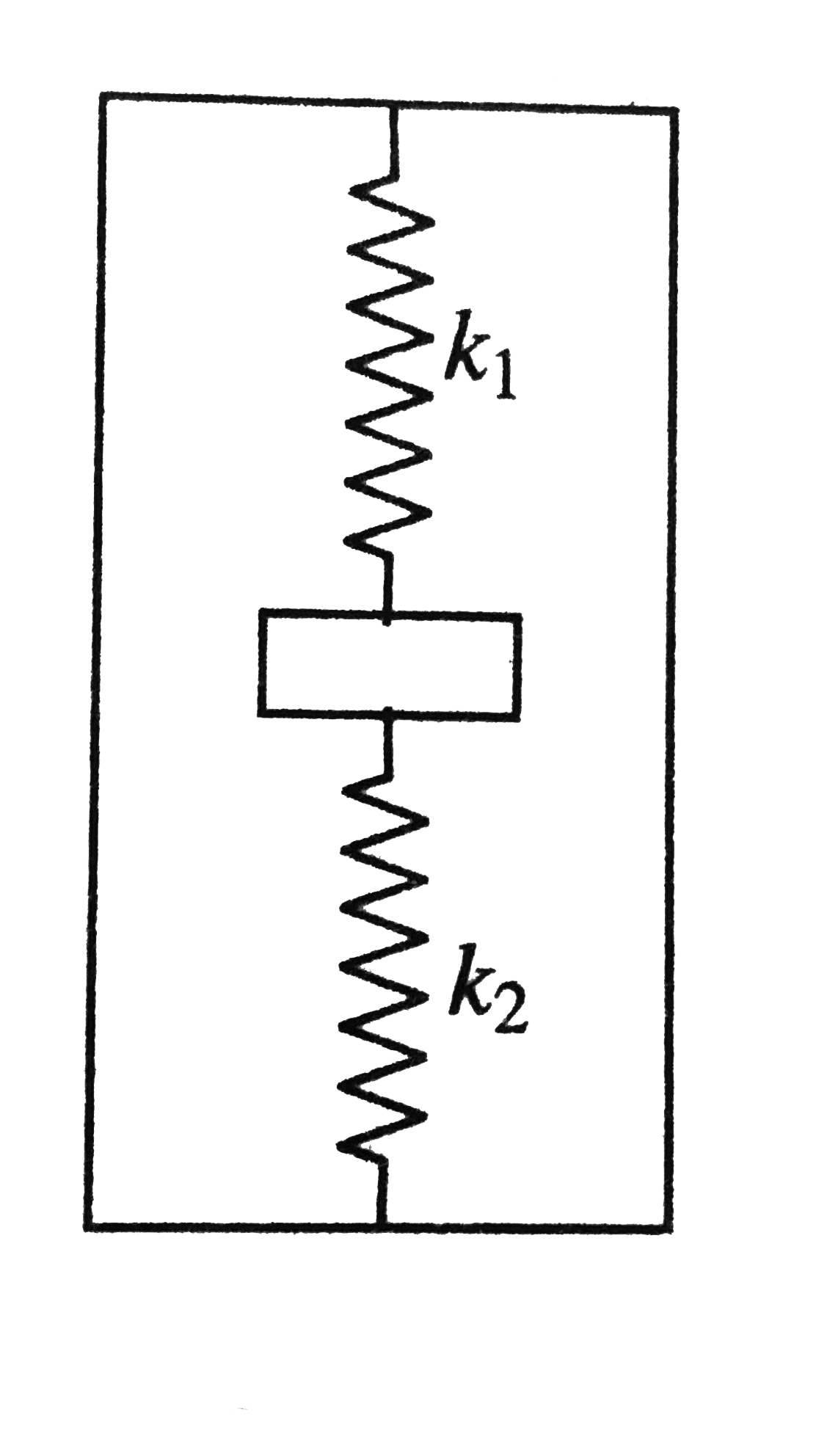
View SolutionA spring of spring constant 5×103.Nm−1 is stretched initially by 5 cm from the unstretched position. The work required to stretch it further by another 5 cm is
View SolutionThere are two identical springs, each of spring constant 240 N/m, one of them is compressed by 10cm and the other is stretched by 10 cm. What is the difference in the potential energies stored in the two springs ?
View SolutionA spring of spring constant 5×102Nm is streched initially by 5cm from the unstriched position . Then the work required to streach is further by another 5cm is
View Solution
