m men and w women seat themselves at random on m + w seats arranged in row (circle). If p1(p2) denote the probability of all women sitting together when they are arranged in row (circle), then a) p1=m+1C(m+w,m) b)p1+p2=2m+w+1C(m+w,m) c) p1=p2 if n only if w=1 d) p2<p1 if w>1
Step by step video & image solution for m men and w women seat themselves at random on m + w seats arranged in row (circle). If p_1 (p_2) denote the probability of all women sitting together when they are arranged in row (circle), then a) p_1 =(m+1)/(C(m+w,m)) b)p_1+p2=(2m+w+1)/(C(m+w,m)) c) p_1=p_2 if n only if w=1 d) p_2 lt p_1 if w gt 1 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
m men and w women are to be seated in a row so that no two women sit together.If m>w then the number of ways in which they can be seated is
View Solutionm men and n women are to be seated in a row so that no two women sit together. If (m>n) then show that the number of ways in which they can be seated as m!(m+1)!(m−n+1)! .
View Solution
Knowledge Check
m men and w women are to be seated in a row so that all women sit together. The number of ways in which they can be seated is
A(m+1)!w!Bm!w!Cm!(w−1)!Dm+wCwm men and w women are to be seated in a row so that no two women sit together. If m>w, then the number of ways in which they can be seated is :
Am!(m+1)!(m−w+1)!BmCm−w(m−w)!Cm+wCm(m−w)!Dnone of these
m men and n women are to be seated in a row so that no two women sit together. If (m>n) then show that the number of ways in which they can be seated as m!(m+1)!(m−n+1)! .
View SolutionThere is a planet P mass 2M and mass of earth M density of earth to density of planet. Body weight in earth is W. Then the weight of object on planet P is
View SolutionB={p:p∈W and p2<20}
View SolutionIf l||m||n and p is a transversal and ∠1=110∘ then find values of x,y,z,w
View SolutionIf the equations y=mx+c and xcosα+ysinα=p represent the same straight line, then (a)p=c√1+m2 (b) c=p√1+m2 (c)cp=√1+m2 (d) p2+c2+m2=1
View Solution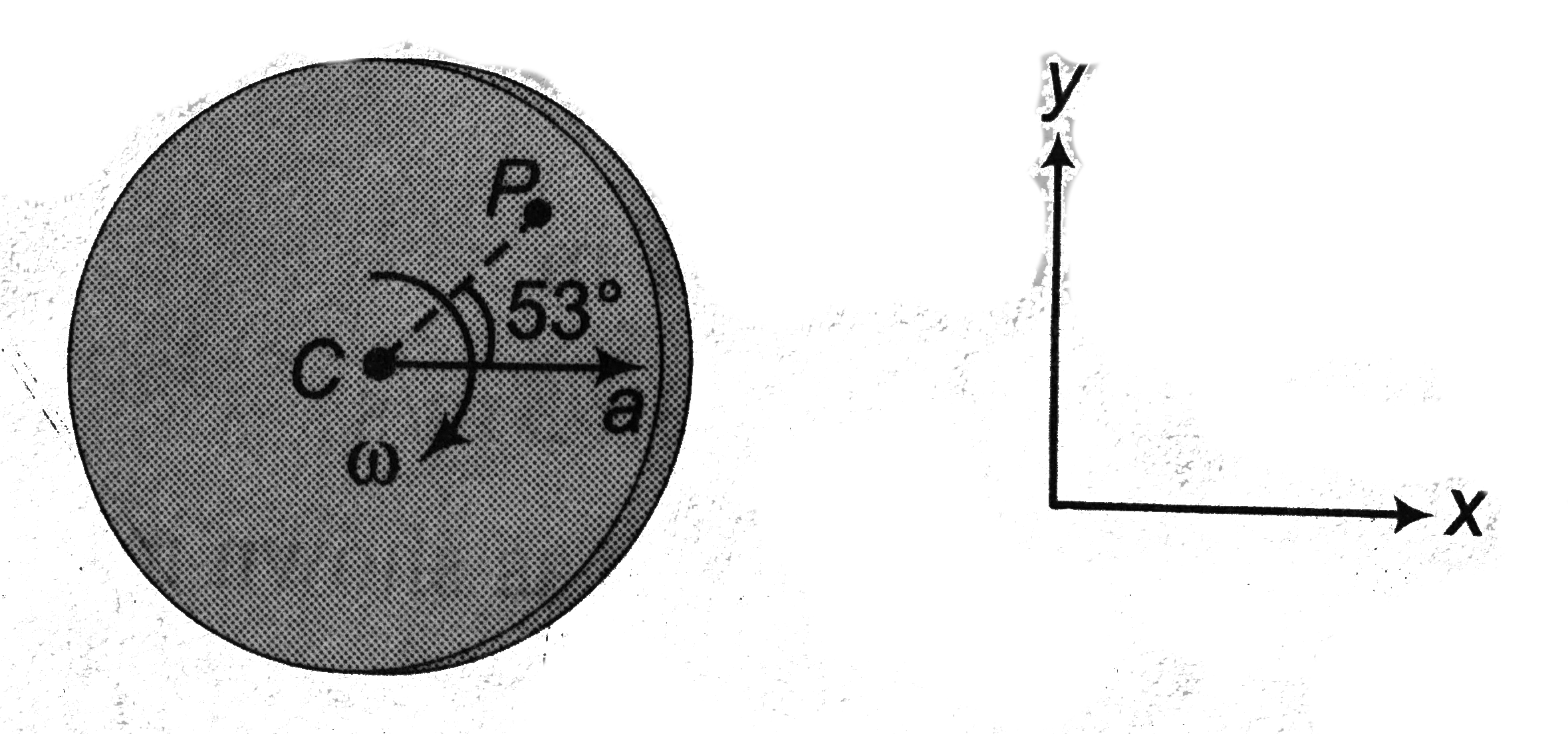
In the shown figure,
a=2m/s2,ω=(2t)rods−1 and CP=1m
In terms of ˆi and ˆj, find linear acceleration of the particle at P at P at t=1 s
View Solution
