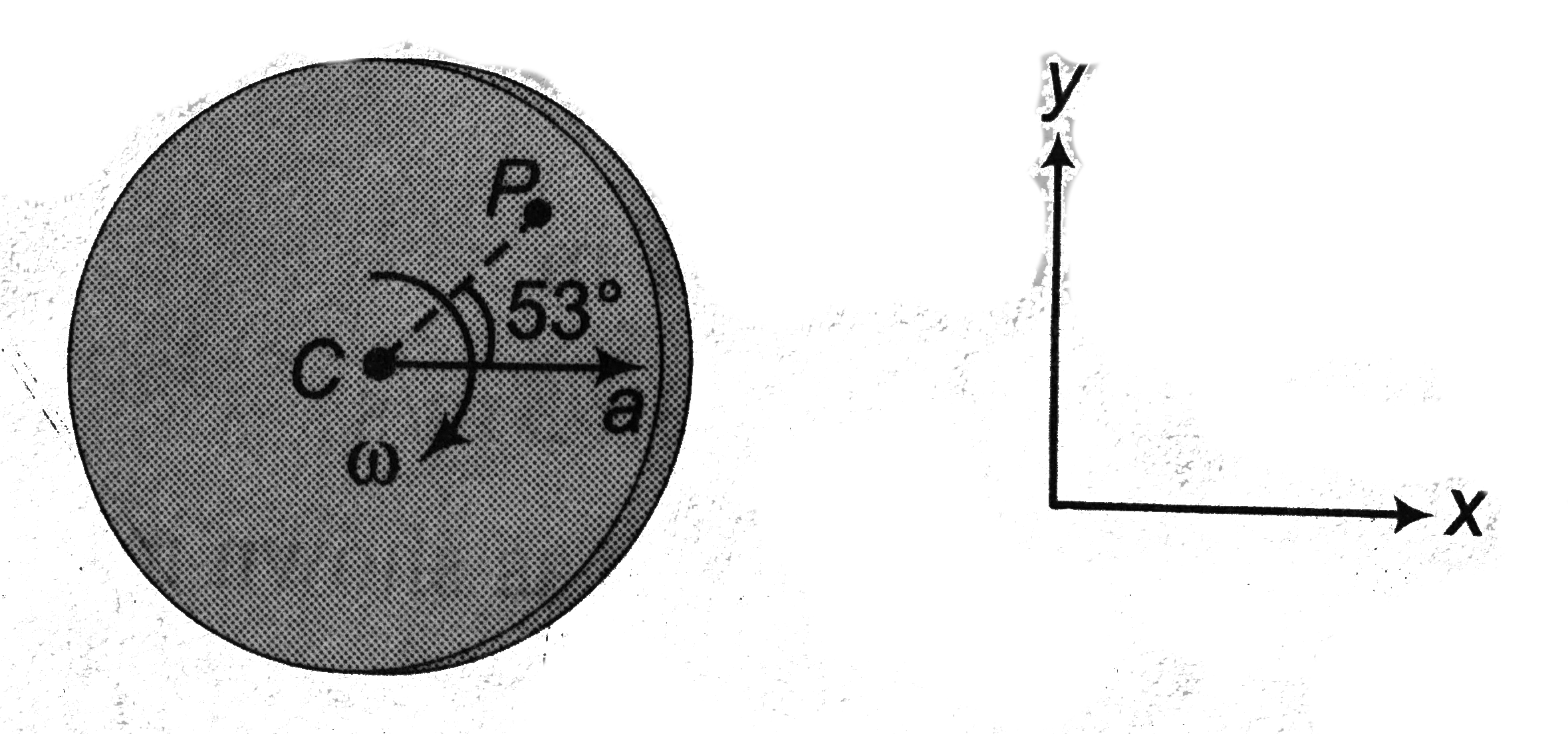
In the shown figure,
a=2m/s2,ω=(2t)rods−1 and CP=1m
In terms of ˆi and ˆj, find linear acceleration of the particle at P at P at t=1 s
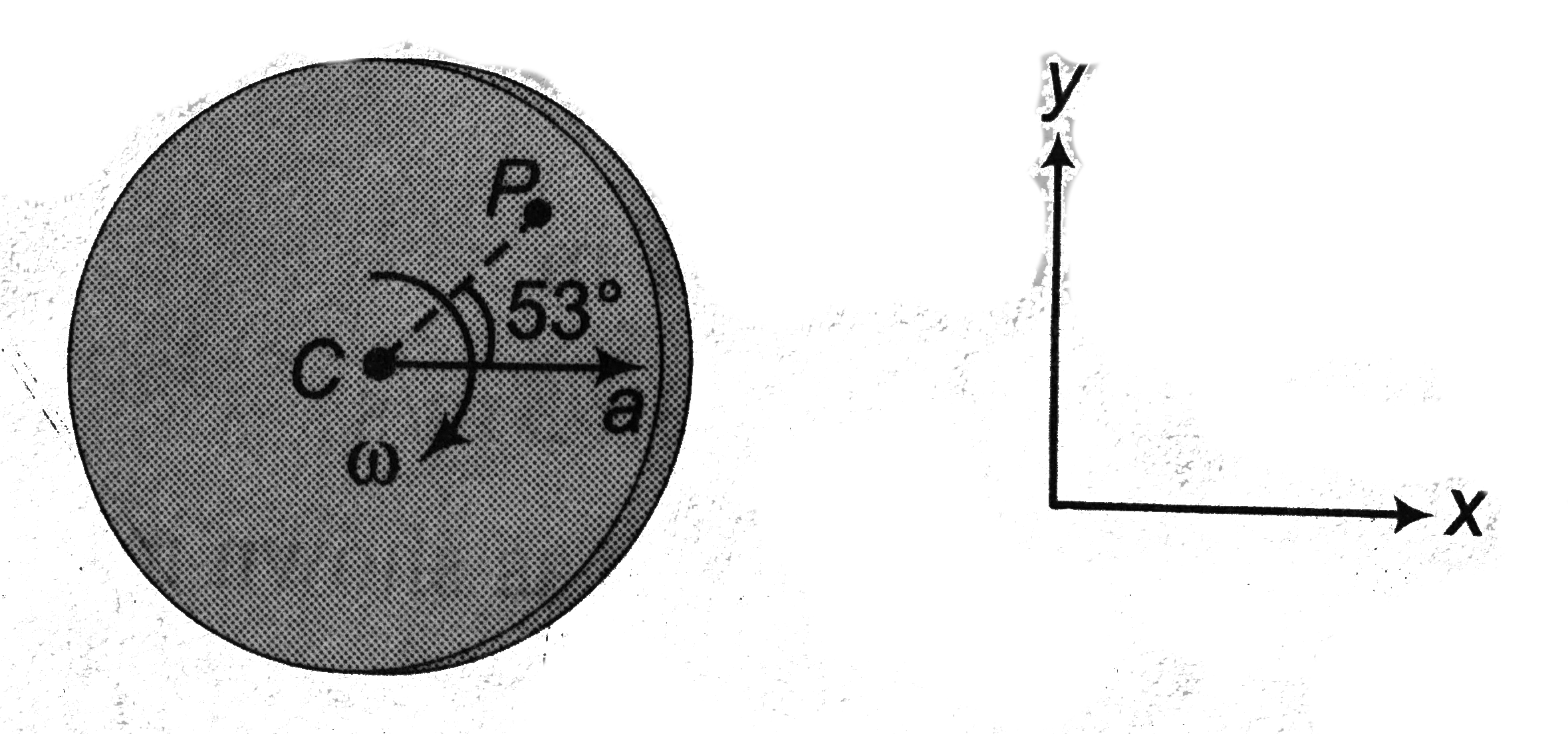 For particle at P
For particle at P
r=CP=1m
⇒α=dωdt=ddt(2t)=2rad/s2
At t=1 s
ω=2rad/s
α=2rad/s2
at=rα=2m/s2
ar=rω2=4m/s2
and a=2m/s2
Net acceleration of P is the vector sum of three terms a, ar and at as shown in figure below.
∴aP=2ˆi+(2cos37∘ˆi−2sin37∘ˆj)+(−4sin37∘ˆi−2sin37∘ˆj)−(−4sin37∘ˆi−4cos37∘ˆj)
=2ˆi+1.6ˆi−1.2ˆj−2.4ˆi−3.2ˆj
=(1.2ˆi−4.4ˆj)m/s2
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Solved Examples25videos
ROTATIONAL MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Miscellaneous Examples2videos
ROTATIONAL MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises39videos
ROTATION
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions17videos
ROTATIONAL MOTION
Similar Questions

In the figure shown υ=2m/s ω=5rad/s and CP=1m
In terms of ˆi and ˆj find linear velocity of particle P.View Solution
In the figure shown omega = v/2R in terms of i and j find the linear velocities of particles M,N,R and S .View Solution
A circular disc is rotating with an angular speed (in radian per sec)
ω=2t2
given, CP=2m
In terms of ˆi,ˆj and ˆk at t=1s
find,
(a). ω
(b). α
(c). linear velocity of the particle lying at P (d). linear acceleration of the particle lying PView Solutionthe angular velocity omega of a particle varies with time t as ω=5t2+25rads. the angular acceleration of the particle att=1s is
View SolutionIn the figure shown, find acceleration of the system and tenslons T1,T2andT3(Take=10m/s2)
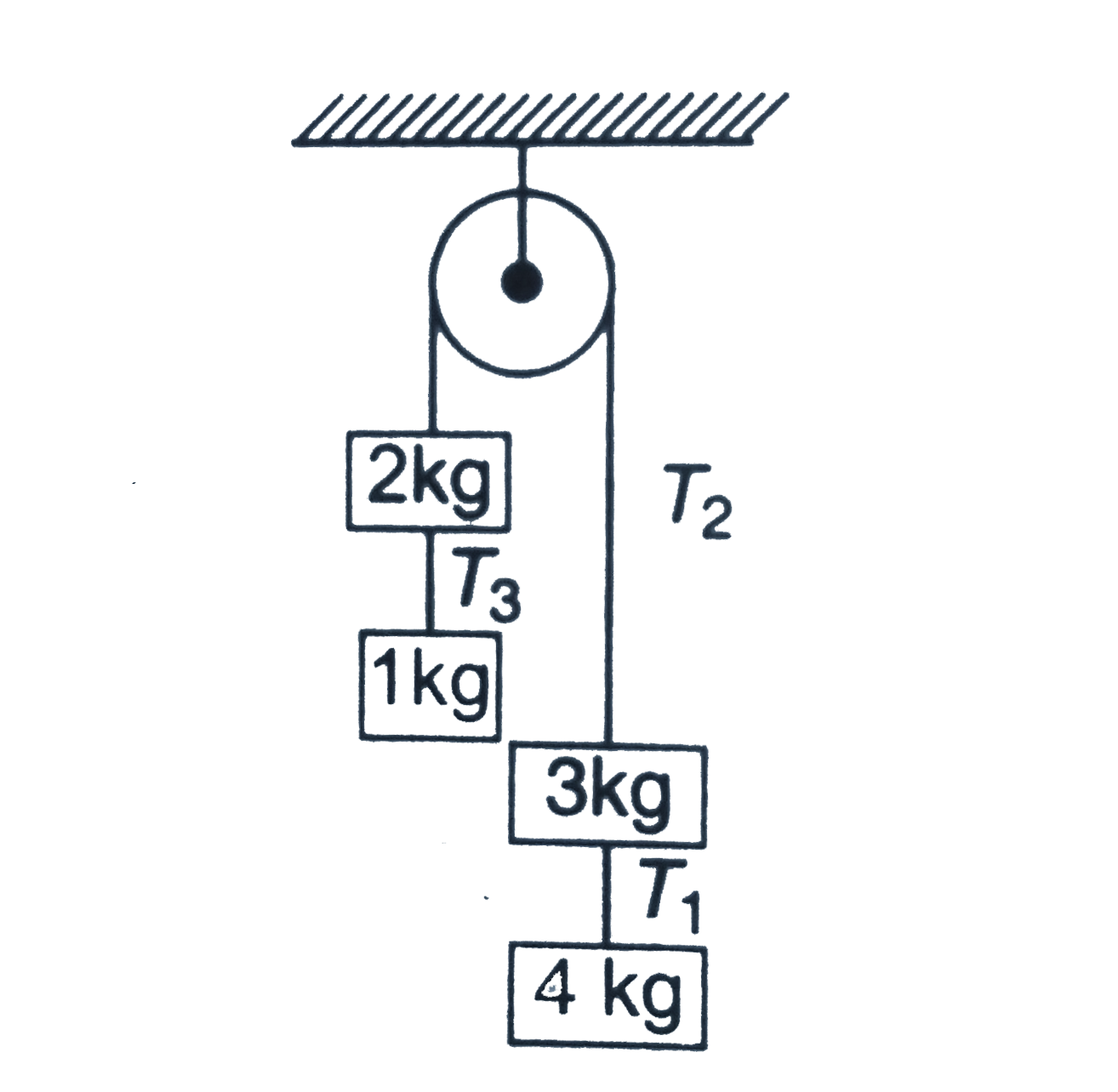 View Solution
View SolutionVelocity of a particle in x-y plane at any time t is v=(2tˆi+3t2ˆj)m/s At t=0, particle starts from the co-ordinates (2m,4m). Find
(a) acceleration of the particle at t=1s.
(b) position vector and co-ordinates of the particle at t=2s.View SolutionIn the same figure. If v and ω both are constant, then find linear acceleration of point M,N,R and S in terms of R,ω,ˆi and ˆj where R is the radius disc.
View SolutionA particle moves in circle of radius 1.0 cm at a speed given by v=2.0 t where v is in cm/s and t in seconeds. A. find the radia accelerationof the particle at t=1 s. b. Findthe tangential acceleration at t=1s. c.Find the magnitude of the aceleration at t=1s.
View SolutionIn the figure shown,a3=6m/s2 (downwards) and a2=4m/s2 (upwards) .Find acceleration of 1
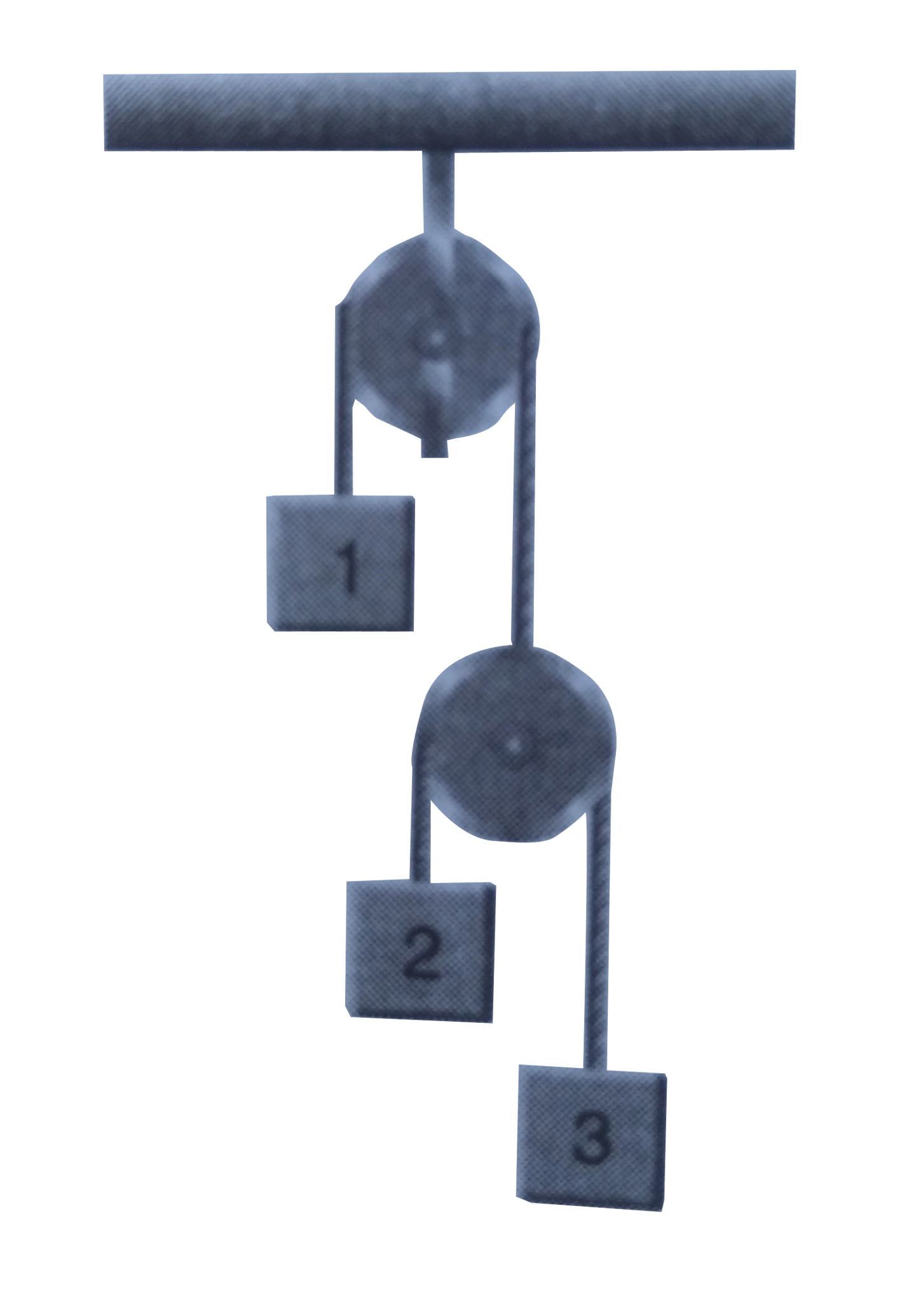 View Solution
View SolutionIn the figure shown,a3=6m/s2 (downwards) and a2=4m/s2 (upwards) .Find acceleration of 1
 View Solution
View Solution

