Question

$Z=30x+20y$ , $x+y\le 8,x+2y\ge 4,6x+4\ge 12,x\ge 0,y\ge 0$

Options

A. unique solution
B. infinitely many solution
C. minimum at (4, 0)
D. minimum 60 at point (0, 3)

Answer

B

Explanation

Feasible region is ABCDFA
and Z = 30 x + 20 Y

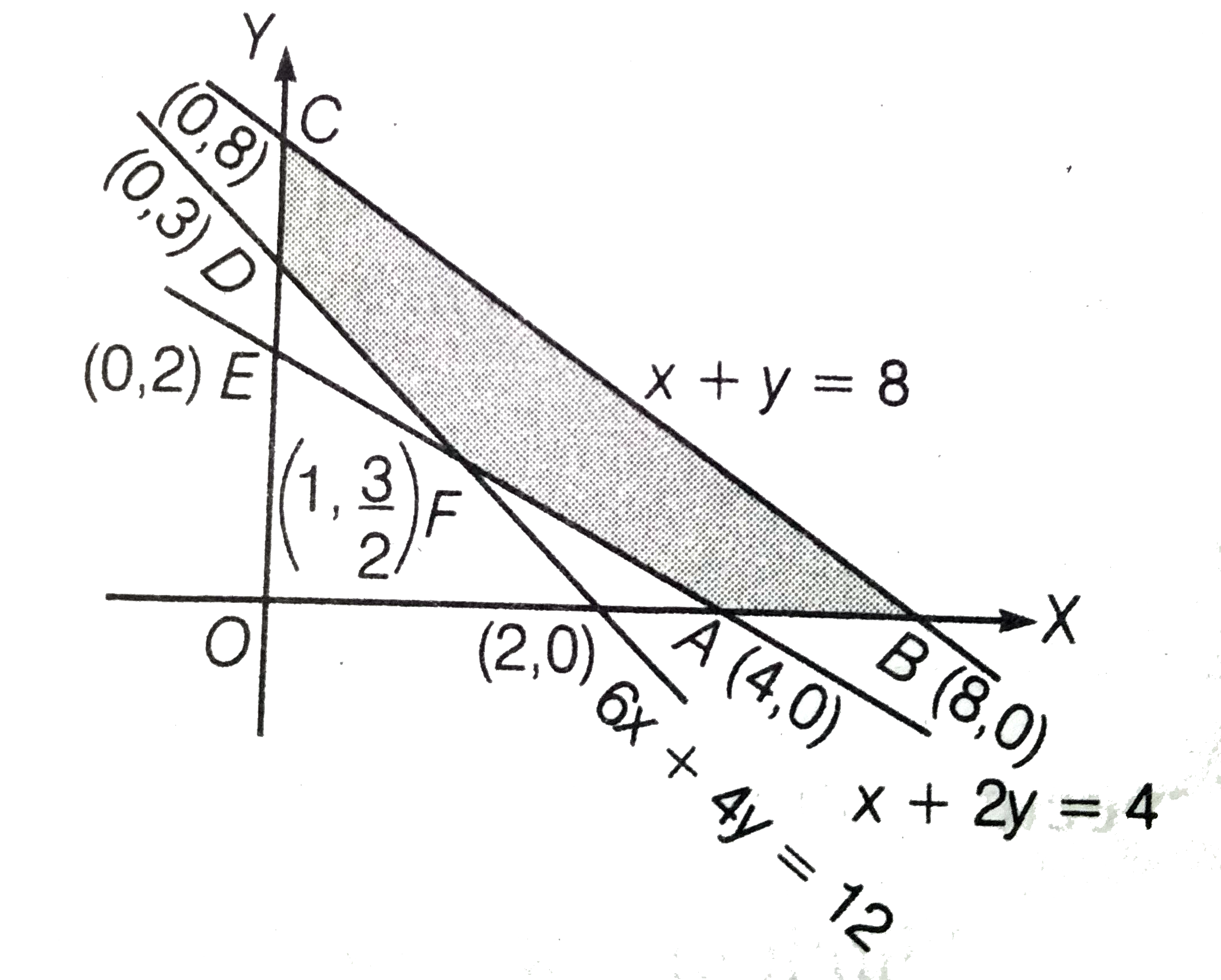
Now, at `A(4,0) , Z= 30 xx 4 +0 = 120`
`B(8,0)Z = 30 xx 8+0 = 240 `
`C(0,8) , Z=0 +20 xx 8 = 160 ` ,brgt ` D(0,3), Z =0 +20 xx 3 = 60 ` (Minimum) and ` F(1,3/2)`
`Z = 30 xx 1+20 xx 3/2 = 60 ` (Maximum)
it is clear that minimum vlaue of Z is 60 at pionts D(0,3) and ` F(1,3/2)` . So, minimum vlaue of Z is obtained at every point of the line segment DF. Hence infinitely many solutions exist .