Two identical rings, 1 and 2 are rotated about a transverse tangent and a diameter as shown by applying torques τ1andτ2, respectively. If they have the same (in magnitude) angular accelerations.
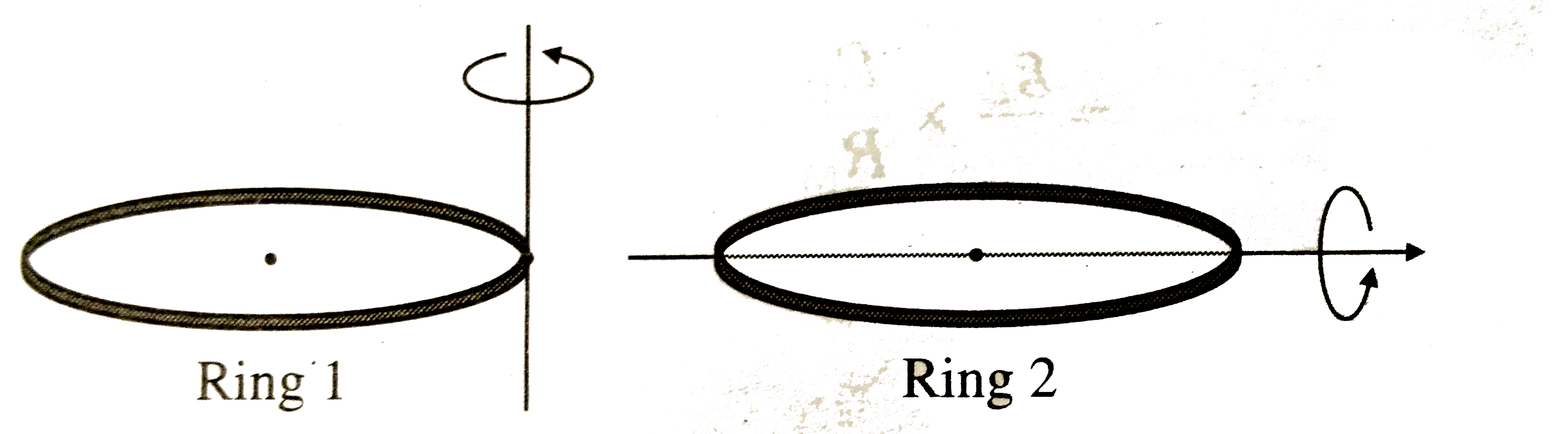
τ1=4τ2
τ1=2τ2
τ1=τ2
4τ1=τ2
The correct Answer is:A
I1=2MR2andI2=12MR2
∝=τ1I1=τ2I2 ∴τ1τ2=I1I2=4
Topper's Solved these Questions
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MULTIPLE CHOICE QUESTIONSEXERCISE - Oscillations5videos
MULTIPLE CHOICE QUESTIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MULTIPLE CHOICE QUESTIONSEXERCISE - Elasticity5videos
MULTIPLE CHOICE QUESTIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MULTIPLE CHOICE QUESTIONSEXERCISE - Gravitation8videos
MULTIPLE CHOICE QUESTIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MODEL QUESTION PAPER FOR PRACTICEEXERCISE - SECTION-D5videos
MODEL QUESTION PAPER FOR PRACTICE
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Semiconductors Devices (Long Answer ( LA) ( 4 marks Each) )3videos
QUESTION BANK 2021
Similar Questions
Does a body rotating about a fixed axis have to be perfectly rigid for all points on the body to have the same angular velocity and the same angular acceleration ?Explain.
View SolutionTwo discs A and B are in contact and rotating with angular velocity with angular velocities ω1andω2 respectively as shown. If there is no slipping between the discs, then
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Two identical rings A and B are acted upon by torques τA and τB respectively. A is rotating about an axis passing through the centre of mass and perpendicular ot the plane of the ring. B is rotating about a chord at a distance 1√2 times the radius of the ring. If the angular acceleration of the ring is the same, then
AτA=τBBτA>τBCτA<τBDNothing can be said about τA and τB as data are insufficient - Question 2 - Select One
A hollow cylinder of mass M and radius R is rotating about its axis of symmetry and a solid sphere of same mass and radius is rotating about an axis passing through its centre. If torques of equal magnitude are applied to them, then the ratio of angular accelerations produced is
A25B52C54D45 - Question 3 - Select One
A hollow cylinder of mass M and radius R is rotating about its axis of symmetry and a solid sphere of same mass and radius is rotating about an axis passing through its centre. It torques of equal magnitude are applied to them, then the ratio of angular accelerations produced is
A2:5B3:5C5:2D2:3
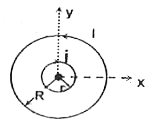
Two circular loops of radii R and r (R > > r) have same cenre and carry currents I and i respectively. Find the maximum torque and maximum angular acceleration of smallar loop, if it is free to rotate about y axis where as the bigger loop is fixed. (Neglect the inductance of the system). The mas of the smaller loop is m.
 View Solution
View SolutionA toruque τ produces an angular acceleration in a body rotating about an axis of rotation. The moment of inertia of the body is increased by 50% by redistributing the masses, about the axis of rotation. To maintain the same angular acceleration, the torque is changed to τ'. What is the relation between τandτ'?
View SolutionTwo identical masses are connected to a horizontal thin massless rod as shown in the figure. When their distance from the pivot is x, a torque produces an angular acceleration α1. If the masses are now repositioned so that they are at distance 2x each from the pivot, the same torque will produce an angular acceleration α2 such that ,
 View Solution
View SolutionIf two disc of moment of inertia l1 and l2 rotating about collinear axis passing through their centres of mass and perpendicular to their plane with angular speeds ω1 and ω2 respectively in opposite directions are made to rotate combinedly along same axis, then the magnitude of angular velocity of the system is
View SolutionTwo uniform spheres of mass M have radii R and 2R. Each sphere is rotating about a fixed axis through a diameter, the rotational kinetic energies of the sphere are identical. What is the ratio of the magnitude of the angular momentum of these spheres gt That is, L2RLR=
View Solution

