A battery of emf 2V and internal resistance 0.5(Ω)is connected across a resistance in 1 second?
To solve the problem step by step, we will determine how many electrons cross through a cross-section of the resistance in one second when a battery of emf 2V and internal resistance 0.5Ω is connected across a resistance of 9.5Ω.
Step 1: Calculate the Total Resistance in the Circuit
The total resistance (R_total) in the circuit is the sum of the internal resistance of the battery (r) and the external resistance (R).
Rtotal=r+R=0.5Ω+9.5Ω=10Ω
Step 2: Calculate the Current Flowing Through the Circuit
Using Ohm's law, the current (I) flowing through the circuit can be calculated using the formula:
I=VRtotal
Where:
- V=2V
- Rtotal=10Ω
Substituting the values:
I=2V10Ω=0.2A
Step 3: Relate Current to Charge
Current is defined as the rate of flow of charge. Therefore, the charge (Q) flowing through the circuit in one second can be calculated as:
Q=I×t
Where:
- I=0.2A
- t=1s
Substituting the values:
Q=0.2A×1s=0.2C
Step 4: Calculate the Number of Electrons
The charge of a single electron (e) is approximately 1.6×10−19C. The number of electrons (n) that flow through the circuit in one second can be calculated using the formula:
n=Qe
Substituting the values:
n=0.2C1.6×10−19C/electron≈1.25×1018electrons
Final Answer
Approximately 1.25×1018 electrons cross through a cross-section of the resistance in one second.
---
The total resistance (R_total) in the circuit is the sum of the internal resistance of the battery (r) and the external resistance (R).
Using Ohm's law, the current (I) flowing through the circuit can be calculated using the formula:
- V=2V
- Rtotal=10Ω
Current is defined as the rate of flow of charge. Therefore, the charge (Q) flowing through the circuit in one second can be calculated as:
- I=0.2A
- t=1s
The charge of a single electron (e) is approximately 1.6×10−19C. The number of electrons (n) that flow through the circuit in one second can be calculated using the formula:
Approximately 1.25×1018 electrons cross through a cross-section of the resistance in one second.
Topper's Solved these Questions
- ELECTRIC CURRENT IN CONDUCTORSBOOK - HC VERMACHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - worked out Example
1 Videos
- ELECTRIC CURRENT IN CONDUCTORSBOOK - HC VERMACHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - Short Answer
17 Videos
- ELECTRIC CURRENT IN CONDUCTORSBOOK - HC VERMACHAPTER - ELECTRIC CURRENT IN CONDUCTORSEXERCISE - Exercises
84 Videos
- DISPERSION AND SPECTRABOOK - HC VERMACHAPTER - DISPERSION AND SPECTRAEXERCISE - Exercises
11 Videos
- ELECTRIC CURRENT THROUGH GASESBOOK - HC VERMACHAPTER - ELECTRIC CURRENT THROUGH GASESEXERCISE - Exercises
23 Videos
Similar Questions
A battery of emf 2 V and internal resistance 0.5Ω is connected across a resistance of 9.5Ω. How many electrons pass through a cross-section of the resistance in 1 second ?
View SolutionA wire of uniform cross section of resistance 16Ω is divided into four pieces in the ratio of 1 : 2 : 3 : 4 and ll the four resistros are connected in the form of a square . Calculate the current drawn from the battery, if a battery of emf 10 V and internal resistance 0.5Ω is connected across the resistor with least resistance.
View Solution
Knowledge Check
Three equal resistances, each of RΩ are connected as shown in figure. A battery of emf 2 V and internal resistance 0.1Ω is connected across the circuit. Calculate the value of R for which the heat generated in the circuit is maximum?
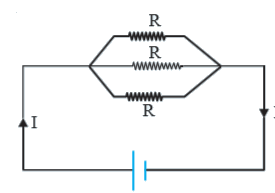 A0.2ΩB0.3ΩC0.4ΩDnone of these
A0.2ΩB0.3ΩC0.4ΩDnone of theseA cell of e.m.f. 2V and internal resistance 0.5Ω is connected across a resistor R. The current that flows is same as that, when a cell of e.m.f. 1.5V and internal resistance 0.3Ω is connected across the same resistor. Then
AR=0.3ΩBR=0.6ΩCR=0.5ΩDR=0.75ΩA battery of emf 10 V and internal resistance 3Ωis connected to a resistor. The current in the circuit is 0.5 A . The terminal voltage of the battery when the circuit is closed is
A10 VB0VC1.5 VD8.5 V
A battery of emf 12 V and internal resistance 2 Ω is connected to a 4 Ω resistor as shown in the Fig. Show that a voltmeter when placed across the cell and across the resistor, in turn, gives the same reading.
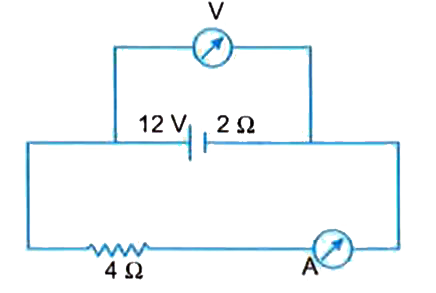
View SolutionA battery of emf ε and internal resistance r, when connected across an external resistance of 12 Ω , produces a current of 0.5 A. When connected across a resistance of 25Ω, it produces a current of 0.25 A. Determine the emf and internal resistance of the cell.
View SolutionA battery of e.m.f 10V and internal resistance 3Omega is connected to a resistor.If the current in the circuit is 0.5A,then the resistance of the resistor is
View SolutionA battery having e.m.f. 5 V and internal resistance 0.5Ω is connected with a resistance of 4.5Ω then the voltage at the terminals of battery is
View SolutionA battery having e.m.f. 5 V and internal resistance 0.5Ω is connected with a resistance of 4.5Ω then the voltage at the terminals of battery is
View Solution
