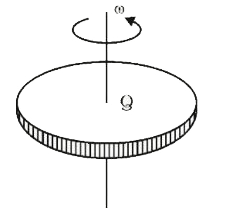
consider a nonconducting ring of radius r and mass m which has a totoal chargej q distributed uniformly on it the ring is rotated about its axis with an angular speed ω. (a) Find the equivalent electric current in the ring (b) find the magnetic moment μ of the . (c) show thatμ=q2m where l is the angular momentum of the ring about its axis of rotation.
(a) I=qT=qw2π[∴W=2πT⇒T=2πw]
(b)m=nia=ia
=qw2ππr2=qwr22
Magnetic moment
mu = ia = (qL)/(2m).
Topper's Solved these Questions
Similar Questions
Consider a nonconducting disc of radius r and mass m which has a total charge q distributed unifromly on it.The ring is rotated about its axis with an angular speed ω.(a) The equivalent electric current in the ring is qωNπ.Then find value of N (b) The magnetic moment of the ring is 4qωr2N.Then find value of N.
View SolutionConsider a non conducting plate of radius a and mass m which has a charge q distributed uniformly over it, The plate is rotated about its own axis with a angular speed ω. Show that the magnetic moment M and the angular momentum L of the plate are related as ML=q2m.
View Solution
Knowledge Check
Consider a solid sphere of radius r and mass m which has a charge q distributed uniformly over its volume. The sphere is rotated about a diameter with an angular speed ω. The magnetic moment of the sphere is
A13qωr2B15qωr2C17qωr2D19qωr2A thin disc of radius R has charge Q distributed uniformly on its surface. The disc is rotated about one of its diametric axis with angular velocity ω. The magnetic moment of the arrangement is
AQR2ω8BQR2ω4CQR2ω2DZeroA conducting ring of radius R has charge Q distributed unevenly over it. If it rotates with an angular velocity ω, the equivalent current will be
AzeroBQωCQω2πDQω2πR
A non-conducting ring of radius r has charge q distributed unevenly over it. What will be the equivalent current if it rotates with an angular velocity Ω?
View SolutionA non-conducting thin disc of radius R and mass m having charge uniformly over one side with surface density σ rotates about its axis with an angular velocity ω. Find :
(a) the magnetic induction at the centre of the disc,
(b) the magnetic moment of the disc.
( c) the ratio of magnetic moment and angular momentum of disc.
View SolutionA ring of dielectric material, with a total charge +q distributed uniformly over its surface, rotates with angular speed ω. Find the magnetic moment of the ring .Compare its magnetic moment with its angular momentum , assuming that its mass is m.
View SolutionA ring of mass m and radius R is being rotated about its axis with constant angular velocity ω in the gravity free space. Find tension in the ring.
View SolutionA non-conducting ring of radius R has charge Q distributed unevenly over it. If it rotates with an angular velocity ω the equivalent current will be:
View Solution
