A ball of mass when suspended by a spring stretches the latter by . Due to external vertical lforce varying according to a harmonic law with amplitude the ball performs forced oscillations. The logarighmic damping decrement if equal to . Neglecting the mass the spring, find the angular frequency of the external force at which the displacemetn amplitude of the ball is maximum. What is the magnitude of that amplitude ?
For the spring
where is its stiffness coefficient. Thus
The equation of motion of the ball is
Here, or
To find the solution of the above equation we look for the solution of the auxiliary equation
Clearyl we can tek Re Now we look for a particular integral for of the form
Thus, substitution gives and we get
so taking the realpart
The amplitude of this oscillation is maximum when the denominator is minimum.
This happens when
is minimum for
Thus
and
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electric Oscillations
56 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Elastic Waves
39 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise
2 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
A deadweight suspended from a weightless spring extends it by Δx=9.8cm. What will be the oscillation period of the dead weight when it is pushed slightly in the vertical direction ? The logarithmic damped decrement is equal to λ=3.1
View SolutionA certain resonance curve describes a mechanical oscillating system with logarithmic damping decrement λ=1.60. For this curve find the ration of the maximum displacemetn amplitude to the displacement amplitude at a very low frequency.
View Solution
Knowledge Check
A ball hung from a vertical spring oscillates in simple harmonic motion with an angular frequency of 2.6 rad/s and an amplitude of 0.075m. What is the maximum acceleration of the ball?
A0.13m/s2B0.20m/s2C0.51m/s2D2.6m/s2
A block of mass m is suspended from a spring and executes vertical SHM of time period T as shown in Fig. The amplitude of the SHM is A and spring is never in compressed state during the oscillation. The magnitude of minimum force exerted by spring on the block isAmg−4π2T2mABmg+4π2T2mACmg−π2T2mADmg+π2T2mA
A ball of mass m=50g is suspended by a weightless spring with stiffness x=20.0N/m. Due to external vertical harmonic force with frequency ω=25.0s−1 the ball performs steady − state oscillations with amplitude a=1.3cm. In this case the displacement of the ball lags in phase behind the external force by φ=34π. Find :
(a) the quality factor of the given oscillator ,
(b) the work performed by the external force during one oscillation period.
View SolutionA body A mass m1=1kg and body B of mass m2=4.1kg. The body A perform free vertical harmonic oscillations with the amplitude 1.6cm and frequency 25Hz. Neglecting the mass of the spring , find the maximum and m inimum value of force that the system exerts on the hearing surface.
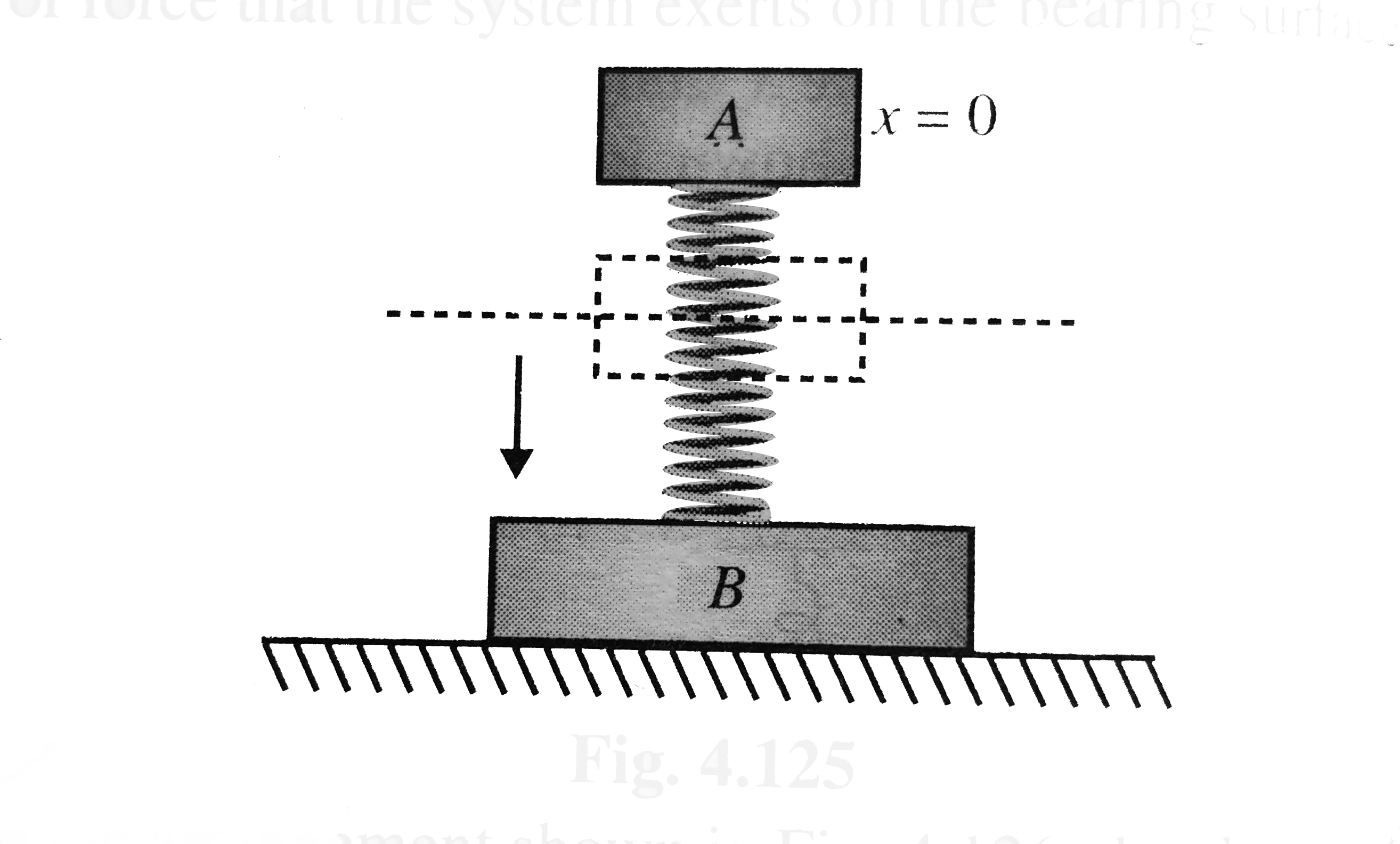
View SolutionA body A mass m1=1kg and body B of mass m2=4.1kg. The body A perform free vertical harmonic oscillations with the amplitude 1.6cm and frequency 25Hz. Neglecting the mass of the spring , find the maximum and m inimum value of force that the system exerts on the hearing surface.

View SolutionA ball of mass m suspended by a weightless spring can perform vertical oscillations with damping coefficient β. The natural oscillation frequency is equal to ω0. Due to the external vertical force varying as F=F0cosωt the ball performs steady − state harmonic oscillations. Find :
(a) the mean power (:P:) , develocped by the force F. averaged over one oscillations perod,
(b) the frequency ω of the force F at which (:P:) is maximum, what is (:P:)max equal to ?
View Solution
A block of mass m is suspended from a spring and executes vertical SHM of time period T as shown in Fig. The amplitude of the SHM is A and spring is never in compressed state during the oscillation. The magnitude of minimum force exerted by spring on the block is
View SolutionA ball of mass, m is attractive to two springwhich are already stretched so that each pulls on to ball with a force F. length of each spring . In this Determine the period of small oscillations of the ball in a difference perpendicular of length of psirng. Difference of the mass of spring and gravity .
View Solution
