A block of mass is placed on a rough horizontal plane A time dependent force acts on the block where Coefficient of friction force of friction between the block and the plane at is
The correct Answer is:d
To solve the problem step by step, we will calculate the force of friction acting on the block at t=2 seconds.
Step 1: Calculate the Normal Force
The normal force N acting on the block can be calculated using the formula:
N=mg
where m is the mass of the block and g is the acceleration due to gravity (approximately 10m/s2).
Given:
- Mass m=4kg
- Acceleration due to gravity g=10m/s2
Calculating the normal force:
N=4kg×10m/s2=40N
Step 2: Calculate the Maximum Force of Friction
The maximum force of friction fmax can be calculated using the formula:
fmax=μN
where μ is the coefficient of friction.
Given:
- Coefficient of friction μ=0.8
Calculating the maximum force of friction:
fmax=0.8×40N=32N
Step 3: Calculate the Applied Force at t=2 seconds
The applied force F is given by the equation:
F=kt2
where k is a constant.
Given:
- k=2N/s2
- t=2s
Calculating the applied force:
F=2N/s2×(2s)2=2×4=8N
Step 4: Determine the Force of Friction
Since the applied force F (8 N) is less than the maximum force of friction fmax (32 N), the force of friction will equal the applied force to prevent motion.
Thus, the force of friction f at t=2 seconds is:
f=F=8N
Final Answer
The force of friction between the block and the plane at t=2 seconds is 8N.
---
The normal force N acting on the block can be calculated using the formula:
N=mg
where m is the mass of the block and g is the acceleration due to gravity (approximately 10m/s2).
- Mass m=4kg
- Acceleration due to gravity g=10m/s2
N=4kg×10m/s2=40N
The maximum force of friction fmax can be calculated using the formula:
fmax=μN
where μ is the coefficient of friction.
- Coefficient of friction μ=0.8
fmax=0.8×40N=32N
The applied force F is given by the equation:
F=kt2
where k is a constant.
- k=2N/s2
- t=2s
F=2N/s2×(2s)2=2×4=8N
Since the applied force F (8 N) is less than the maximum force of friction fmax (32 N), the force of friction will equal the applied force to prevent motion.
f=F=8N
The force of friction between the block and the plane at t=2 seconds is 8N.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Integer Type Questions
7 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Assertion- Reason Type Questions
17 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Problems for practice
112 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 NCERT Comprehension
4 Videos
- MATHEMATICAL TOOLSBOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks
5 Videos
Similar Questions
A block of mass 4kg is placed on a rough horizontal plane A time dependent horizontal force F=kt acts on the block (k=2N/s). Find the frictional force between the block and the plane at t=2 seconds and t=5 seconds (μ=0.2) .
View SolutionA block of mass 5kg is placed on a rough horizontal plane.A time dependent horizontal force F=(t2+2t) N acts on the block. The friction force between the block and the plane at t=3s is (μ=0.4)
View Solution
Knowledge Check
A block of mass 8 kg is placed on a rough horizontal plane. A time dependent force F=kt2acts on the block, where k=3N/s2. Coefficient of friction is0.6. Force of friction between the block and the plane at t=1s is
A48NB4NC2ND3NA block of mass 4kg is placed on a rough horizontal force plane. A time dependent horizontal force F=kt acts on the block. Here k=2Ns−1. The frictional force between the block and plane at time t=2s is (μ=0.2)
A4NB8NC12ND10NA block is placed on a rough horizontal plane. A time-dependent horizontal force F=kt2 acts on the block. Here k is a positive constant. Acceleration -time graph of the block is
A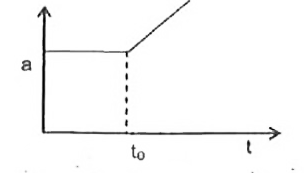 B
B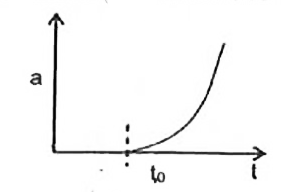 C
C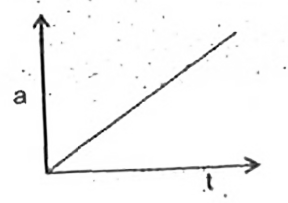 D
D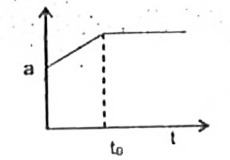
A block is placed on a rough horizontal plane. A time dependent horizontal force F=K t acts on the block K is a postive constant. Accelration-time graph of the block is
View SolutionA block of mass m is placed on a rough horizontal surface. If minimum force to pull the block is mg2 then coefficient of friction is :
View SolutionA block of mass 10 kg is placed on the rough horizontal surface. A pulling force F is acting on the block which makes an angle θ above the horizontal. If coefficient of friction between block and surface is 43 then minimum value of force required to just move the block is (g=10ms2)
View SolutionA block of mass 4 kg is kept on a rough horizontal surface. The coefficient of static friction is 0.8. If a force of 19 N is applied on the block parallel to the floor, then the force of friction between the block and floor is
View SolutionA block is sliding on a rough horizontal surface. If the contact force on the block is √2 times the frictional force, the coefficient of friction is
View Solution
