A block of mass M is kept on as rough horizontal surface. The coefficient of static friction between the block and the surface is μ. The block is to be pulled by applying a force to it. What minimum force is needed to slide the block? In which direction should this force act?
To solve the problem of determining the minimum force needed to slide a block of mass M on a rough horizontal surface with a coefficient of static friction μ, we can follow these steps:
Step 1: Understand the Forces Acting on the Block
The forces acting on the block include:
- The gravitational force mg acting downward.
- The normal force N acting upward.
- The applied force F which can be applied at an angle θ with the horizontal.
- The frictional force f acting opposite to the direction of the applied force.
Step 2: Break Down the Applied Force into Components
When the force F is applied at an angle θ:
- The horizontal component of the force is Fcosθ.
- The vertical component of the force is Fsinθ.
Step 3: Write the Equation for Normal Force
The normal force N can be expressed as:
N=mg−Fsinθ
This equation accounts for the weight of the block and the vertical component of the applied force.
Step 4: Write the Equation for Limiting Friction
The maximum static friction force fmax is given by:
fmax=μN=μ(mg−Fsinθ)
Step 5: Set Up the Condition for Sliding
For the block to start sliding, the horizontal component of the applied force must be equal to or greater than the maximum static friction:
Fcosθ≥μ(mg−Fsinθ)
Step 6: Rearrange the Inequality
Rearranging gives:
Fcosθ+μFsinθ≥μmg
Factoring out F from the left side:
F(cosθ+μsinθ)≥μmg
Step 7: Solve for the Minimum Force F
To find the minimum force F:
F≥μmgcosθ+μsinθ
Step 8: Determine the Angle for Minimum Force
To minimize F, we need to maximize the denominator cosθ+μsinθ. This can be achieved by using trigonometric identities or calculus. The angle θ that maximizes this expression is given by:
tanθ=μ
Thus, θ=tan−1(μ).
Final Result
The minimum force required to slide the block is:
Fmin=μmgcos(tan−1(μ))+μsin(tan−1(μ))
This simplifies to:
Fmin=μmg√1+μ2
And the direction of the force should be at an angle θ=tan−1(μ) with the horizontal.
The forces acting on the block include:
- The gravitational force mg acting downward.
- The normal force N acting upward.
- The applied force F which can be applied at an angle θ with the horizontal.
- The frictional force f acting opposite to the direction of the applied force.
When the force F is applied at an angle θ:
- The horizontal component of the force is Fcosθ.
- The vertical component of the force is Fsinθ.
The normal force N can be expressed as:
N=mg−Fsinθ
This equation accounts for the weight of the block and the vertical component of the applied force.
The maximum static friction force fmax is given by:
fmax=μN=μ(mg−Fsinθ)
For the block to start sliding, the horizontal component of the applied force must be equal to or greater than the maximum static friction:
Fcosθ≥μ(mg−Fsinθ)
Rearranging gives:
Fcosθ+μFsinθ≥μmg
Factoring out F from the left side:
F(cosθ+μsinθ)≥μmg
To find the minimum force F:
F≥μmgcosθ+μsinθ
To minimize F, we need to maximize the denominator cosθ+μsinθ. This can be achieved by using trigonometric identities or calculus. The angle θ that maximizes this expression is given by:
tanθ=μ
Thus, θ=tan−1(μ).
The minimum force required to slide the block is:
Fmin=μmgcos(tan−1(μ))+μsin(tan−1(μ))
This simplifies to:
Fmin=μmg√1+μ2
And the direction of the force should be at an angle θ=tan−1(μ) with the horizontal.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Solved Examples12videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.125videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer5videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE2videos
PROPERTIES OF SOLIDS AND FLUIDS
Similar Questions
A block of mass m is moving on a rough horizontal surface. μ is the coefficient of kinetic friction between the block and the surface. What is the net force exerted by the surface on the block?
View SolutionA block of mass 70 kg is kept on a rough horizontal surface and coefficient of static friction between block and surface is 0.4 . A man is trying to pull the block by applying a horizontal force .The net contact force exerted by the surface on the block is F , then:
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
A10NB5NC2.5ND20N
A block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
View SolutionA block of mass 50 kg can slide on a rough horizontal surface. The coefficient of friction between the block and the surface is 0.6. The least force of pull acting at an
angle of 30∘ to the upwawrd drawn ve3rtical which causes the block to just slide isView SolutionA block of mass m is pulled by a force of constant power P placed on a rough horizontal plane. The friction coefficient between the block and the surface is μ. Then
View SolutionA block of mass m is slowly pulled along a curved surface from position 1 and 2. If the coefficient of kinetic friction between the block and surface is μ, find the work done by the applied force.
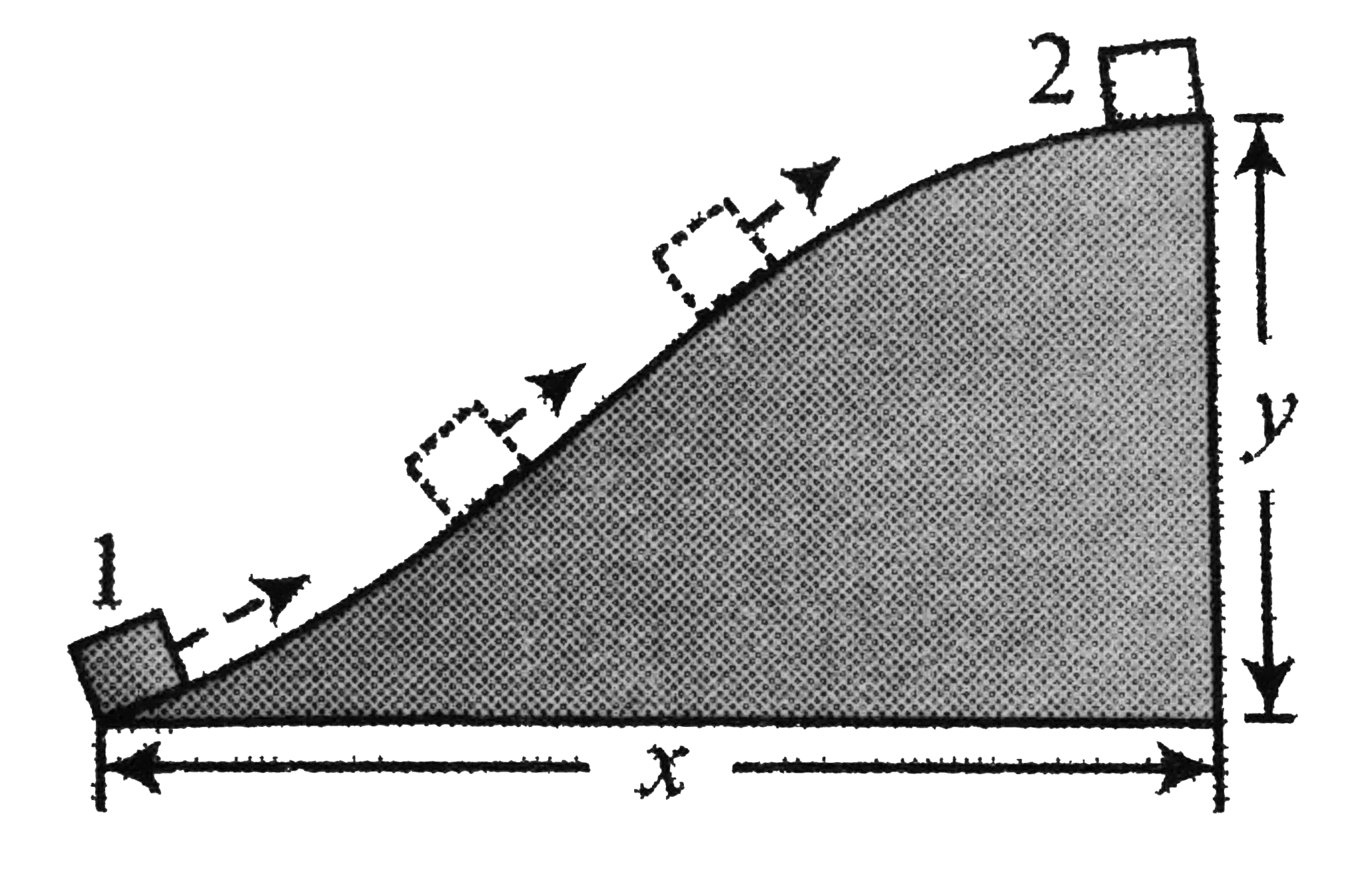 View Solution
View SolutionA block is kept on a rough horizontal surface as shown. Its mass is 2 kg and coefficient of friction between block and surface (μ)=0.5. A horizontal force F is acting on the block. When
 View Solution
View SolutionA block of mass 4 kg is kept on a rough horizontal surface. The coefficient of static friction is 0.8. If a forace of 19 N is applied on the block parallel to the floor, then the force of friction between the block and floor is
View SolutionA block of mass M rests on a rough horizontal surface as shown. Coefficient of friction between the block and the surface is μ. A force F=mg acting at angle θ with the vertical side of the block pulls it. In which of the following cases the block can be pulled along the surface?
 View Solution
View Solution
