A block of mass si suspended by two strings angles and with the horizontal. Find the tensions in the strings.
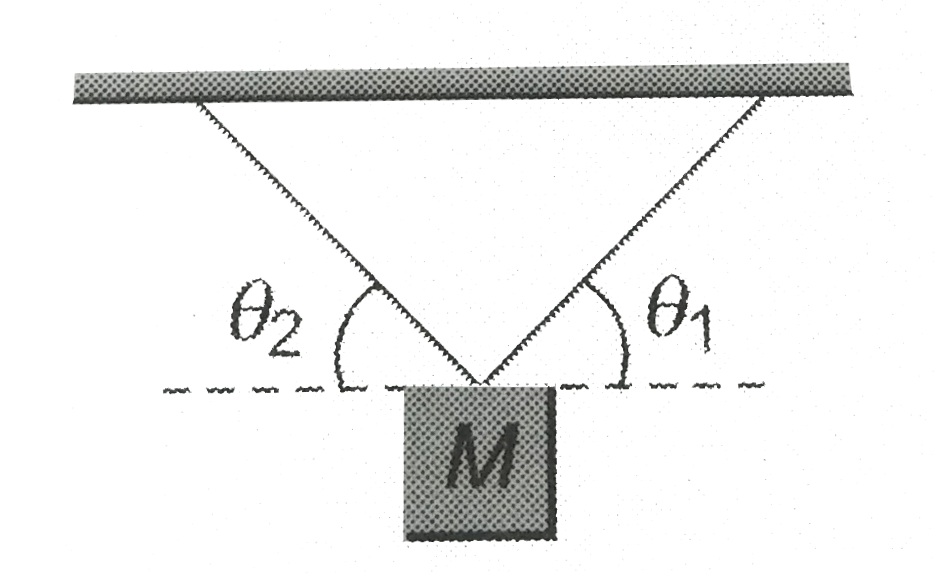
The block is in equilibrium, i.e. net force on it is zero.
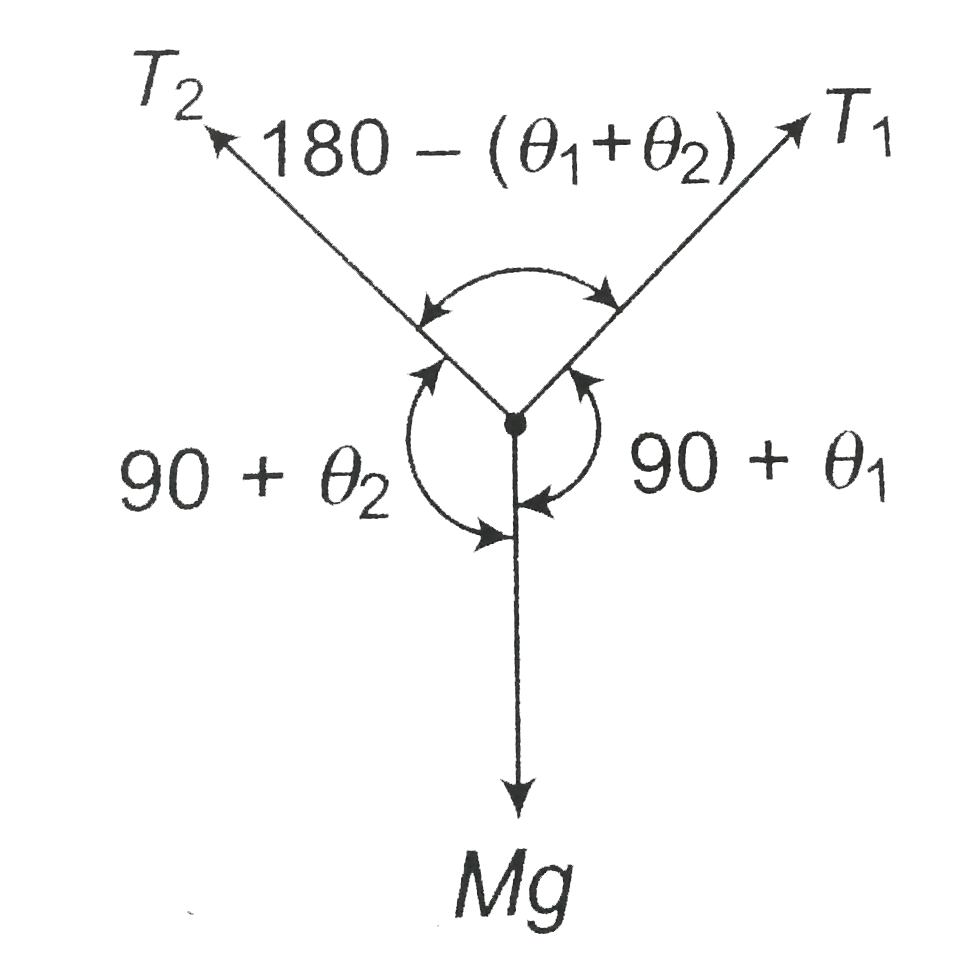
By Lami's theorem
OR
Resolving and in horizontal and vertical directions
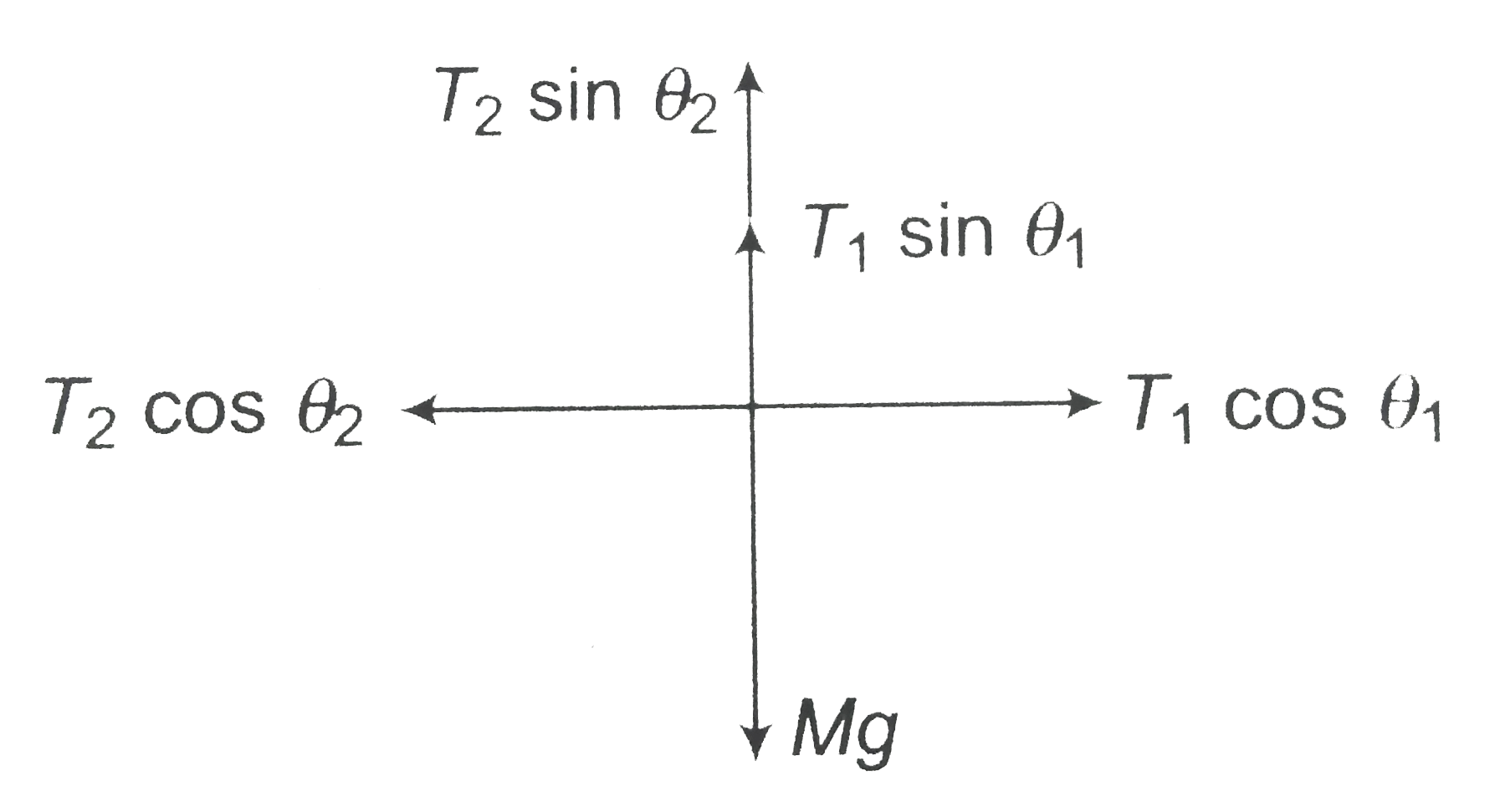
(i)
(ii)
From (i) in (ii)
Topper's Solved these Questions
Similar Questions
A body of mass mis suspended by two strings making angles αandβ with the horizontal. Find the tensions in the strings.
View SolutionA block of mass 0.2 kg is suspended from the ceiling by a light string. A second block of mass 0.3 kg is suspended from the first block through another string. Find the tensions in the two strings. Take g=10ms2.
View Solution
Knowledge Check
The block of mass m is at rest. Find the tension in the string A .
 AmgB2 mgC3 mgD4 mg
AmgB2 mgC3 mgD4 mgA block of mass m is supported by a string passing through a smooth peg as shown in the figure-2.130. Variation of tension in the string T as a function of θ best represented by (here the total length of the string is varied).
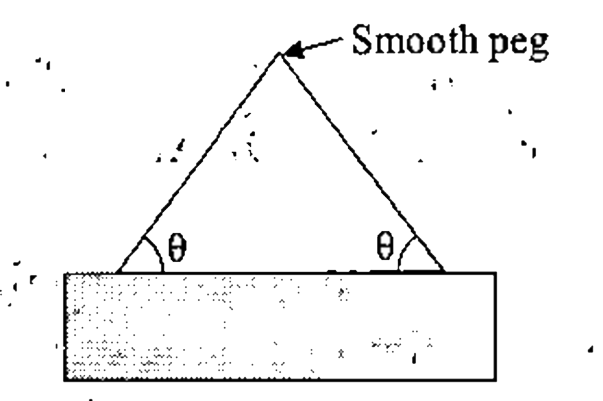 A
A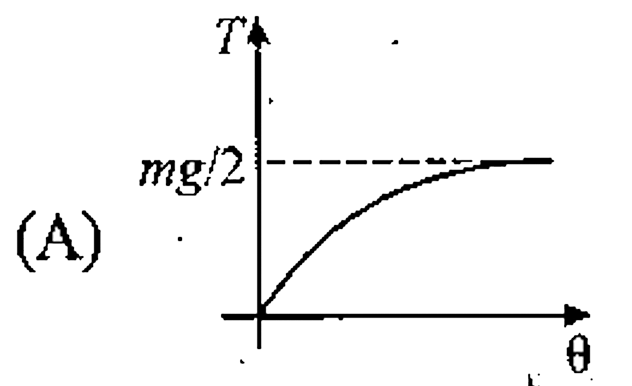 B
B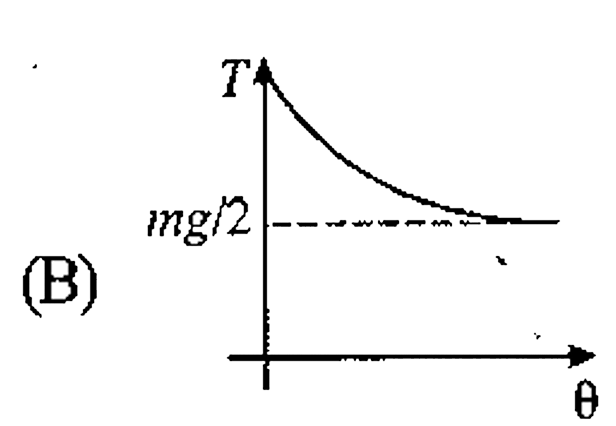 C
C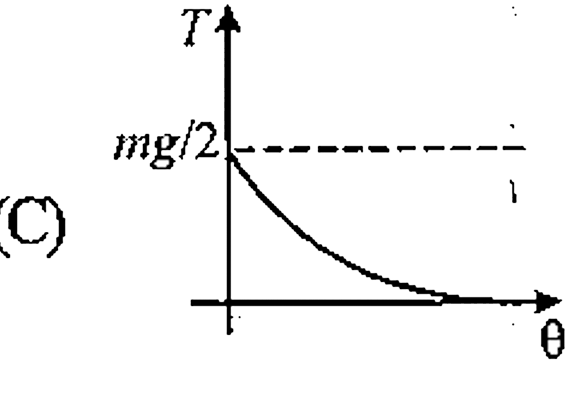 D
D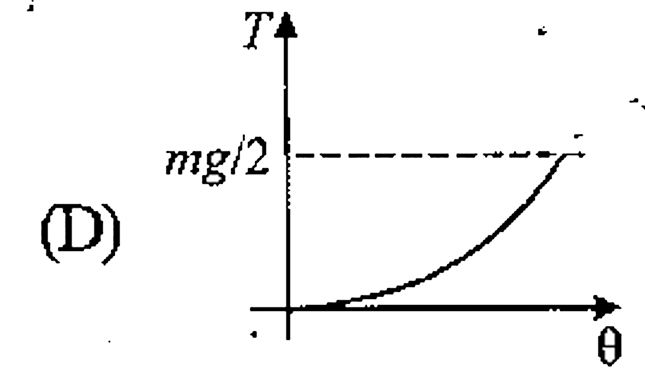
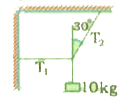
A block of mass 10 kg is suspended by three strings as shown in figure . Tension T2 is
 A100NB100√3NC√3×100ND50√3N
A100NB100√3NC√3×100ND50√3N
A block of mass 2 kg is kept at rest on a smooth inclied plane as shown in (a) with the help of a string Find the tension in the string and reaction on the block if the string is cut, find the acceleration of the block neglecting friction.
View SolutionA block of mass 10 kg is suspended with two strings as shown in the fig. Find the tensions T1andT2 in the two string.
View SolutionA block of mass 30 kg is suspended by three string as shown in fig, Find the tension in each string.

View SolutionA block of mass M is being pulled with the help of a string of mass m and length L. the horizontal force applied by the man on the string is F.

Determine
(a) Find the force exerted by the string on the block and acceleration of system.
(b) Find the tension at the mid point of the string.
(c ) Find the tension at a distance x from the end at which force is applied.
Assume that the block is kept on a frictionless horizontal surface and the mass is uniformly distributed in the string.
View SolutionA block of mass M is pulled on a smooth horizontal table by a string making an angle θ with the horizontal as shown in figure. If the acceleration of the block is a find the force applied by the string and by the table N on the block.

View Solution
