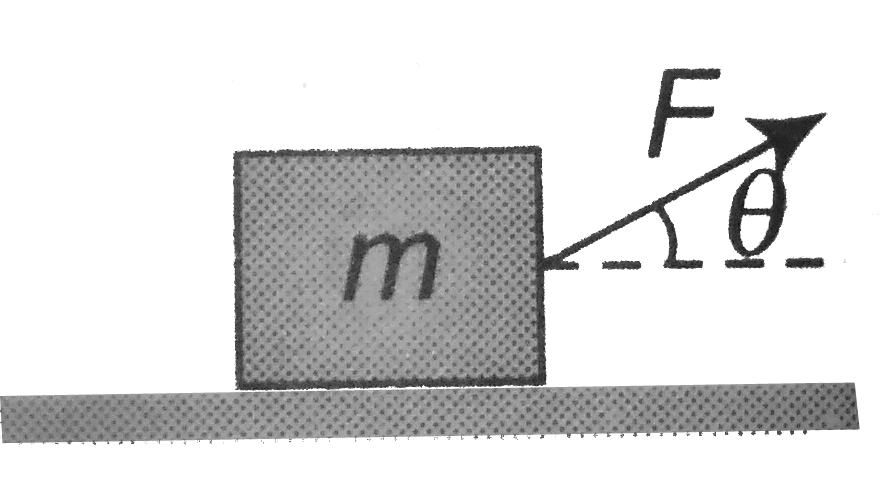
A block placed on a horizotnal surface is being pushed by a force F making an angle θ with the vertical. If the friction coefficient is μ. How much force is needed to get the block just started. Discuss the situation when tanθ<μ.
The situation is shown in ure. In the limiting equilibrium the frictioN/Al force f will be equal to μN. For horizontal equilibrium
Fsinθ=μN
 <br> For verticla equilibrium <br> F cos theta+mgN<span class=) .<br>ElimiNAt∈gNomtheseequations<br>F sin theta=mu F costheta+mu mg<br>or F=(mu mg)/(sintheta-mucostheta)Iftanthetaltmuwehave(sintheta-mucostheta)lt0andthenFis¬ative.Sofor∠s≤ssthantan^-1mu. One cannot push the block ahead, however large the force may be.
.<br>ElimiNAt∈gNomtheseequations<br>F sin theta=mu F costheta+mu mg<br>or F=(mu mg)/(sintheta-mucostheta)Iftanthetaltmuwehave(sintheta-mucostheta)lt0andthenFis¬ative.Sofor∠s≤ssthantan^-1mu. One cannot push the block ahead, however large the force may be.
Topper's Solved these Questions
- FRICTIONBOOK - HC VERMACHAPTER - FRICTIONEXERCISE - Short Answer
11 Videos
- FRICTIONBOOK - HC VERMACHAPTER - FRICTIONEXERCISE - Objective 1
10 Videos
- FRICTIONBOOK - HC VERMACHAPTER - FRICTIONEXERCISE - Exercises
31 Videos
- FLUID MECHANICSBOOK - HC VERMACHAPTER - FLUID MECHANICSEXERCISE - Exercises
35 Videos
- GRAVITATIONBOOK - HC VERMACHAPTER - GRAVITATIONEXERCISE - Exercises
39 Videos
Similar Questions
A block placed on a horizotnal surface is being pushed by a force F making an angle θ with the vertical. If the friction coefficient is μ. How much force is needed to get the block just started. Discuss the situation when tanθ<μ.
View SolutionA block placed on a horizontal surface is being pushed by a force F making an angle θ with the vertical as shown in Fig. 7.39 . The coefficient of friction between block and surface is μ . (a) Find the force required to slide the block with uniform velocity on the floor . (b) Show that if θ is smaller than a certain

angle θ0 , the block cannot be made to slide across the floor , no matter how great the force be .
View Solution
Knowledge Check
A block of mass m is placed at rest on a horizontal rought surface with angle of friction ϕ. The block is pulley with a force F at an angle θ with the horizontal. The minimum value of F required to move the block is
Amgsinϕcos(θ−ϕ)Bmgcosϕcos(θ−ϕ)CmgtanϕDmgsinϕIn previous question, if we pull the block by the force F making an angle theta and the block remains stationary, the value of friction force is.
 AμmgBFcosθCμmgsinθ+μcosθDμmg√1+μ2
AμmgBFcosθCμmgsinθ+μcosθDμmg√1+μ2A block of mass m slides down an inclined plane which makes an angle θ with the horizontal. The coefficient of friction between the block and the plane is μ. The force exerted by the block on the plane is
AmgcosθB√μ2+1mgcosθCμmgcosθ√μ2+1Dμmgθ
A crate is pulled along a horizontal surface at constant velocity by an applied force F that makes an angle θ with the horizontal. The coefficient of kinetic friction between the crate and the surface is μ. Find the angle θ such that the applied force is minimum to slide the block. Also find the minimum value of this force.
View SolutionThe friction coefficient between the blocks is μ. If force F is applied on the lower block , analyse motion of two blocks.
View SolutionA block of mass m is placed on a rough horizontal surface. If minimum force to pull the block is mg2 then coefficient of friction is :
View SolutionA block of mass m lying on a rough horizontal plane is acted upon by a horizontal force P and another force Q inclined at an angle θ to the vertical. The minimum value of coefficient of friction of friction between the block and the surface for which the block will remain in equilibrium is

View SolutionA block of mass m is at rest on a rough inclined plane, whose friction coefficient is μ. Net force exerted by surface on block is :-
View Solution
