A bob of mass m attached with a string of length l tied to a point on ceiling is released from a position when its string is horizontal. At the bottom most point of its motion, an identical mass m gently stuck to it. Find the maximum angle from the vertical to which it rotates.
(a) cos−1(23)
(b) cos−1(34)
(c) cos−1(14)
(d) 60∘
The correct Answer is:B
To solve the problem step by step, we will follow the principles of energy conservation and momentum conservation.
Step 1: Determine the velocity of the bob just before the collision
When the bob is released from the horizontal position, it falls a vertical distance equal to the length of the string, l. The potential energy at the top is converted to kinetic energy at the bottom.
Using the formula for kinetic energy:
KE=PE
12mv2=mgh
where h=l (the height fallen). Thus,
12mv2=mgl
Solving for v:
v2=2gl⇒v=√2gl
Step 2: Apply conservation of momentum at the moment of collision
When the identical mass m strikes the bob, we can apply the conservation of linear momentum. The initial momentum before the collision is:
pinitial=mv+0=m√2gl
After the collision, both masses stick together and move with a common velocity v′:
pfinal=(m+m)v′=2mv′
Setting the initial momentum equal to the final momentum:
m√2gl=2mv′
Cancelling m (assuming m≠0):
v′=√2gl2=12√2gl
Step 3: Determine the height reached after the collision
After the collision, the combined mass rises to a height h due to the kinetic energy it has. Using the work-energy principle:
KEinitial=PEfinal
The initial kinetic energy after the collision is:
KE=12(2m)v′2=2m(12√2gl)2=2m⋅14⋅2gl=mgl
The potential energy at height h is:
PE=(2m)gh
Setting these equal gives:
mgl=2mgh
Cancelling m (assuming m≠0):
gl=2gh⇒h=l2
Step 4: Relate height to the angle θ
From the geometry of the pendulum, we know:
h=l(1−cosθ)
Substituting h=l2:
l2=l(1−cosθ)
Dividing both sides by l (assuming l≠0):
12=1−cosθ
Rearranging gives:
cosθ=12
Step 5: Solve for the angle θ
Taking the inverse cosine:
θ=cos−1(12)
This corresponds to:
θ=60∘
Final Answer
The maximum angle from the vertical to which the bob rotates is 60∘.
---
When the bob is released from the horizontal position, it falls a vertical distance equal to the length of the string, l. The potential energy at the top is converted to kinetic energy at the bottom.
KE=PE
12mv2=mgh
where h=l (the height fallen). Thus,
12mv2=mgl
Solving for v:
v2=2gl⇒v=√2gl
When the identical mass m strikes the bob, we can apply the conservation of linear momentum. The initial momentum before the collision is:
pinitial=mv+0=m√2gl
After the collision, both masses stick together and move with a common velocity v′:
pfinal=(m+m)v′=2mv′
Setting the initial momentum equal to the final momentum:
m√2gl=2mv′
Cancelling m (assuming m≠0):
v′=√2gl2=12√2gl
After the collision, the combined mass rises to a height h due to the kinetic energy it has. Using the work-energy principle:
KEinitial=PEfinal
The initial kinetic energy after the collision is:
KE=12(2m)v′2=2m(12√2gl)2=2m⋅14⋅2gl=mgl
The potential energy at height h is:
PE=(2m)gh
Setting these equal gives:
mgl=2mgh
Cancelling m (assuming m≠0):
gl=2gh⇒h=l2
From the geometry of the pendulum, we know:
h=l(1−cosθ)
Substituting h=l2:
l2=l(1−cosθ)
Dividing both sides by l (assuming l≠0):
12=1−cosθ
Rearranging gives:
cosθ=12
Taking the inverse cosine:
θ=cos−1(12)
This corresponds to:
θ=60∘
The maximum angle from the vertical to which the bob rotates is 60∘.
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONExercise:Level 2 Single Correct Option
Explore 1 Video
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONExercise:Level 2 More Than One Correct
Explore 8 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONExercise:Level 1 Subjective Questions
Explore 1 Video
- CENTRE OF MASS, IMPULSE AND MOMENTUMBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, IMPULSE AND MOMENTUMExercise:Comprehension type questions
Explore 15 Videos
- CIRCULAR MOTIONBook:DC PANDEY ENGLISHChapter:CIRCULAR MOTIONExercise:Medical entrances s gallery
Explore 19 Videos
Similar Questions
A bob of mass m is connected by string of length L to point P. The system is released from rest with the pendulum bob in a horizontal position. Which of the following graph is correctly showing the variation of T (tension) with Ɵ?
View SolutionA particle of mass m is tied to a string of length L and whirled into a horizontal plan. If tension in the string is T then the speed of the particle will be :
View SolutionA stone of mass m tied to a string of length l is rotated in a circle with the other end of the string as the centre. The speed of the stone is v. If the string breaks, the stone will move
View SolutionA simple pendulum consisting of a mass M attached to a string of length L is released from rest at an angle α. A pin is located at a distance l below the pivot point. When the pendulum swings down, the string hits the pin as shown in figure. The maximum angle θ which the string makes with the vertical after hitting the pin is
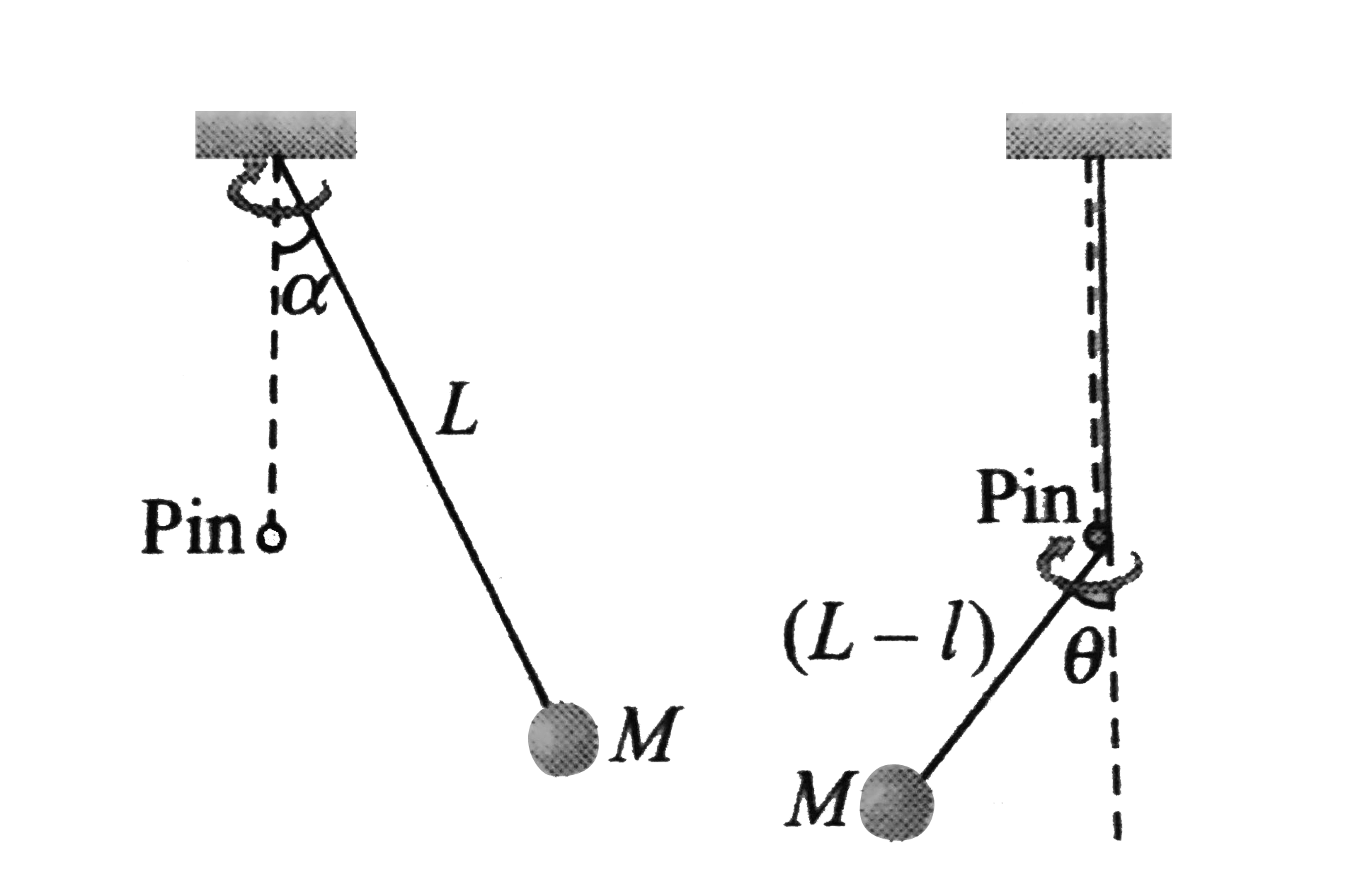
View SolutionA mass is attached to the end of a string of length l which is tied to a fixed point O. The mass is released from the initial horizontal position of the string. Below the point O at what minimum distance a peg P should be fixed so that the mass turns about P and can describe a complete circle in the vertical plane?
View SolutionThe string of a pendulum is horizontal. The mass of the bob is m. Now the string is released. The tension in the string in the lowest position is -
View SolutionIn a simple pendulum, the breaking strength of the string is double the weight of the bob. The bob is released from rest when the string is horizontal. The string breaks when it makes an angle θ with the vertical.
View SolutionA simple pendulum of mass m and length L is held in horizontal position. If it is released from this position, then find the angular momentum of the bob about the point of suspension when it is vertically below the point of suspension.
View SolutionA particle of mass m attached to a string of length l is descending circular motion on a smooth plane inclined at an angle α with the horizontal. For the particle to reach the highest point its velocity at the lowest point should exceed.
View SolutionA particle of mass m is attached to a string of length L and given velocity √10gL in the horizontal direction at the lowest point. Find tension in the string when the particle is at (i) lowest position (ii) highest position,
View Solution