A body floats in water with its one-third volume above the surface. The same body floats in a liquid with one-third volume immersed. The density of the liquid is
9 times more than that of water
2 times more than that of water
3 times more than that of water
1.5 times more than that of water
The correct Answer is:B
To solve the problem step by step, we will apply Archimedes' principle and analyze the conditions under which the body floats in both water and the liquid.
Step 1: Understand the problem
We have a body that floats in water with one-third of its volume above the surface. This means that two-thirds of the volume is submerged. In another liquid, the same body floats with one-third of its volume submerged, meaning two-thirds is above the surface.
Step 2: Define variables
Let:
- V = total volume of the body
- ρ = density of water
- ρL = density of the liquid
- g = acceleration due to gravity
- m = mass of the body
Step 3: Apply Archimedes' Principle for water
For the first situation (in water):
- Volume submerged = 2V3
- Weight of displaced water = ρ⋅g⋅2V3
- Weight of the body = m⋅g
According to Archimedes' principle:
ρ⋅g⋅2V3=m⋅g
Cancelling g from both sides:
ρ⋅2V3=m(Equation 1)
Step 4: Apply Archimedes' Principle for the liquid
For the second situation (in the liquid):
- Volume submerged = V3
- Weight of displaced liquid = ρL⋅g⋅V3
According to Archimedes' principle:
ρL⋅g⋅V3=m⋅g
Cancelling g from both sides:
ρL⋅V3=m(Equation 2)
Step 5: Equate the two equations
From Equation 1 and Equation 2, we can set them equal to each other since both equal m:
ρ⋅2V3=ρL⋅V3
Step 6: Simplify the equation
Cancelling V3 from both sides (assuming V≠0):
ρ⋅2=ρL
Thus, we find:
ρL=2ρ
Step 7: Conclusion
The density of the liquid is twice the density of water.
Final Answer
The density of the liquid is 2×ρ, where ρ is the density of water.
---
We have a body that floats in water with one-third of its volume above the surface. This means that two-thirds of the volume is submerged. In another liquid, the same body floats with one-third of its volume submerged, meaning two-thirds is above the surface.
Let:
- V = total volume of the body
- ρ = density of water
- ρL = density of the liquid
- g = acceleration due to gravity
- m = mass of the body
For the first situation (in water):
- Volume submerged = 2V3
- Weight of displaced water = ρ⋅g⋅2V3
- Weight of the body = m⋅g
ρ⋅g⋅2V3=m⋅g
Cancelling g from both sides:
ρ⋅2V3=m(Equation 1)
For the second situation (in the liquid):
- Volume submerged = V3
- Weight of displaced liquid = ρL⋅g⋅V3
ρL⋅g⋅V3=m⋅g
Cancelling g from both sides:
ρL⋅V3=m(Equation 2)
From Equation 1 and Equation 2, we can set them equal to each other since both equal m:
ρ⋅2V3=ρL⋅V3
Cancelling V3 from both sides (assuming V≠0):
ρ⋅2=ρL
Thus, we find:
ρL=2ρ
The density of the liquid is twice the density of water.
The density of the liquid is 2×ρ, where ρ is the density of water.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 1 Subjective
36 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Subjective Question
2 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Assertion and Reason
1 Videos
- EXPERIMENTSBOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
Knowledge Check
A block of wood floats in water with (4/5)th of its volume submerged. If the same block just floats in a liquid, the density of liquid in (kgm−3) is
A1250B600C400D800
A block of wood floats in freshwater with two - third of its volume submerged . In oil , the block floats with one - fourth of its volume submerged. The density of oil is
View SolutionA body floats in water with 40% of its volume outside water. When the same body floats in an oil. 60% of its volume remians outside oil. The relative density of oil is
View SolutionA body P floats in water with half its volume immersed. Another body Q floats in a liquid of density 34th of the density of water with two - third of the volume immersed. The ratio of density of P to that of Q is
View SolutionWhen a cube of wood floats in water,60% of its volume is submerged. When the same cube floats in an unknown fluid 85% of its volume is submerged. Find the densities of wood and the unknown fluid.
View SolutionA block of wood floats in water with (4/5)th of its volume submerged. If the same block just floats in a liquid, the density of liquid in (kgm−3) is
View Solution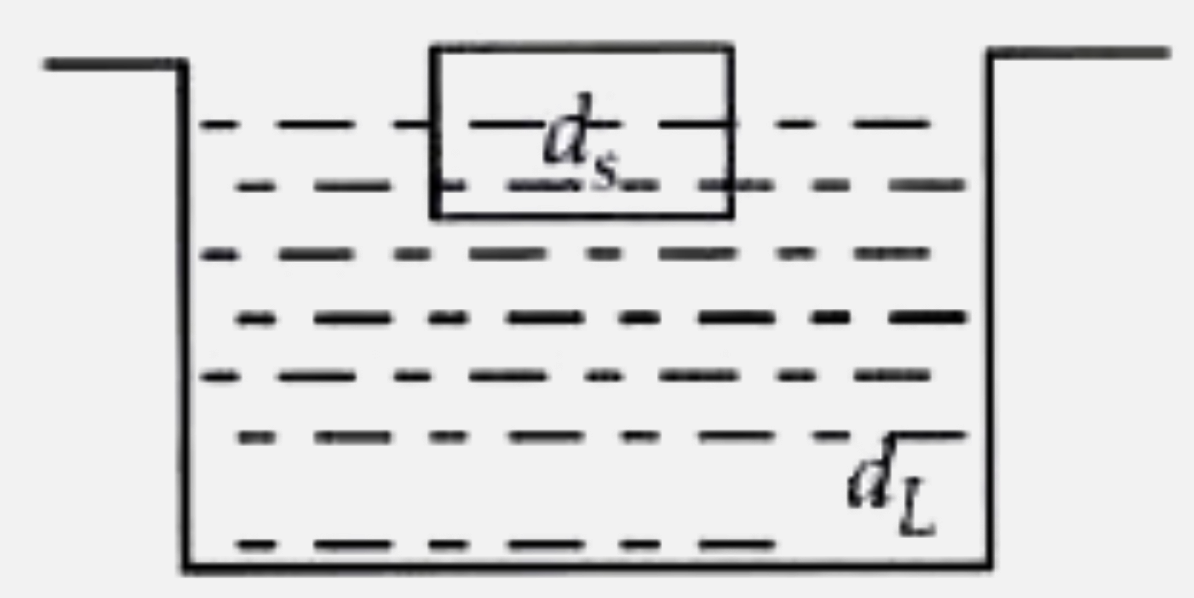
The figure above shows a solid of density dS floating in a liquid of density dL.
The same solid floats in water with 3th5 of its volume immersed in it. Calculate the density of the solid. (density of water = 1 g cm−3 )
View SolutionThe weight of a body in water is one third of its weight in air. The density of the body is
View Solution
